The Bergman Kernel on Tube Domains

Ching-I Hsin,

Minghsin University of Science and Technology, Taiwan.

Abstract
Let 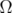be a bounded strictly convex domain in 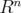, and 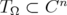the tube domain over 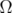. In this paper, we show that the Bergman kernel of 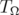can be expressed easily by an integral formula.

2000 Mathematics Subject Classification : 32A07, 32A25.

Key words and phrases. Bergman kernel; Tube domain.

1. Introduction

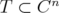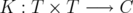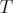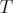Let be a domain. The Bergman kernel (see for instance [4]) is one of the important holomorphic invariants associated to , but it is often difficult to compute . We shall show that if is a tube domain over a bounded strictly convex domain, then an easy computation leads quickly to its Bergman kernel.

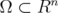Let be a bounded strictly convex domain. We are interested in domains of the type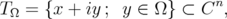

(1.1)

known as the tube domain over 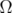. Let 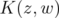be the Bergman kernel of 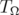. The main result of this paper is as follows.

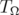Theorem The Bergman kernel on the tube domain is given by

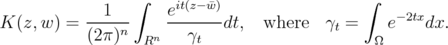(1.2)

Given a function on 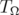, we shall say that 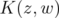reproduces if

(1.3)

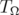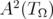where is the Lebesgue measure on . Let denote the Bergman space, namely the Hilbert space of all holomorphic functions 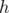on 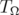in which

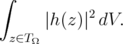(1.4)

The Bergman kernel 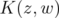is uniquely characterized by the following three properties:

(i) for all ;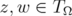

(ii) 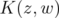reproduces every element in in the sense of (1.3);

(iii) 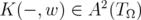for all .

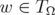Our formulation of 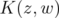in (1.2) clearly satisfies condition (i). Therefore, to prove the theorem, we need to check conditions (ii) and (iii). This will be done by Propositions 2.4 and 2.5 in the next section.

2. The Bergman kernel on tube domains

Let 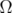be a bounded strictly convex domain in 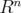, and let 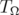be the tube domain as defined in (1.1). Since 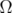is strictly convex, 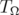is strictly pseudoconvex.

Let 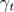and 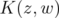be defined as in (1.2). The Bergman space 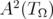consists of holomorphic functions which satisfy (1.4). In this section, we show that satisfies conditions (ii) and (iii) stated in the Introduction. Let denote the polynomial functions, and we define

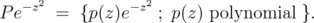
Lemma 2.1 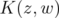reproduces every element of .

Proof: The following identity shall be useful:

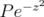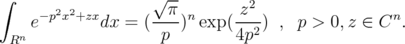(2.1)

Here denote the usual dot product. In deriving this identity, we observe that both sides of (2.1) are holomorphic in 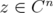. Therefore, it suffices to check that it holds on the totally real subspace . This can be obtained from standard integration tables ([3], 3.323 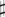2).

Write 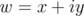on 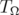, where 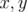are variables on 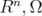respectively. Then

Consequently,

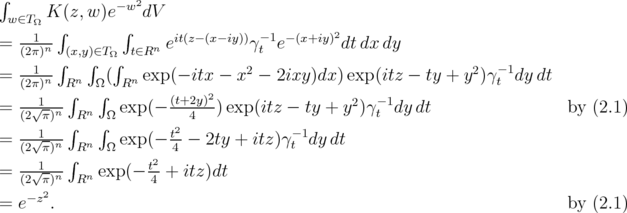
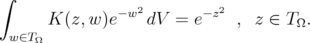(2.2)

From (2.2), substitute with 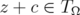, where . This gives

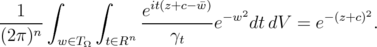
Changing the variable 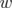to in LHS gives

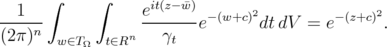
Apply 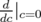to both sides, we see that 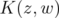reproduces the function 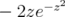. We carry out the procedure 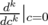for 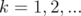, and Lemma 2.1 follows.

We plan to show that 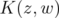reproduces every element of 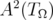. In view of Lemma 2.1, this will follow if we can show that 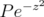is a dense subset of the Hilbert space 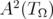. This will be established by the next two lemmas. Our strategy is to convert the problem on 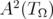to another Hilbert space 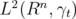by Lemma 2.2, and obtain a result on 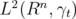by Lemma 2.3. We then transfer this result back to 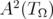, by Proposition 2.4.

Let 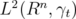denote the Hilbert space of 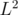functions on 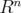, with weight 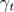given in (1.2). Namely,

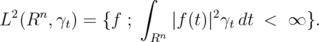
Lemma 2.2 (T. G. Genchev) The transformation

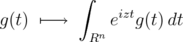(2.3)

is an isometry from 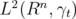to 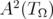, preserving the Hilbert space norms.

Proof: Given , there exists 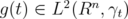such that

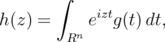(2.4)

see [1],[2]. This means that the transformation in (2.3) is surjective. In order to see that (2.3) is well-defined, injective and preserves the norms, we shall prove that in (2.4), .

Write 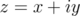, and (2.4) gives

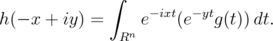
Thus for every fixed 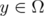, is the Fourier transform of 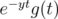. By Plancherel's theorem,

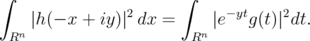
Apply 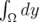to both sides, we get

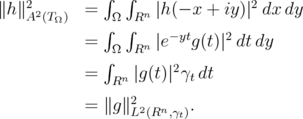
This proves Lemma 2.2. □

Next we define

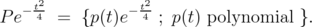
Lemma 2.3 is dense in the Hilbert space .

Proof: We shall show that

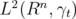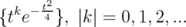(2.5)

is a complete basis of 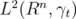, and the lemma follows.

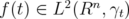Let , and suppose that

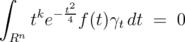(2.6)

for all 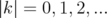. It is easy to see that 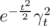is bounded, so

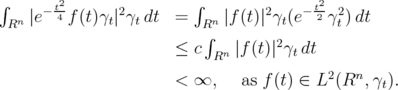
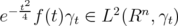Therefore, . Let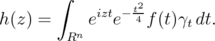

(2.7)

By Lemma 2.2, 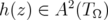. We may assume that 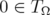, and consider the power series expansion

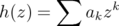
near . Then

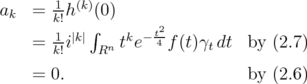
So 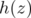vanishes near . However, since is holomorphic, it follows that . By Lemma 2.2,

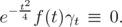
Since 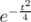and 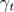are always positive,

This shows that the functions in (2.5) form a complete basis of 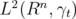, and the lemma is proved. □

We combine Lemmas 2.1, 2.2 and 2.3 to show that:

Proposition 2.4 The function 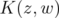of (1.2) reproduces every element of .

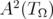Proof: We claim that 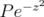is dense in 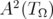. Identity (2.1) implies that

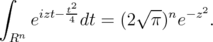
Applying 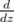repeatedly to both sides, we see that if 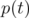is a polynomial, then

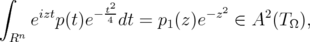
for another polynomial 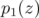. Namely, the isometry in Lemma 2.2 sends every

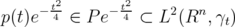
to some

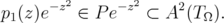
By Lemma 2.3, 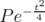is dense in 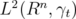. Hence 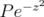is dense in 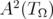as claimed.

By Lemma 2.1, 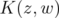reproduces every element of 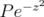. Therefore, since is dense in 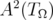, it follows that 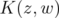reproduces every element of . □

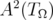With this result, condition (ii) of stated in the Introduction is verified. Therefore, to prove the theorem, it remains only to check condition (iii). This is done by the following proposition.

Proposition 2.5 For each fixed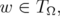

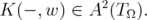
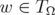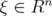Proof: Fix , and consider . For any fixed , formula (1.2) satisfies

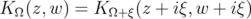
for all 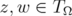. Further, 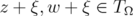and 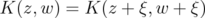. Therefore, without loss of generality, we may assume that 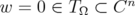in the statement of this proposition. We want to show tha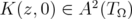t . But

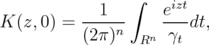
and with Lemma 2.2, we get

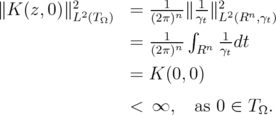
We have shown that, for each , .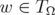

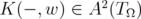This result verifies condition (iii) of the Introduction, and the theorem follows.

References

[1]   T. G. Genchev, Integral representations for functions holomorphic in tube domains, C. R. Acad. Bulgare Sci. 37 (1984), 717-720.         [ Links ]

[2]   T. G. Genchev, Paley-Wiener type theorems for functions in Bergman spaces over tube domains, J. Math. Anal. and Appl. 118 (1986), 496-501.         [ Links ]

[3]   I. S. Gradshteyn, I. M. Ryzhik, Table of Integrals, Series and Products, Academic Press, (1980).         [ Links ]

[4]   S. Krantz, Function Theory of Several Complex Variables, 2ed. Wadsworth & Brooks/Cole, Pacific Grove 1992.         [ Links ]

Ching-I Hsin
Division of Natural Science,
Minghsin University of Science and Technology.
Hsinchu County 304, Taiwan.
hsin@must.edu.tw

Recibido: 6 de mayo de 2004
Aceptado: 28 de marzo de 2005