Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO
Links relacionados
-
 Similares en
SciELO
Similares en
SciELO
Compartir
Revista de la Unión Matemática Argentina
versión impresa ISSN 0041-6932versión On-line ISSN 1669-9637
Rev. Unión Mat. Argent. v.47 n.1 Bahía Blanca ene./jun. 2006
4-step Carnot spaces and the 2-stein condition
María J. Druetta
Dedicated to the memory of my goddaughter María Pía
Abstract: We consider the 2-stein condition on k-step Carnot spaces S. These spaces are a subclass in the class of solvable Lie groups of Iwasawa type of algebraic rank one and contain the homogeneous Einstein spaces within this class. They are obtained as a semidirect product of a graded nilpotent Lie group N and the abelian group R.
We show that the 2-stein condition is not satisfied on a proper 4-step Carnot spaces S.
2000 Mathematics Subject Classification. 53C30, 53C55
Key words and phrases: 2-stein spaces, k-step Carnot spaces, Einstein spaces, Lie groups of Iwasawa type, Damek-Ricci spaces
A Riemannian manifold 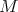 is said to be a
is said to be a 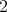 -stein space, if there exist functions
-stein space, if there exist functions 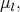
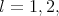 defined on
defined on 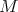 such that
such that
 |
Here,  denote the Jacobi operator associated to
denote the Jacobi operator associated to  defined by
defined by 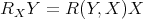 for all
for all 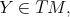 where
where 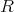 is the curvature tensor of
is the curvature tensor of  Harmonic riemannian manifolds are necessarily
Harmonic riemannian manifolds are necessarily 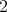 -stein.
-stein.
A 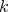 -step Carnot space (
-step Carnot space ( ) is a simply connected solvable Lie group
) is a simply connected solvable Lie group 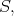 which is a semidirect product of a nilpotent Lie group
which is a semidirect product of a nilpotent Lie group 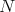 and the abelian group
and the abelian group 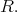 Assume that
Assume that 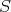 and
and 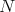 have associated Lie algebras
have associated Lie algebras 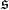 and
and 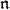 , respectively.
, respectively. 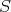 has the left invariant metric induced by the one given on
has the left invariant metric induced by the one given on 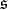 , where
, where 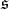 is a solvable metric Lie algebra
is a solvable metric Lie algebra 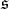 with inner product
with inner product 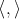 such that:
such that:
(i) 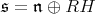 with
with ![𝔫 = [𝔰,𝔰]](/img/revistas/ruma/v47n1/1a0240x.png) and
and 
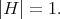
(ii) 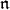 has an orthogonal decomposition
has an orthogonal decomposition 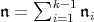 into
into  subspaces given by
subspaces given by
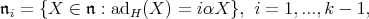 |
for some positive constant 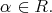
Note that, since the adjoint representation ad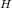 is a derivation of
is a derivation of 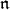 the above decomposition defines a graded Lie algebra structure of
the above decomposition defines a graded Lie algebra structure of 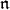 , that is,
, that is,
![[𝔫i,𝔫j] ⊂ 𝔫i+j 1 ≤ i,j ≤ k - 1](/img/revistas/ruma/v47n1/1a0251x.png) |
with the convention  for
for 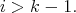 In particular,
In particular, 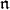 is a (
is a ( -step nilpotent Lie algebra, that is, the (
-step nilpotent Lie algebra, that is, the ( -th derived algebra vanishes:
-th derived algebra vanishes: ![𝔫k-1 = [𝔫,...[𝔫, [𝔫,𝔫 ]]...] = {0 }.](/img/revistas/ruma/v47n1/1a0257x.png)
Note, that up to a constant in the metric, we may suppose that  In this terminology, the
In this terminology, the 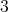 -Carnot spaces are the well known Carnot spaces and the case of a
-Carnot spaces are the well known Carnot spaces and the case of a 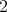 -step Carnot space corresponds to the hyperbolic symmetric space
-step Carnot space corresponds to the hyperbolic symmetric space  Moreover, due to a result of [6], any homogeneous Einstein space of Iwsawa type and rank one is a
Moreover, due to a result of [6], any homogeneous Einstein space of Iwsawa type and rank one is a 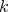 - step Carnot space for some
- step Carnot space for some 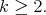
We remark that the 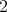 -stein condition was studied in [1] and [3] on Lie groups
-stein condition was studied in [1] and [3] on Lie groups 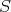 of Iwasawa type in the case of
of Iwasawa type in the case of 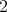 -step nilpotent
-step nilpotent  , and in particular, on Carnot spaces: in this class those which are
, and in particular, on Carnot spaces: in this class those which are 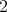 -stein are exactly the Damek-Ricci spaces (also the harmonic ones). Furthermore, in [7] is shown that a simply connected homogenous harmonic space is a Carnot space, which in turn is equivalent to
-stein are exactly the Damek-Ricci spaces (also the harmonic ones). Furthermore, in [7] is shown that a simply connected homogenous harmonic space is a Carnot space, which in turn is equivalent to 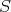 be a Damek-Ricci space.
be a Damek-Ricci space.
Assume that 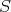 is a
is a 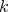 -step Carnot space. Let
-step Carnot space. Let 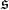 be the Lie algebra of
be the Lie algebra of  : that is
: that is 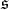 is a solvable metric Lie algebra
is a solvable metric Lie algebra 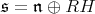 with
with 
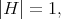 with
with 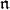
![= [𝔰,𝔰]](/img/revistas/ruma/v47n1/1a0280x.png) a graded nilpotent Lie algebra as described above.
a graded nilpotent Lie algebra as described above.
Let  denote the center of
denote the center of 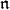 and let
and let 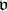 be the orthogonal complement of
be the orthogonal complement of  with respect to the metric
with respect to the metric 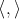 restricted to
restricted to 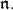 Thus
Thus 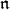 decomposes
decomposes 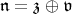 and note that ad
and note that ad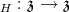 hence ad
hence ad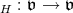 since ad
since ad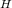 is symmetric.
is symmetric.
For any 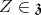 the skew-symmetric linear operator
the skew-symmetric linear operator  is defined by
is defined by
![⟨jZX, Y ⟩ = ⟨[X, Y ],Z⟩ for all X, Y ∈ 𝔳, all Z ∈ 𝔷.](/img/revistas/ruma/v47n1/1a0294x.png) |
The Levi Civita connection and the curvature tensor on 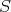 can be computed by,
can be computed by,
![2 ⟨∇X Y, Z⟩ = ⟨[X, Y ],Z⟩ - ⟨[Y,Z ],X ⟩ + ⟨[Z,X ],Y ⟩ R (X, Y) = [∇X ,∇Y ] - ∇ [X,Y]](/img/revistas/ruma/v47n1/1a0296x.png)
for any 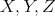 in
in 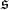 .
.
Recall that since  and
and 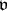 are ad
are ad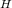 -invariant, they also have decompositions into eigenspaces as
-invariant, they also have decompositions into eigenspaces as 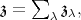
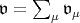 with
with 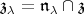 ,
, 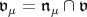 and the equality
and the equality  may be possible
may be possible Moreover,
Moreover, 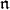 is said to be
is said to be 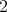 -step nilpotent if
-step nilpotent if ![[𝔫,𝔫] = [𝔳,𝔳 ] ⊂ 𝔷.](/img/revistas/ruma/v47n1/1a02110x.png) Here,
Here, 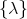 and
and 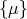 denote the eigenvalues of
denote the eigenvalues of 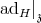 and
and  , respectively, that we assume they are natural numbers.
, respectively, that we assume they are natural numbers.
1.1. Properties of the operator 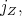
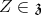 . We recall that for any
. We recall that for any 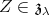 so that
so that 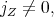
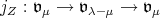 and
and  isomorphically. Consequently, as follows from [5, Lemma1.1])
isomorphically. Consequently, as follows from [5, Lemma1.1])
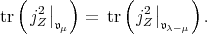 |
1.2. The curvature formulas. By applying the connection formula, one obtains  and if
and if 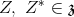 and
and 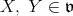 , then
, then ![* * 1 ∇Z Z = ∇Z *Z = ⟨[H, Z],Z ⟩H, ∇X Z = ∇Z X = - 2jZ X](/img/revistas/ruma/v47n1/1a02125x.png) and
and ![∇X Y = 12[X, Y ] + ⟨[H, X ],Y⟩H](/img/revistas/ruma/v47n1/1a02126x.png) in case of
in case of 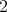 -step nilpotent
-step nilpotent 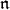 . Consequently, by a direct computation we obtain the following formulas involving curvatures (see [2, Section 2]).
. Consequently, by a direct computation we obtain the following formulas involving curvatures (see [2, Section 2]).
(i) 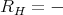 ad
ad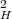
(ii) If either 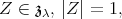 or
or 
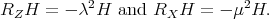 |
(iii) If 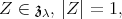 for any
for any 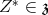 and
and 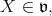 then
then
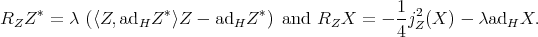 |
(iv) Let 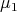 denote the maximum eigenvalue of
denote the maximum eigenvalue of 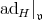 . If
. If 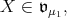
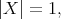 then
then
![1 1 * RX Z = --[X, jZ X ] - μ1adH Z - -(adjZX ) X, Z ∈ 𝔷 4 4 RX Y = - 1-[X, ∇ 𝔫 Y] - 3j[X,Y]X - ∇ 𝔫 (∇𝔫 Y )𝔳 - μ1adH Y, Y ⊥ X in 𝔳. 2 X 4 X X](/img/revistas/ruma/v47n1/1a02142x.png)
1.3. The Einstein condition. Assume that 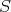 is an Einstein space, that is Ric
is an Einstein space, that is Ric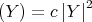 for all
for all 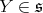 . Let
. Let 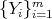 and
and 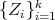 be orthonormal bases of
be orthonormal bases of  and
and  , respectively, with
, respectively, with 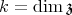 and
and 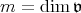 . We use the well known formulas that hold in a solvable metric Lie algebra
. We use the well known formulas that hold in a solvable metric Lie algebra 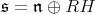 ,
,
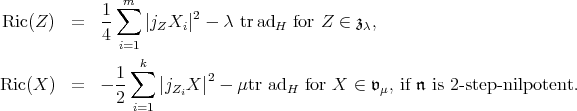
Note that the first Einstein condition becomes
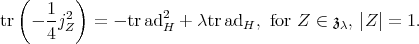 | (1) |
In general, for a unit vector 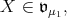
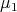 the maximun eigenvalue of
the maximun eigenvalue of 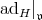 , we have
, we have
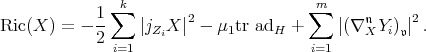 | (2) |
The last one is a direct computation by applying the above expressions of 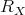 in (iv) to
in (iv) to
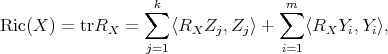 |
having into account that the following equality holds,
![m k ∑ |[X, Y ]|2 = ∑ |j X |2. i Zi i=1 j=1](/img/revistas/ruma/v47n1/1a02161x.png) |
In fact, this follows by computing
![∑m 2 ∑m ∑k 2 ∑m ∑k ⟨ ⟩2 |[X, Yi]| = ⟨[X, Yi],Zj⟩ = jZjX, Yi i=1 i=1 j=1 i=1 j=1 k ( m ) k | | ∑ ∑ ⟨ ⟩2 ∑ | |2 = jZjX, Yi = |jZjX | , j=1 i=1 j=1](/img/revistas/ruma/v47n1/1a02162x.png)
since ![[X, Y ] ∈ 𝔷 i](/img/revistas/ruma/v47n1/1a02163x.png) for any
for any 
1.4. The 2-stein condition. For any 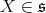 , the Jacobi operator
, the Jacobi operator 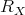 associated to
associated to 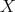 is the symmetric endomorphism of
is the symmetric endomorphism of 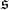 defined by
defined by 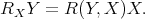 We said that
We said that  is a
is a 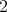 -stein space, or equivalently
-stein space, or equivalently 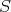 (or
(or 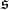 ) satisfies the
) satisfies the 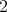 -stein condition, if there exist
-stein condition, if there exist
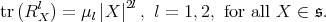 |
In particular, 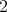 -stein spaces are Einstein: Ric
-stein spaces are Einstein: Ric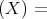 tr
tr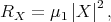
 a constant, for all
a constant, for all 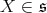 , and harmonic riemannian spaces are necessarily
, and harmonic riemannian spaces are necessarily 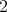 -stein. The
-stein. The 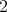 -stein condition was studied on Carnot spaces in [1] and [3]: the
-stein condition was studied on Carnot spaces in [1] and [3]: the 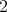 -stein Carnot spaces are exactly the Damek-Ricci spaces (also the harmonic ones) in this class.
-stein Carnot spaces are exactly the Damek-Ricci spaces (also the harmonic ones) in this class.
The proposition below express the 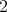 -stein condition in an adequate form for our purposes. Let
-stein condition in an adequate form for our purposes. Let 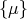 denote the set of eigenvalues of
denote the set of eigenvalues of 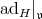 .
.
Proposition 1.1. Assume that 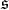 satisfies the
satisfies the 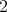 -stein condition. If
-stein condition. If 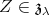 is a unit vector, then
is a unit vector, then
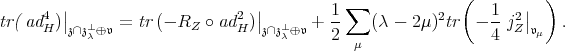 |
In particular, if 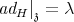 Id
Id
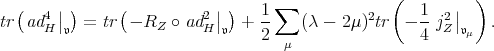 | (3) |
Proof. It follows from the same argument as those used in Theorem 4.1 of [4] for  (See also [5, Proposition 1.2]). □
(See also [5, Proposition 1.2]). □
2. The 2-stein condition on 4-step Carnot spaces
We consider the case of a 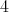 -step Carnot space; that is,
-step Carnot space; that is, 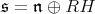 as above with
as above with  . Hence, by the propeties of
. Hence, by the propeties of 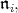
![[𝔫1,𝔫1] ⊂ 𝔫2,](/img/revistas/ruma/v47n1/1a02200x.png)
![[𝔫1,𝔫2] ⊂ 𝔫3,](/img/revistas/ruma/v47n1/1a02201x.png)
![[𝔫1,𝔫3] = 0,](/img/revistas/ruma/v47n1/1a02202x.png)
![[𝔫2,𝔫2] = 0 = [𝔫2, 𝔫3],](/img/revistas/ruma/v47n1/1a02203x.png)
![[𝔫3,𝔫3] = 0](/img/revistas/ruma/v47n1/1a02204x.png) and
and 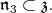 We observe that:
We observe that:
![[𝔫,𝔫] ⊂ 𝔫 ⊕ 𝔫 2 3](/img/revistas/ruma/v47n1/1a02206x.png) and
and ![[[𝔫,𝔫],𝔫] ⊂ [𝔫 ⊕ 𝔫 ,𝔫 ⊕ 𝔫 ⊕ 𝔫 ] ⊂ 𝔫 . 2 3 1 2 3 3](/img/revistas/ruma/v47n1/1a02207x.png) Thus,
Thus, 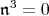 since
since ![[[[𝔫,𝔫 ],𝔫],𝔫] ⊂ [𝔫3,𝔫] = 0;](/img/revistas/ruma/v47n1/1a02209x.png) that is
that is 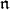 is
is 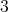 -step nilpotent and
-step nilpotent and 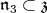 . Since
. Since 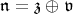 we have that
we have that 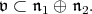 Moreover, from the facts that
Moreover, from the facts that  and
and 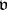 are ad
are ad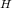 -invariant, it follows that
-invariant, it follows that
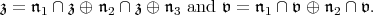 |
We set 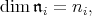
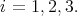 Following with the notation introduced in the previous section we have,
Following with the notation introduced in the previous section we have,
Lemma 2.1. If 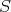 is a
is a 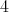 -step Carnot space that satisfies the
-step Carnot space that satisfies the 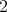 -stein condition, then
-stein condition, then  is either a Damek-Ricci space or, up to scaling, the symmetric hyperbolic space
is either a Damek-Ricci space or, up to scaling, the symmetric hyperbolic space 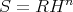
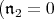 or
or 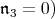 with
with 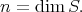 If
If 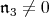 the equalities
the equalities 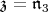 ,
, 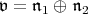 hold in the decomposition of
hold in the decomposition of 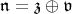 .
.
Proof. ( )
) 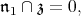 if the Einstein condition is satisfied.
if the Einstein condition is satisfied.
If 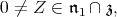
![⟨jZX, Y ⟩ = ⟨[X, Y ],Z⟩ = 0](/img/revistas/ruma/v47n1/1a02236x.png) for all
for all  since
since ![[X, Y ] ∈ ∑ 𝔫i i≥2](/img/revistas/ruma/v47n1/1a02238x.png) and
and 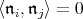 if
if 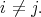 Hence,
Hence, 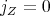 and tr
and tr and the Einstein condition (1) implies that
and the Einstein condition (1) implies that
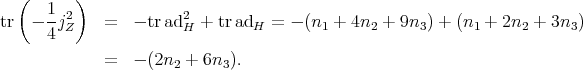
Thus, 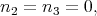 and consequently
and consequently 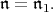 In this case
In this case 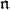 is abelian and
is abelian and 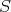 corresponds to the symmetric hyperbolic space
corresponds to the symmetric hyperbolic space 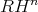 with
with 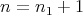 .
.
The previous fact implies that the eigenvalue 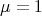 is not achieved in
is not achieved in  , when
, when 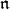 is
is 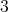 -step properly. Thus,
-step properly. Thus,
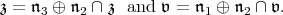 |
(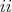 )
)  if the
if the 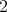 -stein condition holds.
-stein condition holds.
In fact, assume that there exists 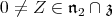 . In this case
. In this case if
if 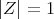 condition (1) gives
condition (1) gives
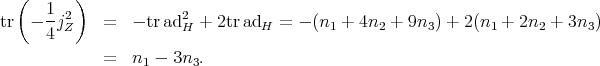
Moreover, since 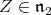 and
and ![⟨jZ X, Y⟩ = ⟨[X, Y ],Z ⟩ = 0](/img/revistas/ruma/v47n1/1a02263x.png) for all
for all 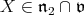 ,
,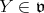 , we have that
, we have that 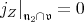 and
and 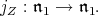 Now, we apply the formula given in Proposition 1.1 for
Now, we apply the formula given in Proposition 1.1 for 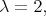 and
and  We first note that,
We first note that,
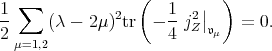 |
since 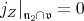 and
and  for
for 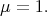 This implies that tr(
This implies that tr(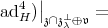 tr
tr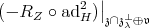 and consequently, equality holds in the Cauchy-Schwartz Inequality
and consequently, equality holds in the Cauchy-Schwartz Inequality
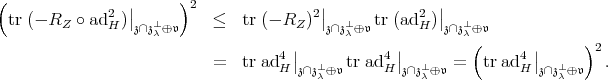
Therefore, 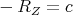 ad
ad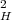 in
in  with
with 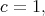 by the Einstein condition. The equality
by the Einstein condition. The equality 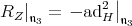 , implies that
, implies that 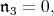 since
since 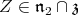 and
and 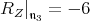 Id
Id Hence,
Hence, 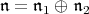 with
with 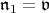 ,
,  and
and 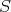 is a Carnot space. In this case
is a Carnot space. In this case 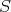 is a Damek-Ricci space (see [3, Theorem 3.1]).□
is a Damek-Ricci space (see [3, Theorem 3.1]).□
Assume that 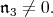 If
If 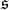 satisfies the
satisfies the 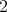 -stein condition then
-stein condition then 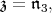 and
and 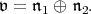
The following proposition is basic for our purposes,
Proposition 2.2. If 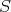 is a
is a 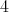 -step Carnot space that is
-step Carnot space that is 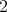 -stein, then
-stein, then 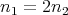 and
and 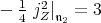 Id for any unit vector
Id for any unit vector 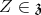 . Equivalently,
. Equivalently,  for all unit vectors
for all unit vectors 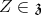 and
and 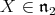 .
.
Proof. Next we will show that 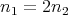 ,
,
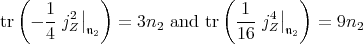 |
for any unit vector 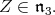
Let 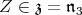 be a unit vector. The Einstein condition (1) applied to
be a unit vector. The Einstein condition (1) applied to  gives,
gives,
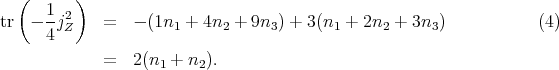
 it follows that
it follows that 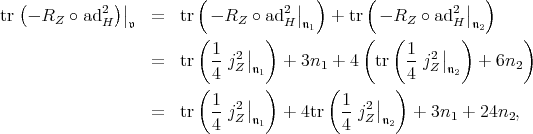
and applying (4),
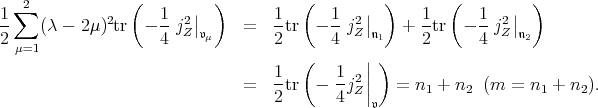
Thus, (3) gives
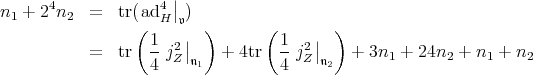
and consequently,
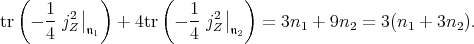 | (5) |
Now, using that 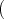 tr
tr tr
tr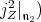 (see 1.1), (4) and (5), that is
(see 1.1), (4) and (5), that is
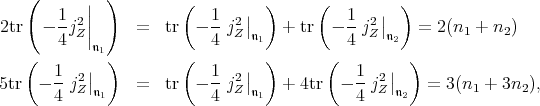
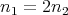 is obtained, since
is obtained, since
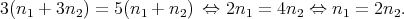 |
Finally, we will show the last assertion of the proposition. Using that 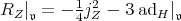 again
again we compute
we compute
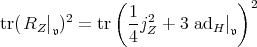 |
by developing  Thus,
Thus,

and substituting the values of 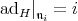 Id, we have
Id, we have
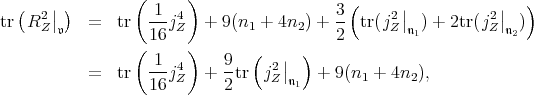
since tr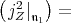 tr
tr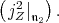 Note, that the same argument used to show this equality, implies
Note, that the same argument used to show this equality, implies
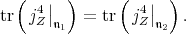 | (6) |
Therefore, the 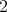 -stein condition, tr
-stein condition, tr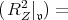 tr
tr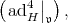 gives
gives
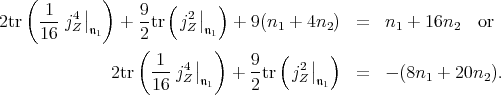
By applying (4), the last equality is equivalent to

It follows from (6) that
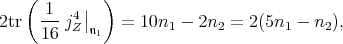 |
and from the fact 
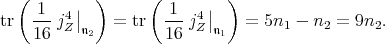 |
The final assertion follows as claimed, since
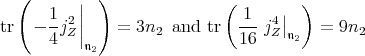 |
implies that equality holds in the Cauchy-Schwartz Inequality
 |
which gives 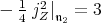 Id
Id or equivalently,
or equivalently, 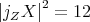 for all unit vector
for all unit vector 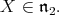 □
□
Let 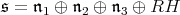 be a
be a 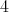 -step Lie algebra so that
-step Lie algebra so that 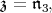 and
and 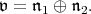 In what follows let
In what follows let 
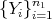 and
and 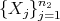 be any orthonormal bases of
be any orthonormal bases of  ,
, 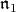 and
and  , respectively. The following basic formulas are deduced from the hypothesis
, respectively. The following basic formulas are deduced from the hypothesis 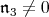 and not assuming that the
and not assuming that the  -stein condition is satisfied. They are shown in [5, Proposition 2.3].
-stein condition is satisfied. They are shown in [5, Proposition 2.3].
If 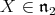 and
and 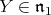 are unit vectors, then
are unit vectors, then
![n2 n3 ∑ 2 ∑ 2 |[Y, Xj]| = |jZkY | j=1 k=1](/img/revistas/ruma/v47n1/1a02360x.png) | (7) |
![∑n2 |( ) | n∑1 || ∇ 𝔫 Y ||2 = 1- |[Y,Y ]|2 Xj 𝔳 4 i j=1 i=1](/img/revistas/ruma/v47n1/1a02361x.png) | (8) |
In order to show that the 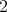 -stein condition is not satisfied by
-stein condition is not satisfied by 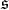 , in case that
, in case that 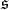 is
is 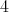 -step properly, we need to compute Ric
-step properly, we need to compute Ric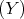 and Ric
and Ric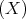 for unit vectors
for unit vectors 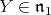 and
and 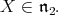
Lemma 2.3. If 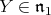 is a unit vector then,
is a unit vector then,
![n2 n1 1∑ 2 1∑ 2 tr RY = - n1 - 2n2 - 3n3 - 2 |[Y, Xj]| - 2 |[Y, Yi]| . j=1 i=1](/img/revistas/ruma/v47n1/1a02371x.png) |
Proof. Let 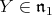 be a unit vector. We first note, tha it is a direct computation to see that
be a unit vector. We first note, tha it is a direct computation to see that
![1 ∇Y W = -[Y,W ] for W ∈ 𝔫1, W ⊥ Y 2 ∇ X = 1[Y,X ] + (∇ 𝔫X ) for X ∈ 𝔫 . Y 2 Y 𝔳 2](/img/revistas/ruma/v47n1/1a02373x.png)
Therefore, for unit vectors 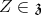 ,
, 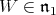 and
and 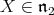 using the definition of
using the definition of 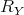 we obtain
we obtain
![1 RY Z = - 3Z + -∇Y jZY, 2 1- RY W = - W - 2∇Y [W, Y ] - ∇ [W,Y]Y , 1 RY X = - 2X - ∇Y ∇X Y + -j[X,Y ]Y , RY H = - H. 2](/img/revistas/ruma/v47n1/1a02378x.png)
Now, it is a strighforward computation using the definition of 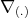 to see that,
to see that,
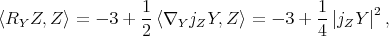 |
![1- ⟨ ⟩ ⟨RY W, W ⟩ = - 1 - 2 ⟨∇Y [W, Y ],W ⟩ - ∇ [W,Y ]Y,W 1 1 = - 1 - -|[Y,W ]|2 + -⟨[Y,W ],[W, Y ]⟩ 4 2 = - 1 - 3|[Y,W ]|2 4](/img/revistas/ruma/v47n1/1a02381x.png)
and
![⟨ ⟩ ⟨RY X, X ⟩ = - 2 - ⟨∇Y ∇X Y,X ⟩ + 1- j[X,Y ]Y, X 2 1- = - 2 + ⟨∇X Y,∇Y X ⟩ + 2 ⟨[Y, X ],[X, Y ]⟩ 1 = - 2 + |∇X Y |2 + ⟨∇X Y,[Y,X ]⟩ ---|[X, Y ]|2 2 2 2 = - 2 + |∇X Y | - |[X, Y]| 3 2 2 = - 2 - -|[X,Y ]| + |(∇ 𝔫XY )𝔳| . 4](/img/revistas/ruma/v47n1/1a02382x.png)
Next, by computing
![n n ∑3 1-∑ 3 2 tr RY |𝔷⊕RH = ⟨RY Zk, Zk⟩ - 1 = - 3n3 + 4 |jZkY | - 1 k=1 k=1 n∑1 3 n∑1 tr RY |𝔫1 = ⟨RY Yi,Yi⟩ = - (n1 - 1) - -- |[Y,Yi]|2 i=1 4 i=1 n∑2 n∑2 ∑n2 tr R | = ⟨R X ,X ⟩ = - 2n + ||(∇ Y ) ||2 - 3- |[X ,Y ]|2, Y 𝔫2 Y j j 2 Xj 𝔳 4 j j=1 j=1 j=1](/img/revistas/ruma/v47n1/1a02383x.png)
it is immediate that
![n3 n1 1∑ 2 3-∑ 2 tr RY = - n1 - 2n2 - 3n3 + 4 |jZk Y| - 4 |[Y,Yi]| k=1 i=1 n∑2 |( ) |2 3∑n2 2 + | ∇Xj Y 𝔳| - -- |[Y, Xj ]| . j=1 4 j=1](/img/revistas/ruma/v47n1/1a02384x.png)
By applying the equalities given by (7) and (8) we have,
![n2 n1 1-∑ 2 1-∑ 2 trRY = - n1 - 2n2 - 3n3 - 2 |[Y,Xj ]| - 2 |[Y,Yi]| , j=1 i=1](/img/revistas/ruma/v47n1/1a02385x.png) |
the expression stated in the lemma. □
Corollary 2.4. If 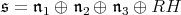 satisfies the Einstein condition, then for all unit vectors
satisfies the Einstein condition, then for all unit vectors 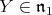 and
and 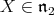 we have,
we have,
![∑n1 1 ∑k (i) |(∇ 𝔫XYi)𝔳|2 - -- |jZiX |2 = n1 - 3n3 i=1 2 i=1 ∑n2 ∑n1 (ii) |[Y, X ]|2 + |[Y, Y ]|2 = 4 (n + 3n ). j i 2 3 j=1 i=1](/img/revistas/ruma/v47n1/1a02389x.png)
Proof. (i) and (ii) are obtained by applying the Einstein condition tr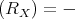 tr
tr ad
ad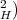 (2) to
(2) to  and tr
and tr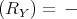 tr
tr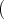 ad
ad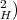 to
to 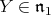 (Lemma 2.3), respectively. In fact, they are immediate since
(Lemma 2.3), respectively. In fact, they are immediate since

and
![n n 1-∑ 2 2 1-∑ 1 2 2 - n1 - 2n2 - 3n3 - 2 |[Y, Xj]| - 2 |[Y, Yi]| = - tr(adH ) j=1 i=1 - n1 - 4n2 - 9n3,](/img/revistas/ruma/v47n1/1a02399x.png)
implying the required formulas. □
Theorem 2.5. If 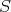 is a
is a 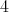 -step Carnot space that satisfies the
-step Carnot space that satisfies the 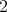 -stein condition, then
-stein condition, then 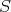 is either, up to scaling, the hyperbolic space
is either, up to scaling, the hyperbolic space 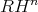
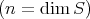 or a Damek-Ricci space.
or a Damek-Ricci space.
Proof. Let  be a
be a 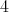 -step Carnot space with associated Lie algebra
-step Carnot space with associated Lie algebra 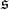 . If
. If 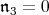 then either
then either 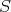 is, up to scaling, the hyperbolic symmetric space
is, up to scaling, the hyperbolic symmetric space 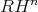 with
with 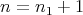 or
or 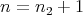 according to
according to  or
or 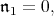 or
or 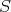 is a Damek-Ricci space by [3, Theorem 3.1].
is a Damek-Ricci space by [3, Theorem 3.1].
Assume that 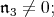 we will show that
we will show that 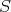 cannot be
cannot be 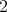 -stein unless
-stein unless 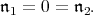
(i) Let 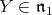 a unit vector. If
a unit vector. If 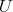 is the operator on
is the operator on 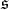 defined by
defined by 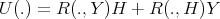 it follows that
it follows that
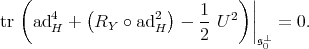 | (9) |
where 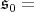 span
span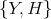 is the totally geodesic subalgebra of
is the totally geodesic subalgebra of 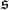 which corresponds to the symmetric hyperbolic space
which corresponds to the symmetric hyperbolic space 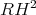 of constant curvature
of constant curvature  We show this expression (9) following the same argument developed in [5, Proposition 1.2]. In order to do that we need to revise some properties fulfilled by
We show this expression (9) following the same argument developed in [5, Proposition 1.2]. In order to do that we need to revise some properties fulfilled by 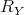 and
and 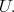 First, note that
First, note that
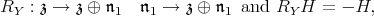 |
hence 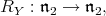 since it is symmetric.
since it is symmetric.
Next, by using the definition of 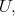 for any unit vectors
for any unit vectors 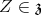 ,
, 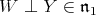 and
and 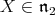 we compute:
we compute:
![U (Z ) = ∇Z ∇Y H - ∇Y ∇Z H - ∇ [Z,H ]Y = - ∇Z Y + 3 ∇Y Z + 3∇Z Y 5 = 5∇Z Y = - 2jZY,](/img/revistas/ruma/v47n1/1a02439x.png)
![U (W ) = ∇W ∇Y H - ∇Y ∇W H - ∇ [W,Y]H - ∇ [W,H ]Y = - ∇W Y + ∇Y W + 2[W, Y ] + ∇W Y 1- 3- = 2[Y,W ] - 2[Y,W ] = - 2 [Y, W ] and](/img/revistas/ruma/v47n1/1a02440x.png)
![U (X ) = ∇X ∇Y H - ∇Y ∇X H - ∇ [X,Y]H - ∇ [X,H ]Y = - ∇X Y + 2∇Y X + 3[X, Y ] + 2∇X Y = ∇X Y + 2∇Y X + 3 [X, Y ] = 3∇X Y + [X, Y ] = 3-[X, Y ] + 3(∇ 𝔫X Y )𝔳 + [X, Y ] = 5-[X, Y ] + 3(∇𝔫X Y )𝔳. 2 2](/img/revistas/ruma/v47n1/1a02441x.png)
Thus,  and
and  This property, togheter with the fact
This property, togheter with the fact 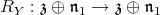 and
and 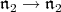
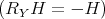 imply that (9) holds, since
imply that (9) holds, since
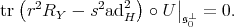 |
(ii) Next, (9) it will be applied, computing separately each term and using (7) and (8). First, from the above computations we obtain
![⟨U 2Z,Z ⟩ = |U (Z )|2 = 25-|jZY |2 for all Z ∈ 𝔷, |Z | = 1 4 ⟨ 2 ⟩ 2 9- 2 U W, W = |U (W )| = 4 |[Y,W ]| , |W | = 1, and ⟨ ⟩ 25 U 2X, X = |U (X )|2 = --|[X, Y]|2 + 9 |(∇ 𝔫X Y )𝔳|2, for X ∈ 𝔫2, |X | = 1. 4](/img/revistas/ruma/v47n1/1a02448x.png)
Hence,
![( 2|| ) n∑3 2 ∑n1 2 ∑n2 2 tr U 𝔰0⊥ = |U (Zk)|+ |U(Yi)|+ |U(Xj )| k=1n i=1 n j=1 n n 25∑ 3 2 9 ∑1 2 25 ∑2 2 ∑2 || 𝔫 ||2 = 4 |jZkY| + 4 |[Y,Yi]| + 4 |[Y,Xj]|+ 9 |(∇ XjY)𝔳| k=n11 i=1 n2 j=1 j=1 = 29∑ |[Y, Y]|2 + 225∑ |[Y,X ]|2, 4 i=1 i 4 j=1 j](/img/revistas/ruma/v47n1/1a02449x.png)
which implies that
![1 ( | ) 9 n∑1 25 ∑n2 - --tr U 2| = - -- |[Y, Yi]|2 - --- |[Y,Xj ]|2 . 2 𝔰0⊥ 4 i=1 4 j=1](/img/revistas/ruma/v47n1/1a02450x.png) |
It is a straightforward computation to see that,
![2 | tr (RY ∘ ad H)|𝔰⊥0 = 9tr RY |𝔷 + tr (RY |𝔫1∩Y⊥) + 4tr(RY |𝔫2) ( n3 ) n1 ∑ 1- 2 3∑ 2 = 9 - 3n3 + 4 |jZkY | - (n1 - 1) - 4 |[Y, Yi]| ( k=1 i=)1 ∑n2 | |2 3 ∑n2 +4 - 2n2 + |∇Xj Y | - -- |[Y,Xj ]|2 j=1 4 j=1 ∑n2 ∑n1 = - 27n - (n - 1) - 8n - 3- |[Y, X ]|2 + 1- |[Y,Y ]|2, 3 1 2 4 j 4 i j=1 i=1](/img/revistas/ruma/v47n1/1a02451x.png)
and (9) becomes
![( ) | 0 = tr ad4 + (R ∘ ad2 ) - 1-U 2 || = (n - 1) + 16n + 81n H Y H 2 |𝔰⊥ 1 2 3 n 0 n 3-∑2 2 1∑ 1 2 - 27n3 - (n1 - 1) - 8n2 - 4 |[Y, Xj ]| + 4 |[Y, Yi]| j=1 i=1 9∑n1 25 ∑n2 - -- |[Y, Yi]|2 - --- |[Y,Xj ]|2 4 i=1 4 j=1 n2 n1 = 54n + 8n - 28 1∑ |[Y, X ]|2 - 8 1∑ |[Y, Y ]|2. 3 2 4 j 4 i j=1 i=1](/img/revistas/ruma/v47n1/1a02452x.png)
Thus,
![n2 n1 ∑ 2 ∑ 2 7 |[Y,Xj ]| + 2 |[Y, Yi]| = 54n3 + 8n2. j=1 i=1](/img/revistas/ruma/v47n1/1a02453x.png) | (10) |
(iii) Now, from the equality given by Corollary 2.4 (ii), we obtain
![n2 n1 2 ∑ |[Y,X ]|2 + 2∑ |[Y, Y]|2 = 24n + 8n = 2 (12n + 4n ) j i 3 2 3 2 j=1 i=1](/img/revistas/ruma/v47n1/1a02454x.png) | (11) |
and the expression (10) (11) gives
(11) gives
![n2 n2 ∑ 2 ∑ 2 5 |[Y, Xj]| = 30n3 ⇔ |[Y, Xj]| = 6n3 for a unit vector Y ∈ 𝔫1. j=1 j=1](/img/revistas/ruma/v47n1/1a02456x.png) |
Finally, it follows from (7) that
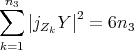 |
and we get a contradiction, since 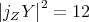 for any unit vectors
for any unit vectors 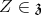 and
and 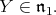 In order to show this last assertion, note that
In order to show this last assertion, note that 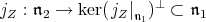 isomorphically (1.1) and by Proposition 2.2, a unit vector
isomorphically (1.1) and by Proposition 2.2, a unit vector 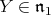 is expressed as
is expressed as 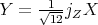 with
with 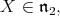
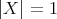 (
(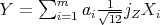 where
where 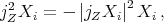
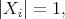 in terms of the orthonormal basis
in terms of the orthonormal basis 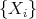 and
and 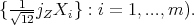 Hence
Hence 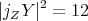 and we have that
and we have that  □
□
[1] Benson P., Payne T., Ratcliff G.; Three-step harmonic solvmanifolds. Geometriae Dedicata 101, 2003, 103-127. [ Links ]
[2] Dotti I, Druetta M.J.; Negatively curved homogeneous Osserman spaces. Differential Geometry and Applications 11, 1999, 163-178. [ Links ]
[3] Druetta M.J.; On harmonic and 2-stein spaces of Iwasawa type. Differential Geometry and its Applications 18, 2003, 351-362. [ Links ]
[4] Druetta M.J.; Carnot spaces and the k-stein condition. Advances in Geometry 6, 2006, 447-473. [ Links ]
[5] Druetta M.J.; The 2-stein condition on generalized Carnot spaces, 2005 (pre-print). [ Links ]
[6] Heber J.; Noncompact homogeneous Einstein spaces. Inventiones Math. 133, 1998, 279-352. [ Links ]
[7] Heber J.; On harmonic and asymptotically harmonic homogeneous spaces. Geometric and Functional Analysis 16 (4), 2006, 869-890. [ Links ]
María J. Druetta
CIEM-Conicet y FAMAF,
Universidad Nacional de Córdoba,
Córdoba 5000, Argentina
druetta@mate.uncor.edu
Recibido: 26 de enero de 2006
Aceptado: 1 de noviembre de 2006














