Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO
Links relacionados
-
 Similares en
SciELO
Similares en
SciELO
Compartir
Revista de la Unión Matemática Argentina
versión impresa ISSN 0041-6932versión On-line ISSN 1669-9637
Rev. Unión Mat. Argent. v.47 n.1 Bahía Blanca ene./jun. 2006
On Complete Spacelike Submanifolds in the De Sitter Space With Parallel Mean Curvature Vector
Rosa Maria S. Barreiro Chaves and Luiz Amancio M. Sousa Jr.
Abstract: The text surveys some results concerning submanifolds with parallel mean curvature vector immersed in the De Sitter space. We also propose a semi-Riemannian version of an important inequality obtained by Simons in the Riemannian case and apply it in order to obtain some results characterizing umbilical submanifolds and a product of submanifolds in the (n + p)-dimensional De Sitter space 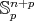 .
.
2000 Mathematics Subject Classification. Primary 53C42, 53A10
Key words and phrases.De Sitter space, Simons type formula, complete spacelike submanifolds, parallel mean curvature vector
Let 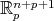 be an
be an 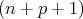 -dimensional real vector space endowed with an inner product of index
-dimensional real vector space endowed with an inner product of index 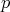 given by
given by
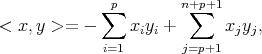
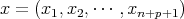 is the natural coordinate of
is the natural coordinate of 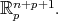
We also define the semi-Riemannian manifold 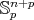 , by
, by
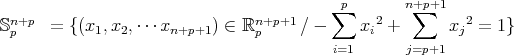 .
.
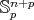 is called
is called  -dimensional De Sitter space of index
-dimensional De Sitter space of index 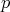 .
.
Let 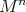 be an
be an 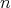 -dimensional semi-Riemannian manifold immersed in
-dimensional semi-Riemannian manifold immersed in 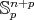 .
. 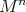 is said to be spacelike if the induced metric on
is said to be spacelike if the induced metric on  from the metric of
from the metric of 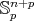 is positive definite.
is positive definite.
From now on, we will consider spacelike submanifolds 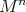 of
of 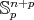 with parallel mean curvature vector
with parallel mean curvature vector 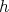 . Let
. Let 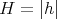 be the mean curvature of
be the mean curvature of  . If
. If 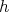 is parallel it is easy to verify that
is parallel it is easy to verify that 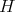 is constant and, when
is constant and, when 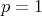 , these two conditions are equivalent. We say that
, these two conditions are equivalent. We say that 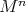 is a maximal submanifold if
is a maximal submanifold if 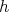 vanishes identically.
vanishes identically.
It was proved by E. Calabi [6] (for 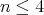 ) and by S.Y. Cheng and S.T. Yau [8] (for all n) that a complete maximal spacelike hypersurface in
) and by S.Y. Cheng and S.T. Yau [8] (for all n) that a complete maximal spacelike hypersurface in 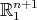 is totally geodesic. In [17], S. Nishikawa obtained similar results for others Lorentzian manifolds. In particular, he proved that a complete maximal spacelike hypersurface in
is totally geodesic. In [17], S. Nishikawa obtained similar results for others Lorentzian manifolds. In particular, he proved that a complete maximal spacelike hypersurface in 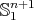 is totally geodesic. We recall that a submanifold
is totally geodesic. We recall that a submanifold 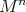 is said totally geodesic if its second fundamental form
is said totally geodesic if its second fundamental form 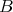 vanishes identically.
vanishes identically.
A. Goddard [11] conjectured that the complete spacelike hypersurfaces of 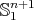 with
with 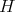 constant must be totally umbilical. The totally umbilical hypersurfaces of
constant must be totally umbilical. The totally umbilical hypersurfaces of 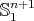 are obtained by intersecting
are obtained by intersecting 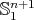 with linear hyperplanes through the origin of
with linear hyperplanes through the origin of 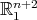 , where
, where 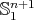 can be viewed as hypersphere of
can be viewed as hypersphere of 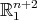 .
.
J. Ramanathan [19] proved Goddard's conjecture for 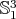 and
and 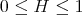 . Moreover, if
. Moreover, if 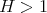 he showed that the conjecture is false as can be seen from an example due to Dajczer-Nomizu [10]. In his proof, Ramanthan used the complex structure of
he showed that the conjecture is false as can be seen from an example due to Dajczer-Nomizu [10]. In his proof, Ramanthan used the complex structure of  . K. Akutagawa [2] proved that Goddard's conjecture is true when
. K. Akutagawa [2] proved that Goddard's conjecture is true when  and
and  or when
or when 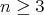 and
and 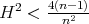 . He also constructed complete spacelike rotation surfaces in
. He also constructed complete spacelike rotation surfaces in 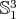 with constant
with constant  satisfying
satisfying 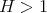 and which are not totally umbilical.
and which are not totally umbilical.
In [15], S. Montiel proved that Goddard's conjecture is true provided that 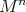 is compact. Furthermore, he exhibited examples of complete spacelike hypersurfaces with constant
is compact. Furthermore, he exhibited examples of complete spacelike hypersurfaces with constant  satisfying
satisfying 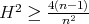 and being not totally umbilical - the so called hyperbolic cylinders (cf. [2] and [13]), which are isometric to the Riemannian product
and being not totally umbilical - the so called hyperbolic cylinders (cf. [2] and [13]), which are isometric to the Riemannian product 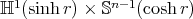 of a hyperbolic line and an
of a hyperbolic line and an  -dimensional sphere of constant seccional curvatures
-dimensional sphere of constant seccional curvatures 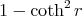 and
and  , respectively. Later, Montiel [16] studied complete spacelike hypersurfaces with constant mean curvature
, respectively. Later, Montiel [16] studied complete spacelike hypersurfaces with constant mean curvature 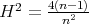 and proved the following result.
and proved the following result.
Theorem 1.1. Let 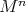 be a complete spacelike hypersurfaces in
be a complete spacelike hypersurfaces in 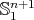 with constant mean curvature
with constant mean curvature 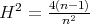 . If
. If 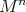 is not connected at infinity, that is, if
is not connected at infinity, that is, if 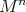 has at least two ends, then
has at least two ends, then 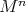 is, up to isometry, a hyperbolic cylinder.
is, up to isometry, a hyperbolic cylinder.
Concerning to submanifolds 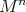 of
of 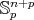 with parallel mean curvature vector we may cite the following remarkable results. In [12], T. Ishihara proved the following theorem that generalizes for higher codimension the result of Cheng-Yau [8]
with parallel mean curvature vector we may cite the following remarkable results. In [12], T. Ishihara proved the following theorem that generalizes for higher codimension the result of Cheng-Yau [8]
Theorem 1.2. Let 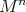 be an n-dimensional complete Riemannian manifold isometrically immersed in
be an n-dimensional complete Riemannian manifold isometrically immersed in  or
or 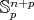 . If
. If 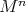 is maximal, then the immersion is totally geodesic and
is maximal, then the immersion is totally geodesic and 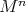 is a Riemannian space of constant curvature.
is a Riemannian space of constant curvature.
In [7], Q.M. Cheng showed that Akutagawa's result [2] is valid for higher codimensional complete spacelike submanifolds in 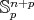 with parallel mean curvature vector. More precisely, he proved the following result.
with parallel mean curvature vector. More precisely, he proved the following result.
Theorem 1.3. Let 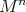 be an n-dimensional complete spacelike submanifold in
be an n-dimensional complete spacelike submanifold in 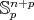 with parallel mean curvature vector. If
with parallel mean curvature vector. If 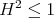 , when n=2 or
, when n=2 or  , when
, when  then
then  is totally umbilical.
is totally umbilical.
In [14], H. Li obtained the following extension of Theorem 1.1.
Theorem 1.4. Let 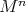 be an n-dimensional complete spacelike submanifold in
be an n-dimensional complete spacelike submanifold in 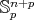 with parallel mean curvature vector. If
with parallel mean curvature vector. If 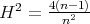 and
and 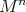 is not connected at infinity, that is, if
is not connected at infinity, that is, if 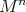 has at least two ends, then
has at least two ends, then 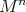 is, up to isometry, a hyperbolic cylinder in
is, up to isometry, a hyperbolic cylinder in 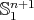 .
.
R. Aiyama [1] studied compact spacelike submanifold in 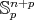 with parallel mean curvature vector and proved the following results:
with parallel mean curvature vector and proved the following results:
Theorem 1.5. Let 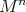 be an n-dimensional compact spacelike submanifold in
be an n-dimensional compact spacelike submanifold in 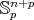 with parallel mean curvature vector. If the normal connection of
with parallel mean curvature vector. If the normal connection of 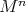 is flat, then
is flat, then 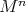 is totally umbilical.
is totally umbilical.
Theorem 1.6. Let 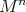 be an n-dimensional compact spacelike submanifold in
be an n-dimensional compact spacelike submanifold in 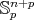 with parallel mean curvature vector. If the sectional curvature of
with parallel mean curvature vector. If the sectional curvature of 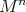 is non-negative, then
is non-negative, then  is totally umbilical.
is totally umbilical.
We point out that L. Alias and A. Romero [3] also obtained results related to complete spacelike submanifolds in 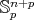 with parallel mean curvature vector.
with parallel mean curvature vector.
Let 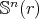 be an n-dimensional sphere in
be an n-dimensional sphere in 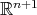 with radius
with radius  and let
and let 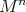 be an
be an 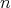 -dimensional submanifold minimally immersed in
-dimensional submanifold minimally immersed in 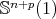 . Denote by
. Denote by 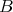 the second fundamental form of this immersion and by
the second fundamental form of this immersion and by 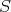 the square of the length of
the square of the length of 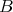 . In his pioneering work, J. Simons [20] proved the following inequality for
. In his pioneering work, J. Simons [20] proved the following inequality for 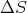
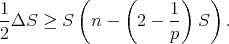 | (1.1) |
As an application of formula (1.1), Simons [20] obtained the following result.
Theorem 1.7. Let 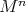 be a closed minimal submanifold of
be a closed minimal submanifold of 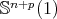 . Then either
. Then either 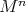 is totally geodesic, or
is totally geodesic, or 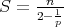 , or
, or 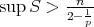 .
.
Two years later, S.S. Chern, M. do Carmo and S. Kobayashi [9], determined all the minimal submanifolds of 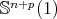 satisfying
satisfying 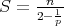 . More precisely, they proved:
. More precisely, they proved:
Theorem 1.8. Let 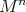 be a closed minimal submanifold of
be a closed minimal submanifold of 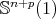 . Assume that
. Assume that 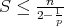 . Then:
. Then:
(i) Either  (and
(and 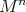 is totally geodesic) or
is totally geodesic) or 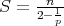 .
.
(ii) 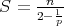 if and only if:
if and only if:
a)  and
and 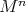 is locally a Clifford torus
is locally a Clifford torus  .
.
b) 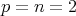 and
and 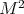 is locally a Veronese surface in
is locally a Veronese surface in 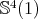 .
.
In the case of a submanifold 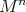 of
of 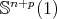 with non-zero parallel mean curvature vector
with non-zero parallel mean curvature vector 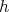 , it is convenient to modify slightly the second fundamental form
, it is convenient to modify slightly the second fundamental form 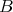 and to introduce the tracelless tensor
and to introduce the tracelless tensor 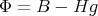 , where
, where 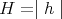 is the mean curvature and g stands for the induced metric on
is the mean curvature and g stands for the induced metric on 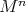 . W. Santos [21] established the following inequality for the Laplacian of
. W. Santos [21] established the following inequality for the Laplacian of 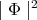
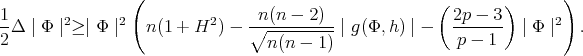
Let 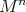 be a complete spacelike maximal submanifold of
be a complete spacelike maximal submanifold of 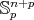 . In [12], T. Ishihara derived the following inequality for
. In [12], T. Ishihara derived the following inequality for 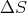
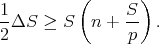 | (1.2) |
As an important application of (1.2), Ishihara proved Theorem 1.2.
If 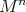 is a spacelike hypersurface of
is a spacelike hypersurface of 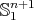 with constant mean curvature
with constant mean curvature 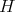 , as in the Riemannian case, it is convenient to consider the tensor
, as in the Riemannian case, it is convenient to consider the tensor 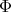 . U.H. Ki, H.J. Kim and H. Nakagawa [13], established the following inequality for
. U.H. Ki, H.J. Kim and H. Nakagawa [13], established the following inequality for 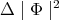
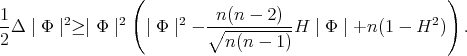 | (1.3) |
By applying (1.3) they obtained a constant  that depends on
that depends on 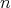 and
and  and such that
and such that  . They also characterized the hyperbolic cylinders as the only complete spacelike hypersurfaces of
. They also characterized the hyperbolic cylinders as the only complete spacelike hypersurfaces of 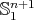 with non-zero constant
with non-zero constant 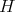 and
and 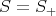 . Moreover, they proved that a complete spacelike hypersurface of
. Moreover, they proved that a complete spacelike hypersurface of 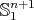 with non-zero constant
with non-zero constant  and non-negative sectional curvature is totally umbilical, provided that
and non-negative sectional curvature is totally umbilical, provided that 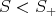 .
.
A. Brasil, G. Colares and O. Palmas [5] obtained the following gap theorem.
Theorem 1.9. Let 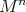 ,
, 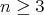 , be a complete spacelike hypersurface in
, be a complete spacelike hypersurface in 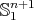 with constant mean curvature
with constant mean curvature 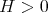 . Then
. Then 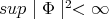 and
and
a)either 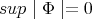 and
and 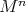 is totally umbilical or
is totally umbilical or
b)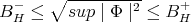 , where
, where  are the roots of the polynomial
are the roots of the polynomial
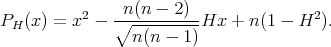
Recently, A. Brasil, R.M.B. Chaves and G. Colares [4] extended the above result for complete spacelike submanifolds in 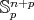 with parallel mean curvature vector.
with parallel mean curvature vector.
Let 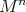 be a spacelike submanifold of
be a spacelike submanifold of 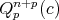 with non-zero parallel mean curvature vector
with non-zero parallel mean curvature vector 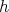 and let
and let  . Define the second fundamental form with respect to the normal direction
. Define the second fundamental form with respect to the normal direction  by
by 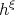 . If
. If 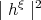 denotes the squared norm of
denotes the squared norm of 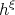 , set
, set 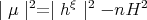 . In [7], Q. M. Cheng proved that
. In [7], Q. M. Cheng proved that
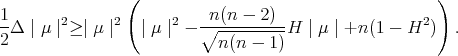 | (1.4) |
Now we are going to state our main results. Theorem 1.10 is a Simons' type inequality for submanifolds in De Sitter space 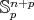 .
.
Theorem 1.10. Let 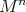 be a spacelike submanifold immersed in
be a spacelike submanifold immersed in 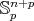 with parallel mean curvature. Then the following inequality holds
with parallel mean curvature. Then the following inequality holds
 | (1.5) |
Next Theorem is a Lorentzian version of results obtained by K. Yano and S. Ishihara [22] and also by S.T. Yau [23] for Riemannian submanifolds.
Theorem 1.11. Let 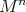 be a complete spacelike submanifold in
be a complete spacelike submanifold in 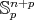 with parallel mean curvature vector and non-negative sectional curvature. If
with parallel mean curvature vector and non-negative sectional curvature. If 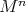 has constant scalar curvature R, then
has constant scalar curvature R, then 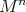 is totally umbilical or a product
is totally umbilical or a product 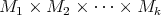 , where each
, where each 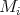 is a totally umbilical submanifold of
is a totally umbilical submanifold of 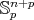 and the
and the 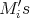 are mutually perpendicular along their intersections.
are mutually perpendicular along their intersections.
As we saw in the Theorem 1.6, compact spacelike submanifolds in 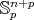 with parallel mean curvature vector and non-negative sectional curvature are totally umbilic.
with parallel mean curvature vector and non-negative sectional curvature are totally umbilic.
The following result is an application of formula (1.5).
Theorem 1.12. Let  be a complete spacelike submanifold in
be a complete spacelike submanifold in 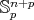 with parallel mean curvature vector. If
with parallel mean curvature vector. If 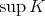 denotes the function that assigns to each point of
denotes the function that assigns to each point of 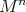 the supremum of the sectional curvatures at that point, there exists a constant
the supremum of the sectional curvatures at that point, there exists a constant  such that if
such that if 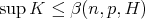 , then either:
, then either:
(i)  and
and 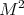 is totally umbilical or
is totally umbilical or
(ii) 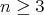 and
and 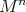 is totally geodesic.
is totally geodesic.
In this section we will introduce some basic facts and notations that will appear on the paper. Let 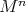 be an
be an 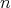 -dimensional Riemannian manifold immersed in
-dimensional Riemannian manifold immersed in 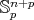 . As the indefinite Riemannian metric of
. As the indefinite Riemannian metric of 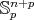 induces the Riemannian metric of
induces the Riemannian metric of 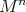 , the immersion is called spacelike. We choose a local field of semi-Riemannian orthonormal frames
, the immersion is called spacelike. We choose a local field of semi-Riemannian orthonormal frames 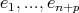 in
in 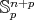 such that, at each point of
such that, at each point of 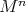 ,
, 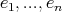 span the tangent space of
span the tangent space of 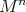 . We make the following standard convention of indices
. We make the following standard convention of indices
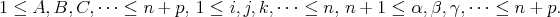
Take the correspondent dual coframe 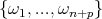 such that the semi-Riemannian metric of
such that the semi-Riemannian metric of 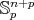 is given by
is given by 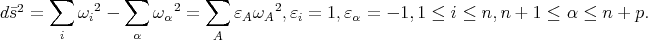 Then the structure equations of
Then the structure equations of 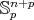 are given by
are given by
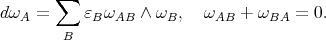 | (2.1) |
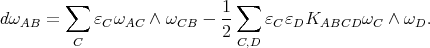 | (2.2) |
 | (2.3) |
Next, we restrict those forms to 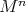 . First of all we get
. First of all we get
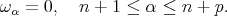 | (2.4) |
So the Riemannian metric of 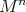 is written as
is written as 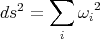 .
.
Since 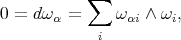 from Cartan's lemma, we can write
from Cartan's lemma, we can write
 | (2.5) |
Set 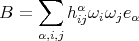 ,
, 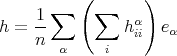 and
and 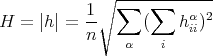 the second fundamental form, the mean curvature vector and the mean curvature of
the second fundamental form, the mean curvature vector and the mean curvature of  , respectively.
, respectively.
Using the structure equations we obtain the Gauss equation
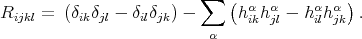 | (2.6) |
The scalar curvature 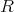 is given by
is given by
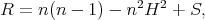 | (2.7) |
where 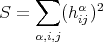 is the squared norm of the second fundamental form of
is the squared norm of the second fundamental form of 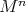 .
.
We also have the structure equations of the normal bundle of 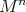
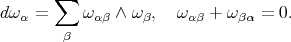 | (2.8) |
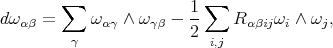 | (2.9) |
where
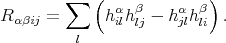 | (2.10) |
The covariant derivatives 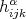 of
of  satisfy
satisfy
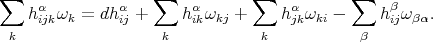 | (2.11) |
Then, by exterior differentiation of (2.5), we obtain the Codazzi equation
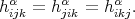 | (2.12) |
Similarly, we have the second covariant derivatives  of
of 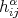 so that
so that
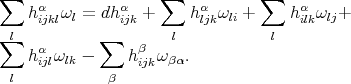 | (2.13) |
By exterior differentiation of (2.11), we can get the following Ricci formula
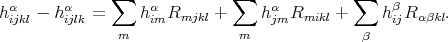 | (2.14) |
The Laplacian 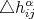 of
of 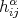 is defined by
is defined by 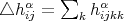 . From (2.12) and (2.14), we have
. From (2.12) and (2.14), we have
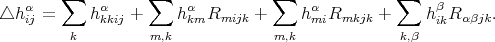 | (2.15) |
If 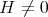 , we choose
, we choose 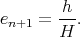 Thus
Thus
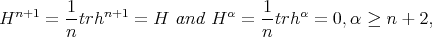 | (2.16) |
where 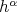 denotes the matrix
denotes the matrix ![[h α]. ij](/img/revistas/ruma/v47n1/1a09254x.png)
From (2.6), (2.10), (2.15) and (2.16) it is straightforward to verify that
![1 ∑ ∑ -△S = (hαijk)2 + n h αijH αij+ 2 α,i,j,k α,i,j 2 2 ∑ n+1 α 2 (nS - n H ) - nH tr(h (h ))+ α ∑ ∑ [tr(hαh β)]2 + N (h αhβ - hβh α), α,β α,β](/img/revistas/ruma/v47n1/1a09255x.png) | (2.17) |
where 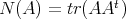 , for all matrix
, for all matrix ![A = [aij].](/img/revistas/ruma/v47n1/1a09257x.png)
Recall that 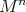 is a submanifold with parallel mean curvature vector
is a submanifold with parallel mean curvature vector  if
if 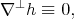 where
where 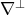 is the normal connection of
is the normal connection of 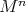 in
in 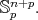 Note that this condition implies that
Note that this condition implies that 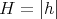 is constant and
is constant and
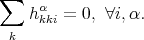 | (2.18) |
We will need the following generalized Maximum Principle due to Omori and Yau (cf. [18] and [23]).
Lemma 2.1. Let 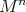 be a complete Riemannian manifold with Ricci curvature bounded from below and let
be a complete Riemannian manifold with Ricci curvature bounded from below and let  be a
be a 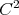 -function which is bounded from below on
-function which is bounded from below on 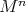 . Then there is a sequence of points
. Then there is a sequence of points 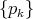 in
in 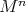 such that
such that
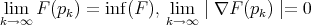 and
and 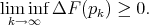
We also will need the following algebraic Lemma (for a proof see [21]).
Lemma 2.2. Let 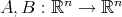 be symmetric linear maps such that
be symmetric linear maps such that 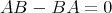 and
and 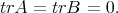 Then
Then
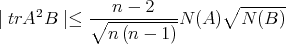 | (2.19) |
and the equality holds if and only if  of the eigenvalues
of the eigenvalues 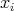 of
of  and the corresponding eigenvalues
and the corresponding eigenvalues 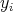 of
of 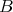 satisfy
satisfy
 | (2.20) |
3. Proof of Simons' type Inequality
Proof of Theorem 1.10. If 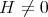 , set
, set 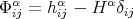 and consider the following symmetric tensor
and consider the following symmetric tensor
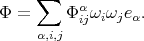 | (3.1) |
It is easy to check that 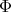 is traceless and
is traceless and
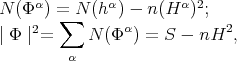 | (3.2) |
where  denotes the matrix
denotes the matrix ![[Φα ] ij](/img/revistas/ruma/v47n1/1a09290x.png) .
.
Because  is parallel, we have
is parallel, we have 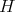 constant. Moreover, as
constant. Moreover, as 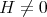 , we can choose a local field of orthonormal frames
, we can choose a local field of orthonormal frames  such that
such that 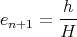 . With this choice (2.16) implies that
. With this choice (2.16) implies that
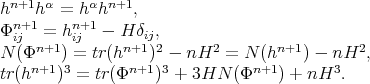 | (3.3) |
 | (3.4) |
Since 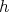 is parallel, from (2.17), (3.2), (3.3) and (3.4) we have
is parallel, from (2.17), (3.2), (3.3) and (3.4) we have
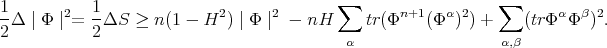 | (3.5) |
As the matrices  and
and 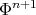 are traceless and the matrix
are traceless and the matrix 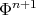 comutes with all the matrices
comutes with all the matrices  , we can apply Lemma 2.2 in order to obtain
, we can apply Lemma 2.2 in order to obtain
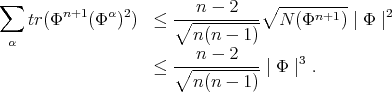 | (3.6) |
Due to Cauchy-Schwarz inequality we can write
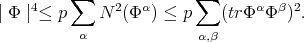 | (3.7) |
It follows from (3.5), (3.6) and (3.7) that formula (1.5) holds.
If  ,
, 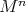 is said to be maximal. In this case, from (1.2) we have
is said to be maximal. In this case, from (1.2) we have
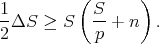 | (3.8) |

4. Proofs of Theorems 1.11 and 1.12
Proof of Theorem 1.11. Since the mean curvature vector 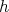 is parallel and
is parallel and 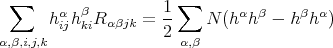 , from (2.15) we have
, from (2.15) we have
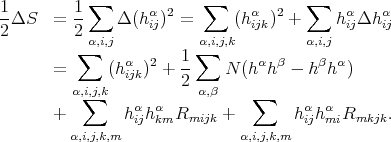 | (4.1) |
Next, we will obtain a pointwise estimate for the last two terms. For each fixed 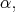 let
let 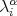 be an eigenvalue of
be an eigenvalue of 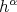 , i.e.
, i.e. 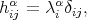 and denote by
and denote by 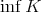 the infimum of the sectional curvatures at a point
the infimum of the sectional curvatures at a point 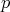 of
of 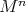 . Then
. Then
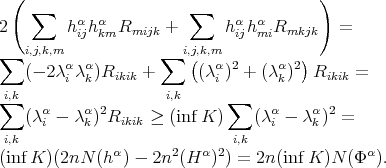 | (4.2) |
It implies that
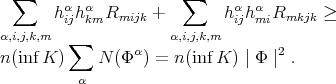 | (4.3) |
As 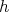 parallel implies
parallel implies  constant, by (2.7) we see that
constant, by (2.7) we see that 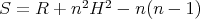 is also constant, thus
is also constant, thus 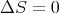 .
.
Since 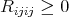 , from (4.1) and (4.3), we get
, from (4.1) and (4.3), we get
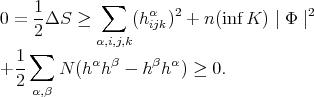 | (4.4) |
It turns out that:
i)  , for all
, for all 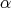 and
and 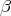 and so the normal bundle of
and so the normal bundle of 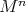 is flat. Hence, all the matrices
is flat. Hence, all the matrices 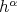 can be diagonalized simultaneously;
can be diagonalized simultaneously;
ii) 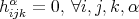 and so the second fundamental form
and so the second fundamental form 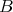 is parallel. In particular, it implies that
is parallel. In particular, it implies that  is constant for all
is constant for all 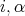 .
.
From i), ii), (4.1) and (4.2) we can write 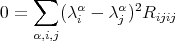 and, since
and, since  , we obtain
, we obtain 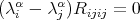 .
.
Consequentely, we may apply the same methods used by Ishihara (see [12], Lemmas 5.1, 5.2 and Theorem 1.3) to conclude that 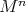 is totally umbilical or a product
is totally umbilical or a product 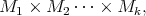 where
where 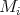 is a totally umbilical submanifold in
is a totally umbilical submanifold in 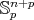 and the
and the  are mutually perpendicular along their intersections.
are mutually perpendicular along their intersections. 
Remark: Let 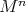 be a complete spacelike submanifold in
be a complete spacelike submanifold in 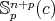 with parallel mean curvature vector and non-negative sectional curvature. In (4.4), we got the inequality
with parallel mean curvature vector and non-negative sectional curvature. In (4.4), we got the inequality 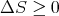 , which shows that
, which shows that 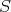 is a subharmonic smooth function. Therefore, if the supremum of
is a subharmonic smooth function. Therefore, if the supremum of 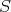 is attained on
is attained on 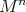 , it follows from the Maximum Principle that S is constant and we have the same conclusions as in Theorem 1.11.
, it follows from the Maximum Principle that S is constant and we have the same conclusions as in Theorem 1.11.
Proof of Theorem 1.12. In the proof of Theorem 1.10 we used the following inequality
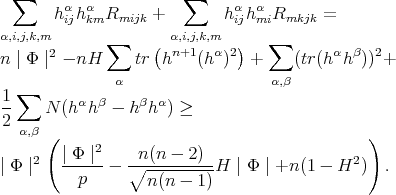 | (4.5) |
Applying the same arguments as in the proof of the inequality (4.3), we obtain
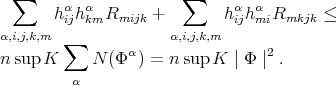 | (4.6) |
For technical reasons, we will write the expression (4.1) for the Laplacian of 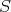 as
as
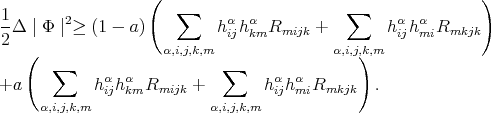 | (4.7) |
Thus, from (4.5), (4.6) and (4.7), if 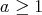 , we have
, we have
![( 1 ∣ Φ ∣2 n(n - 2) -Δ ∣ Φ ∣2 ≥ a ∣ Φ ∣2 ------ ∘----------H ∣ Φ ∣ 2 (p ) n(n - 1)) 2 1 - a +n[1 - H + ------ sup K] . a](/img/revistas/ruma/v47n1/1a09357x.png) | (4.8) |
Using similar arguments as in [14], it is possible to show that 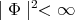 . Therefore, we can apply Lemma 2.1 to the function
. Therefore, we can apply Lemma 2.1 to the function 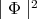 and obtain a sequence of points
and obtain a sequence of points 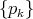 in
in 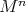 such that
such that
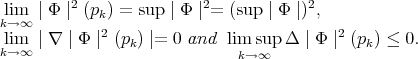 | (4.9) |
By applying inequality (4.8) at 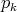 , taking the limit, and using (4.9) we get
, taking the limit, and using (4.9) we get
![( -1- 2 2 sup-∣ Φ-∣2 0 ≥ 2a limk→su∞p Δ ∣ Φ ∣ ≥ (sup ∣ Φ ∣) p ( ) ) - ∘n(n---2)--H sup ∣ Φ ∣ + n[1 - H2 + 1---a- supK] . n (n - 1) a](/img/revistas/ruma/v47n1/1a09364x.png) | (4.10) |
If ![a ( 2 2) sup K ≤ β(n, p,H) = --------------- 4(n - 1) - [p(n - 2) + 4(n - 1)]H 4(a - 1)(n - 1)](/img/revistas/ruma/v47n1/1a09365x.png) , it can be easily checked that
, it can be easily checked that
![( 2 ( ) ) (sup-∣ Φ-∣) - ∘n(n----2)-H sup∣ Φ ∣ + n[1 - H2 + 1 --a- sup K] ≥ 0, p n (n - 1) a](/img/revistas/ruma/v47n1/1a09366x.png)
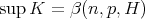 and
and 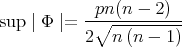 .
. Thus, if 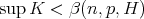 , from (4.10) and the last inequality we conclude that
, from (4.10) and the last inequality we conclude that 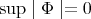 and
and 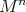 is totally umbilical.
is totally umbilical.
If 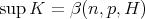 , we will suppose that
, we will suppose that 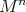 is not totally umbilical and derive a contradiction. First, let us prove that
is not totally umbilical and derive a contradiction. First, let us prove that  . Notice that
. Notice that
![( ) (sup ∣ Φ ∣)2 n(n - 2) (1 - a) (sup ∣ Φ ∣)2 ------------ ∘----------H sup ∣ Φ ∣ + n[1 - H2 + ------ sup K] = 0. p n (n - 1) a](/img/revistas/ruma/v47n1/1a09375x.png)
It shows that all the estimates used to obtain inequality (4.10) turn into equalities. More precisely, (3.6) and (3.7) can now be written as
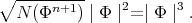 | (4.11) |
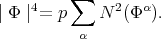 | (4.12) |
As mentioned before, taking subsequences if necessary, we can arrive to a sequence 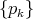 in
in 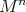 , which satisfies (4.9) and such that
, which satisfies (4.9) and such that
 | (4.13) |
By evaluating (4.11) at 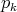 , taking the limit for
, taking the limit for 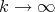 and using (4.13) it gives
and using (4.13) it gives
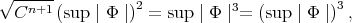 | (4.14) |
Since 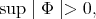 we have
we have
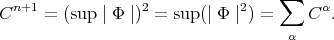 | (4.15) |
Hence, 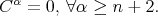 By evaluating (4.12) at
By evaluating (4.12) at 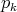 and taking the limit for
and taking the limit for 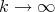 , from (4.13) and (4.15), we get
, from (4.13) and (4.15), we get
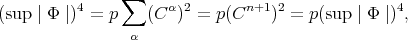
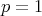 .
. Next, let us prove that 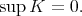 Since
Since 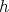 is parallel and the equality holds in (4.6) and (4.7), we arrive to
is parallel and the equality holds in (4.6) and (4.7), we arrive to
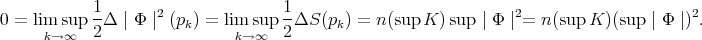
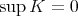 .
. Now we are in position to prove that 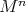 is totally umbilical. Observe that
is totally umbilical. Observe that 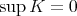 and
and 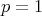 yield
yield
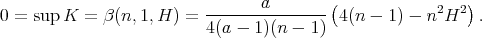
Hence 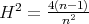 . In this case, according to Montiel (cf. [16], Proposition 2), either
. In this case, according to Montiel (cf. [16], Proposition 2), either 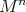 is a totally umbilical hypersurface or
is a totally umbilical hypersurface or  and the supremum of the scalar curvature of
and the supremum of the scalar curvature of 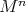 is equal to
is equal to 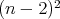 .
.
As 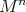 is not totally umbilical, we conclude that the supremum of the scalar curvature of
is not totally umbilical, we conclude that the supremum of the scalar curvature of 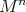 is equal to
is equal to 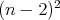 , which contradicts the fact that
, which contradicts the fact that 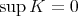 . Therefore,
. Therefore, 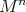 is totally umbilical.
is totally umbilical.
Because  is arbitrary, taking the limit for
is arbitrary, taking the limit for  in
in ![( ) supK ≤ β(n, p,H) = -------a------- 4(n - 1) - [p(n - 2)2 + 4(n - 1)]H2 4(a - 1)(n - 1)](/img/revistas/ruma/v47n1/1a09411x.png) , we get
, we get ![----1----( 2 2) supK ≤ β(n, p,H) = 4(n - 1) 4(n - 1) - [p(n - 2) + 4(n - 1)]H](/img/revistas/ruma/v47n1/1a09412x.png) .
.
Moreover, since 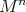 is totally umbilical, if
is totally umbilical, if 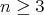 we obtain
we obtain
![1 ( ) 1 - H2 = sup K ≤ --------- 4(n - 1) - [p(n - 2)2 + 4(n - 1)]H2 , 4(n - 1)](/img/revistas/ruma/v47n1/1a09415x.png) thus
thus
 , which implies
, which implies  and shows that
and shows that 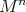 is totally geodesic.
is totally geodesic. 
Acknowledgements. The authors would like to express their thanks to Fernanda Ester C. Camargo for valuable comments and suggestions about this paper, as well as to the referee for his careful reading of the original manuscript. This work was carried out while the second author was visiting the Institute of Mathematics and Statistics at the University of São Paulo (Brazil). He would like to thank Professor Claudio Gorodski and Professor Paolo Piccione for the warm hospitality and financial support, during his visit.
[1] R. Aiyama, Compact spacelike m-submanifolds in a pseudo-Riemannian sphere 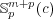 , Tokyo J. Math. 18 no 1 (1995), 81-90. [ Links ]
, Tokyo J. Math. 18 no 1 (1995), 81-90. [ Links ]
[2] K. Akutagawa, On spacelike hypersurfaces with constant mean curvature in the de Sitter space, Math. Z. 196 (1987), 13 - 19. [ Links ]
[3] L.J. Alias and A. Romero, Integral formulas for compact spacelike n-submanifolds in De Sitter spaces. Applications to the parallel mean curvature vector case, Manuscripta Math. 87(1995), 405-416. [ Links ]
[4] A. Brasil, R.M.B. Chaves and G. Colares, Rigidity results for submanifolds with parallel mean curvature vector in De Sitter space, Glasg. Math. J. 48 (2006), 1-10. [ Links ]
[5] A. Brasil, G. Colares and O. Palmas, Complete spacelike hypersurfaces with constant mean curvature in the de Sitter space: A gap theorem, Illinois J. of Math, 47 (2003). [ Links ]
[6] E. Calabi, Examples of Bernstein problems for some nonlinear eqquations, Math. Proc. Cambridge Phil. Soc. 82 (1977), 489-495. [ Links ]
[7] Q.M. Cheng , Complete space-like submanifolds in de Sitter space with parallel mean curvature vector, Math. Z. 206 (1991), 333-339. [ Links ]
[8] S.Y. Cheng and S.T. Yau, Maximal spacelike hypersurfaces in the Lorentz-Minkowski spaces, Ann. of Math.(2) 104 (1976), 407-419. [ Links ]
[9] S.S. Chern, M. do Carmo and S. Kobayashi, Minimal submanifolds of the sphere with second fundamental form of constant length, Functional Analysis and Related Fields (F. Browder, ed.), Springer-Verlag, Berlin, (1970). [ Links ]
[10] M. Dajczer and K. Nomizu, On the flat surfaces in 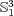 and
and 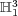 , Manifolds and lie Groups Birkauser, Boston, (1981). [ Links ]
, Manifolds and lie Groups Birkauser, Boston, (1981). [ Links ]
[11] A.J. Goddard, Some remarks on the existence of spacelike hypersurfaces of constant mean curvature, Math. Proc. Cambridge Phil. Soc. 82 (1977), 489-495. [ Links ]
[12] T. Ishihara, Maximal spacelike submanifolds of a pseudo-Riemannian space of constant curvature, Mich. Math. J. 35 (1988), 345-352. [ Links ]
[13] U.H. Ki, H.J. Kim and H. Nakagawa, On spacelike hypersurfaces with constant mean curvature of a Lorentz space form, Tokyo J.Math. 14 (1991), 205-216. [ Links ]
[14] H. Li, Complete spacelike submanifolds in de Sitter space with parallel mean curvature vector satisfying  , Annals of Global Anal. and Geom. 15 (1997), 335-345. [ Links ]
, Annals of Global Anal. and Geom. 15 (1997), 335-345. [ Links ]
[15] S. Montiel, An integral inequality for compact spacelike hypersurfaces in the de Sitter space and applications to case of constant mean curvature, Indiana Univ. Math. J. 37 (1988), 909-917. [ Links ]
[16] S. Montiel, A characterization of hyperbolic cylinders in the de Sitter space, Tohoku Math. J. 48 (1996), 23-31. [ Links ]
[17] S. Nishikawa, On spacelike hypersurfaces in a Lorentzian manifold, Nagoya Math. J. 95 (1984), 117-124. [ Links ]
[18] H. Omori, Isometric Immersions of Riemannian manifolds, J. Math. Soc. Japan 19 (1967), 205-214. [ Links ]
[19] J. Ramanathan, Complete spacelike hypersurfaces of constant mean curvature in de Sitter space, Indiana University Math. J. 36 (1987), 349-359. [ Links ]
[20] J. Simons, Minimal varieties in Riemannian manifolds, Ann. of Math. 88 (1968), 62-105. [ Links ]
[21] W. Santos, Submanifolds with parallel mean curvature vector in spheres, Tohoku Math. J. 46 (1994), 403-415. [ Links ]
[22] S.T. Yau, Submanifolds with constant mean curvature II, Amer. J. Math. 97 (1975), 78-100. [ Links ]
[23] S.T. Yau, Harmonic functions on complete Riemannian manifolds, Comm. Pure Appl. Math. 28 (1975), 201-228. [ Links ]
[24] K. Yano and S. Ishihara, Submanifolds with parallel mean curvature vector, J. Diff. Geom. 6 (1971), 95-118. [ Links ]
Rosa Maria S. Barreiro Chaves
Instituto de Matemática e Estatística
Universidade de São Paulo, Rua do Matão, 1010,
São Paulo - SP, Brazil, CEP 05508-090
rosab@ime.usp.br
Luiz Amancio M. Sousa Jr.
Departamento de Matemática e Estatística
Universidade Federal do Estado do Rio de Janeiro, Avenida Pasteur, 458,
Urca, Rio de Janeiro - RJ, Brazil, CEP 22290-240
amancio@impa.br
Recibido: 17 de noviembre de 2005
Aceptado: 22 de septiembre de 2006














