Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO
Related links
-
 Similars in
SciELO
Similars in
SciELO
Share
Revista de la Unión Matemática Argentina
Print version ISSN 0041-6932On-line version ISSN 1669-9637
Rev. Unión Mat. Argent. vol.47 no.1 Bahía Blanca Jan./June 2006
Integrability of f-structures on generalized flag manifolds
Sofía Pinzón
Financiado parcialmente por COLCIENCIAS, contract No. 138-2004.
Abstract: Here we consider a generalized flag manifold 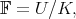 and a differential structure
and a differential structure  which satisfy
which satisfy 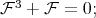 these structures are called f-structures. Such structure
these structures are called f-structures. Such structure 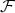 determines in the tangent bundle of
determines in the tangent bundle of  some
some  invariant distributions. Since flag manifolds are homogeneous reductive spaces, they certainly have combinatorial properties that allow us to make some easy calculations about integrability conditions for
invariant distributions. Since flag manifolds are homogeneous reductive spaces, they certainly have combinatorial properties that allow us to make some easy calculations about integrability conditions for 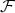 itself and the distributions that it determines on
itself and the distributions that it determines on 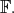 An special case corresponds to the case
An special case corresponds to the case 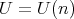 , the unitary group, this is the geometrical classical flag manifold and in fact tools coming from graph theory are very useful.
, the unitary group, this is the geometrical classical flag manifold and in fact tools coming from graph theory are very useful.
A tensor field  of type (1,1) on a Riemannian manifold is called an
of type (1,1) on a Riemannian manifold is called an  -structure if
-structure if  , and almost complex if
, and almost complex if 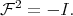 Obviously, an almost complex structure is also an
Obviously, an almost complex structure is also an 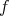 -structure. Integrability of almost complex structures is equivalent to the associate Nijenhuis tensor being null. In [7] Ishihara and Yano present an analogous theorem in the case of
-structure. Integrability of almost complex structures is equivalent to the associate Nijenhuis tensor being null. In [7] Ishihara and Yano present an analogous theorem in the case of 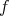 -structures. We use their results to study integrability conditions when one generalized flags manifold are consider.
-structures. We use their results to study integrability conditions when one generalized flags manifold are consider.
Let 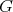 be a semisimple Lie group. A generalized flag manifold with Lie group
be a semisimple Lie group. A generalized flag manifold with Lie group 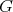 is a reductive homogeneous space
is a reductive homogeneous space 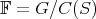 where
where 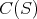 is a centralizer of a torus. This manifold can be expressed as a
is a centralizer of a torus. This manifold can be expressed as a 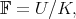 where
where 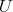 is the compact connected form of
is the compact connected form of 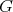 and
and 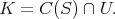 This manifold and its tangent space
This manifold and its tangent space 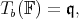 have a characterization in terms of the corresponding root system terms. We will consider along this paper a generalized flag manifold together with an invariant metric
have a characterization in terms of the corresponding root system terms. We will consider along this paper a generalized flag manifold together with an invariant metric 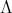 and an
and an 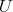 -invariant
-invariant 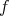 -structure
-structure 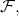 meaning that
meaning that 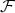 commute with the adjoint action of
commute with the adjoint action of  Ww will denote by
Ww will denote by 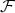 the complexification of this
the complexification of this 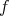 -structure, which is diagonalizable with eigenvalues
-structure, which is diagonalizable with eigenvalues 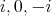 and eigenspaces
and eigenspaces 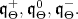 In analogy with the almost complex case we will distinguish between vectors of types
In analogy with the almost complex case we will distinguish between vectors of types  ,
,  or
or 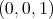 corresponding to the eigenvalues of
corresponding to the eigenvalues of 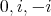 respectively.
respectively.
The integrability of 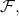 the distributions that it determines in
the distributions that it determines in 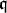 and the properties of that distributions are our central topic. We characterize in root terms and in graph theoretical terms the integrability conditions.
and the properties of that distributions are our central topic. We characterize in root terms and in graph theoretical terms the integrability conditions.
In particular, we get that the only integrable 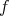 -structure, different from the null structure, in the maximal classical flag manifold is the structure which corresponds to the integrable almost complex structure, that is, in graph theoretic terms which corresponds to the canonical tournament.
-structure, different from the null structure, in the maximal classical flag manifold is the structure which corresponds to the integrable almost complex structure, that is, in graph theoretic terms which corresponds to the canonical tournament.
Theorem A necessary and sufficient condition for 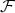 to be integrable is that
to be integrable is that  Therefore
Therefore 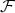 is integrable if in
is integrable if in 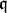 there are not exist triples of type
there are not exist triples of type 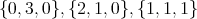 or
or 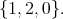 In
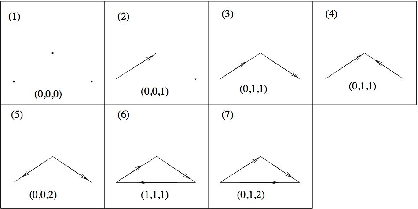
In 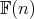 this condition is equivalent to the associated digraph avoiding the subdigraphs (2), (3), (4), (5) or (6) in figure 2, that is, the digraph associated to
this condition is equivalent to the associated digraph avoiding the subdigraphs (2), (3), (4), (5) or (6) in figure 2, that is, the digraph associated to 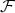 must be isomorphic to the null digraph or the canonical tournament.
must be isomorphic to the null digraph or the canonical tournament.
The Theorem above will appear like Theorem 8.8 and with this result we generalized a Theorem from Burstall [4] given in the context of almost complex structures where he shows that one almost complex structure is integrable if and only if its associated tournament is isomorphic to the canonical tournament, that is, the tournament which does not have three-cycles in root terms it avoids {0,3,0}-triples.
In this section we shall briefly review some general concepts involving generalized flag manifold and some operators and structures which we will use in all this paper. First we need to present a survey about some operators in differential geometry, then we will calculate them specifically on generalized flag manifolds, to this topic we used, specially Props I.3.2, I.3.4, I.3.5 in [8].
2.1. Operators on a general differential manifold.
- Lie derivative. This is the resulting derivative when a tensor field or a differential form is differentiated with respect to a vector field.
1) Lie derivative on tensor fields:![LieX Y = [X, Y]](/img/revistas/ruma/v47n1/1a1051x.png) .
.
2) Lie derivative on tensors: On tensors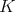 of type (1,r) we get
of type (1,r) we get ![∑r (LieX K)(Y1, ...,Yr) = [X, K(Y1, ...,Yr)] - K(Y1, ...,[X, Yi]...,Yr). i=1](/img/revistas/ruma/v47n1/1a1053x.png)
(1) 3) Lie derivative on forms: If
 is an
is an  -form, then
-form, then![r ∑ (LieXω)(Y1, ...,Yr) = X ω(Y1, ...,Yr) - w(Y1, ...,[X, Yi]...,Yr). i=1](/img/revistas/ruma/v47n1/1a1056x.png)
(2) - The riemannian invariant connection. Each Riemannian manifold admits a unique metric connection with vanishing torsion, called the Riemannian connection or Levi-Civita connection, and it satisfies
![T(X, Y ) = [X, Y ] - ∇X Y + ∇Y X = 0](/img/revistas/ruma/v47n1/1a1058x.png) and
and 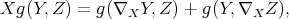 where
where 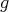 is the metric on the manifold and
is the metric on the manifold and 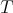 is the torsion tensor [8].
is the torsion tensor [8].
1) The covariant derivative on tensors. Given a tensor field
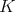 of type
of type  the covariant differential
the covariant differential 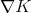 of
of 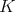 is a tensor field of type
is a tensor field of type 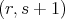 defined as follows.
defined as follows. 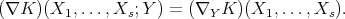
2) The covariant derivative on forms. If
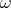 is an
is an  -form, then
-form, then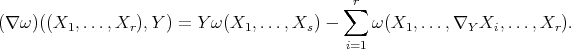
- Exterior derivative on forms. Exterior differentiation
 can be characterize as follow:
can be characterize as follow: 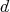 is a degree-increasing
is a degree-increasing 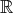 -linear mapping, that is if
-linear mapping, that is if  is a
is a 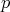 form,
form, 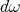 is a
is a  -form;
-form;- signed derivation w.r.t. the wedge product, that is, if
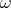 is a
is a 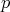 -form and
-form and 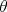 is a
is a 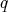 -form, then
-form, then 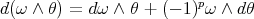 ;
; 
- For 0-forms
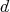 is defined by
is defined by 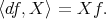
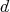 extends to
extends to  -forms coefficient-wise, using basis expansions, resulting in
-forms coefficient-wise, using basis expansions, resulting inHere
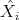 means that you not consider that component. On functions the 1-form
means that you not consider that component. On functions the 1-form  is defined by
is defined by 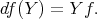 Otherwise we get
Otherwise we get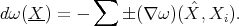
(3) On 1-forms we get
![2dω(X, Y) = X( ω(Y )) - Y ω(X) - ω([X, Y]).](/img/revistas/ruma/v47n1/1a1094x.png)
(4) On 2-forms,
![3dω(X, Y, Z) = Cyclic {X ω(Y,Z) - ω([X, Y ],Z)}](/img/revistas/ruma/v47n1/1a1095x.png)
(5) where
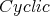 is the cyclic symmetrization operator w.r.t. the vector fields involved. See [8] Prop. I.3.11. By duality we get
is the cyclic symmetrization operator w.r.t. the vector fields involved. See [8] Prop. I.3.11. By duality we get 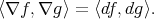
A generalized flag manifold is and homogeneous space of the form 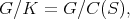 where
where 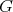 is a semisimple compact Lie group and
is a semisimple compact Lie group and 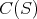 is the centralizer of some torus
is the centralizer of some torus  in
in 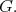 If the torus
If the torus 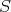 is maximal, say
is maximal, say 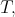 then
then 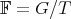 is called a maximal (full) flag manifold, if
is called a maximal (full) flag manifold, if  is not maximal,
is not maximal, 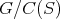 is called a partial flag manifold. Lets us describe generalized flag manifolds associated with the Lie group
is called a partial flag manifold. Lets us describe generalized flag manifolds associated with the Lie group 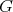 in terms of the root systems associated with the corresponding semisimple Lie algebra
in terms of the root systems associated with the corresponding semisimple Lie algebra 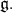
Assume  complex and let
complex and let 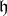 be a Cartan subalgebra of
be a Cartan subalgebra of 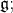 denote by
denote by  and
and 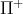 the root system and the positive root system , respectively, (of
the root system and the positive root system , respectively, (of 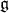 with respect to
with respect to 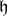 ) and
) and
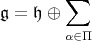
its root decomposition, where ![𝔤α = {X ∈ 𝔤 : [H, X] = α(H)X, ∀H ∈ 𝔥}](/img/revistas/ruma/v47n1/1a10118x.png) ,
, 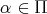 , is the one-dimensional complex root space corresponding to
, is the one-dimensional complex root space corresponding to 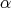 . As
. As  is the generator of
is the generator of 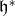 (the dual of
(the dual of 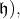 we have the elements
we have the elements 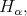 defined by
defined by 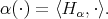 Denote by
Denote by  the subspace of
the subspace of  generated over
generated over 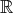 by
by 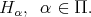 Choose now
Choose now  a simple root system, take
a simple root system, take  and denote by
and denote by  the set of roots generated by
the set of roots generated by 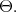 Each subset
Each subset 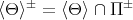 splits
splits 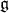 as follows
as follows
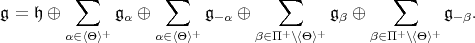 | (6) |
Fix a Weyl basis of 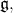 that is, a set of vectors
that is, a set of vectors 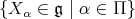 which satisfies
which satisfies ![[X ,X ] = H α -α α](/img/revistas/ruma/v47n1/1a10139x.png) or equivalently
or equivalently 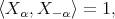 since
since ![[X ,X ] = ⟨X ,X ⟩H α -α α -α α](/img/revistas/ruma/v47n1/1a10141x.png) and
and ![[X α,X β] = m α,βX α+ β](/img/revistas/ruma/v47n1/1a10142x.png) with
with 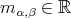 ,
, 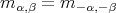 and
and 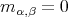 if
if  .
.
Let now
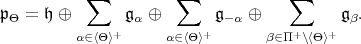 | (7) |
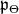 is the parabolic subalgebra determined by
is the parabolic subalgebra determined by  in
in  Then equation ((6)) becomes
Then equation ((6)) becomes
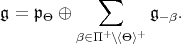 | (8) |
Then the generalized flag manifold 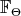 associated to
associated to 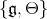 corresponds to the homogeneous space
corresponds to the homogeneous space 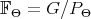 , where
, where 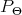 is the normalizer of
is the normalizer of 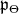 in
in 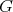 .
.
Denote by 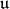 a real compact form of
a real compact form of  , and by
, and by  the connected subgroup associated to
the connected subgroup associated to 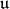 . Assume
. Assume 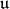 the real subspace generated by
the real subspace generated by 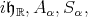 with
with 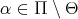 , where
, where 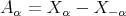 and
and 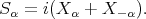 Let
Let 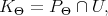 which, by construction, is the centralizer of a torus.
which, by construction, is the centralizer of a torus. 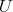 acts in a transitively way on
acts in a transitively way on  and we can write
and we can write 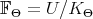 . If
. If  ,
,  correspondes to the maximal flag manifold
correspondes to the maximal flag manifold 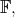 otherwise it corresponds to a partial flag manifold.
otherwise it corresponds to a partial flag manifold.
The generalized flag manifold 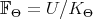 is a reductive homogeneous space. In fact let
is a reductive homogeneous space. In fact let 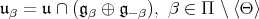 and
and
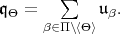 Then,
Then,
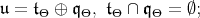
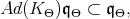 that is,
that is, ![[𝔱 ,𝔮 ] ⊂ 𝔮 , Θ Θ Θ](/img/revistas/ruma/v47n1/1a10179x.png)
and 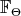 satisfies the condition to be a a reductive homogeneous space (see [8]).
satisfies the condition to be a a reductive homogeneous space (see [8]).
Denote by 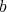 the origin of
the origin of 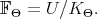 We identify with
We identify with 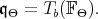 This identification is given by
This identification is given by 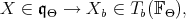 that is, by evaluation of
that is, by evaluation of 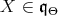 in
in 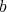 like a vector field in
like a vector field in 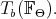 The tangent space to
The tangent space to 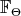 in
in 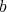 is, naturally, identify with
is, naturally, identify with 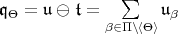 generated by
generated by 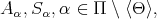 where
where 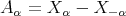 and
and 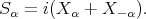 In the same way, the complexified tangent space of
In the same way, the complexified tangent space of 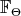 is identified with
is identified with 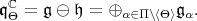
One special case of flag manifold corresponds to the geometrical or classical flag manifold, in this case 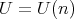 the unitary group and
the unitary group and 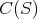 has to be conjugate to some subgroup
has to be conjugate to some subgroup 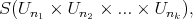 with
with 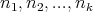 positive integers and
positive integers and  . If
. If  , the homogeneous space
, the homogeneous space 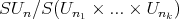 can be identified with the set of "partial flags"
can be identified with the set of "partial flags"  that is, the manifold of the flags
that is, the manifold of the flags 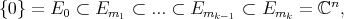 where
where 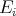 is an
is an 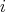 -dimensional subspace of
-dimensional subspace of 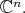 The flag manifold corresponding to the case
The flag manifold corresponding to the case 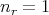 for all
for all 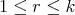 will be denoted by
will be denoted by 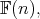 the space of "full flags" or maximal flags in
the space of "full flags" or maximal flags in 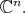 Each flag consists of the sequence
Each flag consists of the sequence 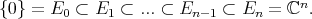 In particular, the vectors
In particular, the vectors 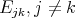 y
y 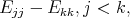 is a Weyl basis for
is a Weyl basis for 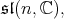 and
and 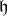 is the subalgebra of diagonal matrices in
is the subalgebra of diagonal matrices in 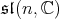 ,
, 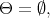 then
then 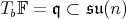 is spanned by
is spanned by 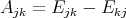 and
and 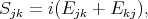 where
where 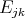 is the usual canonical basis in
is the usual canonical basis in 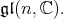
Example 1.1: Consider
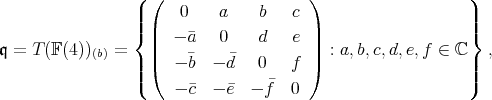
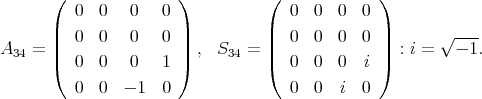
A  -invariant Riemannian metric
-invariant Riemannian metric  in
in 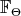 is completely determined by its values in
is completely determined by its values in 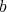 , that is, by an inner product
, that is, by an inner product 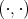 in
in  invariant under the associated action of
invariant under the associated action of 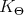 ([3]). Any inner product in
([3]). Any inner product in 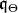 , invariant under the associated action of
, invariant under the associated action of  has the form
has the form 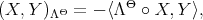 with
with  definite with respect to the Cartan-Killing form and
definite with respect to the Cartan-Killing form and  is the Hadamard product or term by term product [3]. The inner product
is the Hadamard product or term by term product [3]. The inner product 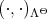 admits a natural extension to a bilinear symmetric form on
admits a natural extension to a bilinear symmetric form on  We use the same notation
We use the same notation 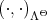 for this form, as well as for the correspondent complexified form
for this form, as well as for the correspondent complexified form 
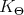 -invariance of
-invariance of 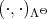 amounts to the Weyl basis being a complex basis of eigenvectors for the action of
amounts to the Weyl basis being a complex basis of eigenvectors for the action of  , in other words in
, in other words in 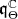 we have
we have
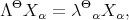 | (9) |
with 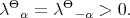 for
for 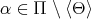
for the real space  the elements of the canonical base
the elements of the canonical base 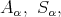 with
with 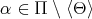 , are eigenvectors for the same eigenvalue
, are eigenvectors for the same eigenvalue 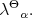 We denote by
We denote by 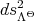 the
the  -invariant metric associated with
-invariant metric associated with  In what follows we will use
In what follows we will use 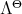 as synonymous of
as synonymous of 
K. Yano [20] in 1961 introduced 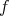 -structure for general manifolds; here we shall be interested in invariant structures. An
-structure for general manifolds; here we shall be interested in invariant structures. An 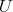 -invariant
-invariant 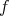 -structure in
-structure in 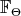 is completely determined by an endomorphism
is completely determined by an endomorphism  , satisfying
, satisfying 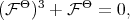 which commutes with the adjoint action of
which commutes with the adjoint action of 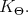 We also denote by
We also denote by 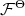 its complexification
its complexification  which is diagonalizable with eigenvalues
which is diagonalizable with eigenvalues 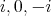 , and denote by
, and denote by  the corresponding eigenspaces. Then we have
the corresponding eigenspaces. Then we have  with
with  The
The 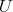 -invariance of
-invariance of 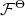 guarantees that
guarantees that 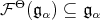 for all
for all 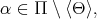 with equality when
with equality when 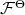 is an invariant almost complex structure (see [17]). Thus
is an invariant almost complex structure (see [17]). Thus 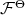 is determined uniquely by the values
is determined uniquely by the values 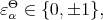
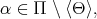 defined by
defined by  . These values satisfy
. These values satisfy 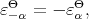 therefore
therefore  is defined by its values in
is defined by its values in 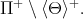 In the sequel we allow some abuse of notation and identify the invariant
In the sequel we allow some abuse of notation and identify the invariant 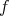 -structure
-structure 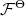 on
on 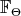 with
with 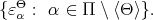 In our invariant context if the
In our invariant context if the 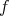 -structure is an invariant almost complex structure this amounts to
-structure is an invariant almost complex structure this amounts to 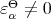 for all
for all 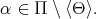
In what follows we shall simplify notation by suppressing the subscript 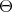 in the context of partial flag manifolds.
in the context of partial flag manifolds.
Denote by 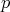 and
and 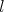 the complementary projections onto the spaces
the complementary projections onto the spaces 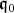 and
and  denoted as
denoted as 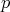 and
and 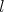 , respectively and defined as follow
, respectively and defined as follow

Since 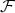 is an
is an 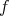 -structure we have
-structure we have
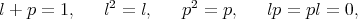 | (11) |
where 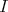 denote the identity. In other words
denote the identity. In other words 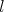 and
and 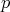 are complementary projection operators in
are complementary projection operators in 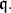
Consider an 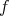 -estructure
-estructure 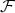 and
and 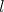 ,
, 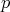 like below. Then:
like below. Then:

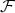 act in
act in  like an almost complex structure and in
like an almost complex structure and in 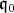 like the null operator.
like the null operator. We are interested in studying integrability conditions for 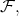 the distributions
the distributions 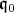 and
and  For this purpose, we need the Nijenhuis tensor which describes the torsion of
For this purpose, we need the Nijenhuis tensor which describes the torsion of  It is given by
It is given by
![N (X, Y ) = 2([F(X), F (Y )] - F ([F (X), Y ]) - F ([X, F (Y )]) - L([X, Y ]).](/img/revistas/ruma/v47n1/1a10319x.png)
Using Weyl basis properties we get
![1∕2N (X ,X ) = [F (X ),F (X )] - F ([F(X ),X ])+ α β α β α β - F ([X α,F (X β)]) - L([X α,X β] = (- ɛαɛβ + ɛαɛα+ β + ɛβ ɛα+β + ɛ2 )[Xα, Xβ]. α+β](/img/revistas/ruma/v47n1/1a10320x.png)


With some simple calculations and using, again, Weyl basis properties we obtain this other identities:
 Riemannian Connection. Since
Riemannian Connection. Since 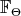 is a naturally reductive homogeneous space its Riemannian connection is given by
is a naturally reductive homogeneous space its Riemannian connection is given by
![2 ∇X Y = [X, Y ]𝔮Θ + 2U (X, Y),](/img/revistas/ruma/v47n1/1a10327x.png) | (16) |
where 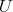 is a symmetric bilinear application
is a symmetric bilinear application 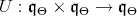 defined by
defined by
![2 ΛΘ(U (X, Y ),Z) = Λ Θ(X, [Y, Z]𝔮Θ) + ΛΘ([Z, X] 𝔮Θ, Y),](/img/revistas/ruma/v47n1/1a10330x.png)
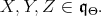
Then the concrete action of the Riemannian connection on the elements of the Weyl basis is given by:
Proposition 6.1. For 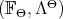 let
let 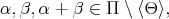 and
and 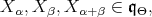 elements in the Weyl base. Then
elements in the Weyl base. Then
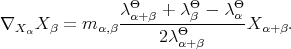 | (17) |
 Kähler form.
Kähler form. 
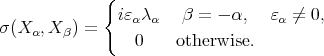
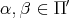 .
.  Derivative in the connection.
Derivative in the connection.
![(d∇F )(X ,X ) = ∇ F X - ∇ F X - F [X ,X ] α β (Xλαα-λβ)(βɛα-ɛβ)+Xβλα+β(ɛαα+ɛβ-2ɛαα+β) β = i------------2(λα+β)------------[X α,X β].](/img/revistas/ruma/v47n1/1a10341x.png)
 Lie derivative:
Lie derivative: ![(Lie F )X = F [X ,X ] - [F X ,X ] Xβ α α β α β = i(ɛα+β - ɛβ)[X α,X β].](/img/revistas/ruma/v47n1/1a10343x.png)
7. Graph theoretic description of 
On  invariant
invariant 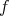 -structures are in 1:1 correspondence with digraphs
-structures are in 1:1 correspondence with digraphs  The correspondence is given by associating with the
The correspondence is given by associating with the  -structure
-structure 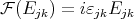 a digraph
a digraph 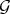 whose vertices are
whose vertices are  and whose arrows are given by the following rules: For
and whose arrows are given by the following rules: For 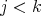
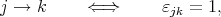
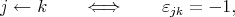
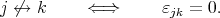
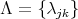 we may identify an invariant metric
we may identify an invariant metric 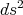 on
on 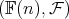 with a positive weighting on the edge set
with a positive weighting on the edge set  of the digraph.
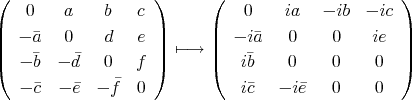
of the digraph. Example 7.1. Again in  let the
let the  -structure
-structure
| |
There exists a complete classification for invariant 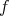 -structures on
-structures on  (see [5]). Here we present, up to isomorphism, the invariant
(see [5]). Here we present, up to isomorphism, the invariant 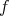 -structures in the case
-structures in the case 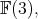 this graphs are the most relevant in our present work.
this graphs are the most relevant in our present work.
| |
We are now ready to present our characterization of integrability in graph theoretical terms (classical case) or in root terms (general case).
8. Integrability via projections
In this section we will use all the formulas given before to establish integrability conditions for 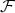 and for the associated distributions
and for the associated distributions 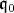 and
and  .
.
First, we will call the root triple 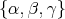 with
with 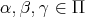 whenever
whenever 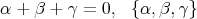 is called a zero-sum triple. Given an invariant
is called a zero-sum triple. Given an invariant 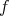 -structure
-structure 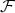 , each root assumes a sign in
, each root assumes a sign in 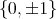 .
.
Roots triples may then be classified by their sign characteristic, which is a triple 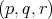
 where
where 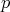 corresponds to the quantity of roots in triple who has
corresponds to the quantity of roots in triple who has 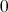 like its eigenvalue,
like its eigenvalue, 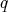 corresponds to the quantity of roots in triple who has
corresponds to the quantity of roots in triple who has 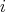 like its eigenvalue and
like its eigenvalue and  corresponds to the quantity of roots in triple who has
corresponds to the quantity of roots in triple who has  like its eigenvalue. There are six possible sign characteristics:
like its eigenvalue. There are six possible sign characteristics: 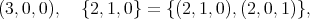


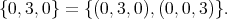
By the Frobenious Theorem we know that a distribution is integrable if and only if it is involutive. Thus 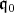 is integrable if and only if
is integrable if and only if ![l[pX α,pX β] = 0,](/img/revistas/ruma/v47n1/1a10395x.png) that is,
that is,
![l[pX α,pX β] = (ɛ2- 1)(ɛ2 - 1)ɛ2 [X α,X β] = 0. α β α+ β](/img/revistas/ruma/v47n1/1a10396x.png) | (18) |
Theorem 8.1. A necessary and sufficient condition for the distribution 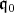 to be integrable is that in
to be integrable is that in 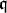 does not admit triples of type
does not admit triples of type 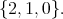 In case of
In case of 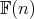 this condition is equivalent to the associated digraph avoiding the subdigraph (2) in Figure 2.
this condition is equivalent to the associated digraph avoiding the subdigraph (2) in Figure 2.
PROOF. By equation (18) 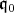 is not integrable in case where
is not integrable in case where  This can only occur when the triple
This can only occur when the triple 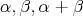 is a
is a 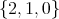 -triple. In the classical case, this corresponds the configuration (2) in Figure 2.
-triple. In the classical case, this corresponds the configuration (2) in Figure 2. 
Now  is integrable if and only if
is integrable if and only if ![p[lX α,lX β] = 0](/img/revistas/ruma/v47n1/1a10407x.png) but
but ![p[lXα, lX β] = (F 2 + 1)[- F 2X α,- F 2Xβ] (19) 2 2 2 = ɛα ɛβ(F + 1)[X α,X β] (20) 2 2 2 = ɛα ɛβ(1 - ɛα+β)[X α,X β]. (21)](/img/revistas/ruma/v47n1/1a10408x.png)
Thus we have the following.
Theorem 8.2. A necessary and sufficient condition for distribution  to be integrable is that in
to be integrable is that in 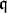 does not amit triples of type
does not amit triples of type  and
and 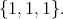 In case of
In case of 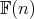 this condition is equivalent to the associated digraph avoiding the subdigraph (3),(4) and (5) in Figure 2.
this condition is equivalent to the associated digraph avoiding the subdigraph (3),(4) and (5) in Figure 2.
PROOF. By equation (21)  is not integrable in case
is not integrable in case 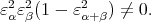 This can only occur when the triple
This can only occur when the triple 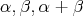 is a
is a 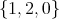 -triple or a
-triple or a  -triple. In the classical case, this corresponds to configurations (3), (4) and (5) in Figure 2.
-triple. In the classical case, this corresponds to configurations (3), (4) and (5) in Figure 2. 
When 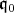 and
and  are integrable, the structure of the submanifolds defined by these distributions and its properties is of interest. We hope to be able to report on this structure in a future communication.
are integrable, the structure of the submanifolds defined by these distributions and its properties is of interest. We hope to be able to report on this structure in a future communication.
Looking for the integrability of 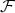 we need another definition from [7].
we need another definition from [7].
Definition 8.3. Assume  integrable and let
integrable and let 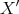 be an arbitrary vector field which is tangent to an integrable manifold of
be an arbitrary vector field which is tangent to an integrable manifold of  It is defined
It is defined  Then
Then 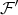 is an almost-complex structure on each integral manifold of
is an almost-complex structure on each integral manifold of 
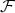 is called partially integrable if both,
is called partially integrable if both,  and
and 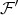 are integrable.
are integrable.
Theorem 8.4. A necessary and sufficient condition for 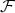 be partially integrable is that one of the following equivalent conditions be satisfied:
be partially integrable is that one of the following equivalent conditions be satisfied:
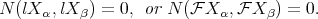
Using Weyl basis properties the Theorem 8.4 is equivalent to the following.
Theorem 8.5. A necessary and sufficient condition for 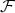 to be partially integrable is that in
to be partially integrable is that in 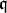 does not admit triples of type {0,3,0}, {1,2,0} and
does not admit triples of type {0,3,0}, {1,2,0} and 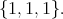 In the case of
In the case of 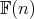 this condition is equivalent to the associated digraph avoiding the subdigraph (3), (4), (5) and (6) in Figure 2.
this condition is equivalent to the associated digraph avoiding the subdigraph (3), (4), (5) and (6) in Figure 2.
PROOF. By Theorem 8.4 is enough to see the conditions 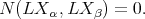 Doing the respective calculations we have
Doing the respective calculations we have
![N (LX α,LX β) = ɛαɛβ(- 1 - ɛβ ɛα+β - ɛαɛα+β + ɛαɛβɛ2α+ β)[X α,X β].](/img/revistas/ruma/v47n1/1a10439x.png)
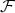 is not integrable in case
is not integrable in case 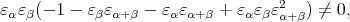 this can only occur when the triples are the type in the Theorem. In the classical case this corresponds to configurations (3), (4), (5) and (6) in Figure 2.
this can only occur when the triples are the type in the Theorem. In the classical case this corresponds to configurations (3), (4), (5) and (6) in Figure 2. 
When 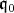 and
and  are both integrables, it is possible to choose a local coordinates system such that the operators
are both integrables, it is possible to choose a local coordinates system such that the operators 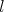 and
and  can be supposed to have the components of the form:
can be supposed to have the components of the form:
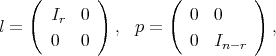
where 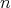 is the dimension of the manifold,
is the dimension of the manifold,  is the rank of
is the rank of 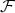 and
and 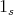 means the
means the  -identity matrix. This coordinate system is called "adapted." Since
-identity matrix. This coordinate system is called "adapted." Since 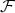 satisfy
satisfy 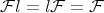 and
and 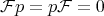 then in an adapted coordinate system, we can express
then in an adapted coordinate system, we can express  in the following way:
in the following way:
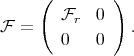
But 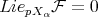 means that the components of
means that the components of 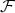 are independent of the coordinates, thus in the next theorem we are interested on this condition in root terms.
are independent of the coordinates, thus in the next theorem we are interested on this condition in root terms.
Theorem 8.6. Suppose that 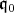 and
and  are both integrable and that an adapted coordinate system has been chosen. A necessary and sufficient condition for the local components of
are both integrable and that an adapted coordinate system has been chosen. A necessary and sufficient condition for the local components of 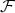 to be functions independent of the coordinates is that
to be functions independent of the coordinates is that 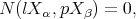 in combinatorial terms a necessary and sufficient condition is that in
in combinatorial terms a necessary and sufficient condition is that in 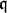 does not admit triples of type
does not admit triples of type 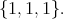 In case of
In case of 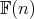 this condition is equivalent to the associated digraph avoiding the subdigraphs (4) and (5) in Figure 2.
this condition is equivalent to the associated digraph avoiding the subdigraphs (4) and (5) in Figure 2.
PROOF. By equation (14) we have the first affirmation and with some calculus we have
![N (lX ,pX ) = ɛ ɛ (1 - ɛ 2)(ɛ ɛ - 1)[X ,X ]. α β α α+β β α α+β α β](/img/revistas/ruma/v47n1/1a10467x.png)
Thus 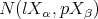 will be different from zero in case
will be different from zero in case 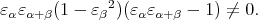
This can only occur when the triple is 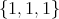 -triple. In the classical case this corresponds to configurations mentioned in the theorem.
-triple. In the classical case this corresponds to configurations mentioned in the theorem. 
When 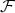 is an almost complex structure integrability is associated with the existence of canonical coordinate systems, which allows us to consider the manifold as a complex manifold as well is known, integrability is equivalent to
is an almost complex structure integrability is associated with the existence of canonical coordinate systems, which allows us to consider the manifold as a complex manifold as well is known, integrability is equivalent to  The following definition in the context of general differential manifolds appears in [7]. Here we present it in the case of flag manifolds.
The following definition in the context of general differential manifolds appears in [7]. Here we present it in the case of flag manifolds.
Definition 8.7. The 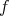 -structure
-structure 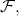 is called integrable if it satisfies the following three conditions:
is called integrable if it satisfies the following three conditions:
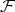 is partially integrable.
is partially integrable.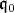 is integrable.
is integrable.- The components of
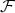 are independent of the coordinates.
are independent of the coordinates.
With Definition 8.7, we arrive at a general integrability theorem for 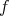 -structures.
-structures.
Theorem 8.8. A necessary and sufficient condition for 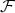 to be integrable is that
to be integrable is that  Therefore
Therefore 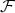 is integrable if in
is integrable if in 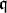 does not admit triples of type
does not admit triples of type  and
and  In
In 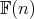 this condition is equivalent to the associated digraph avoiding the subdigraphs (2), (3), (4), (5) and (6) in figure 2, that is, the associated digraph to
this condition is equivalent to the associated digraph avoiding the subdigraphs (2), (3), (4), (5) and (6) in figure 2, that is, the associated digraph to 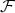 must be isomorphic to the null digraph or canonical tournament.
must be isomorphic to the null digraph or canonical tournament.
PROOF. It is immediate by Definition 8.7 and Theorems 8.1, 8.6 and 8.5. 
Theorem 8.8 is a generalization of the results obtained by Burstall [4] in the case of almost complex structures for classical maximal flag manifolds.
At this point we would like to point out an important connection between integrability and complex structures. It is well known that for a general almost complex structure 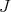 on a differential manifold is parallel, namely
on a differential manifold is parallel, namely 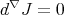 if the manifold with that structure is Kähler. That is, parallelism means that
if the manifold with that structure is Kähler. That is, parallelism means that 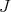 is integrable and the manifold is Kähler.
is integrable and the manifold is Kähler.
Now a manifold with an 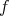 -structure
-structure  will be called Kähler if
will be called Kähler if 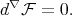
Observe that in the case of generalized flag manifolds integrability condition is stronger than Kähler condition, for example, in 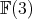 the invariant
the invariant 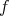 -structures to the digraphs (4) and (5) are Kähler but not integrable.
-structures to the digraphs (4) and (5) are Kähler but not integrable.
[1] M. Arvanitogeorgos. "An introduction to Lie groups and the geometry of homogeneous spaces". American Mathematical Society Books, Vol. 21 2003. [ Links ]
[2] M. Black. "Harmonic Maps into Homogeneous Spaces". Pitman Research Notes, Math series 255, Longman, Harlow, 1991. [ Links ]
[3] A. Borel, Kählerian coset spaces of semi-simple Lie groups. Proc. Nat. Acad. of Sci. (1954), 40:1147-1151. [ Links ]
[4] F.E. Burstall and S. Salamon. "Tournaments, flags and harmonic maps". Math Ann. 277:249-265, 1987. [ Links ]
[5] N. Cohen, M. Paredes, L. A. B. San Martin, C. J. C. Negreiros, S. Pinzón, f-structures on the classical flag manifold which admit (1,2)-symplectic metrics. Tôhoku Math. J. 57(2) (2005), 262-271. [ Links ]
[6] G. Frobenius, "Uber die Unzerlegbaren diskreten Bewegungsgruppen Sitzungsber". König. Prenss. Acad. Wiss. Berlin 29:654-665, 1911. [ Links ]
[7] S. Ishihara and K. Yano. "On integrability Conditions of a structure 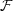 satisfying
satisfying 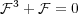 ". Quart. J. Math. Oxford (2), 15:217-222, 1964. [ Links ]
". Quart. J. Math. Oxford (2), 15:217-222, 1964. [ Links ]
[8] S. Kobayashi and K. Nomizu. Foundations of Differential Geometry, Vol. 1. Interscience Publishers, 1963. [ Links ]
[9] S. Kobayashi and K. Nomizu. Foundations of Differential Geometry, Vol. 2, Interscience Publishers, 1969. [ Links ]
[10] A. Lichnerowicz. "Applications harmoniques et variétés Kähleriennes". Symp. Math. 3 (Bologna), 341-402, 1970. [ Links ]
[11] X. Mo and C.J.C. Negreiros. "Horizontal f-estructures,  -matrices and Equiharmonic moving flags". Relatorio de Pesquisa, Instituto de Matemática, Estatística e Computaçao Científica, Universidade Estadual de Campinas, Brasil, 1999. [ Links ]
-matrices and Equiharmonic moving flags". Relatorio de Pesquisa, Instituto de Matemática, Estatística e Computaçao Científica, Universidade Estadual de Campinas, Brasil, 1999. [ Links ]
[12] Nijenhuis, A. " forming sets of eigenvectors." Indag. Math. 13: 200-212, 1951. [ Links ]
forming sets of eigenvectors." Indag. Math. 13: 200-212, 1951. [ Links ]
[13] S. Pinzón. Variedades Bandeira, f-Estruturas e Métricas (1,2)-Simpléticas. Ph. D. tesis, Universidade Estadual de Campinas, Brasil, 2003. [ Links ]
[14] J.H. Rawnsley. "f-Structures, f-Twistor Spaces and Harmonic Maps". Lec. Notes in Math., 1164, Springer 1986. [ Links ]
[15] S. Salamon. Harmonic and holomorphic maps. Lec. Notes in Math. 1164, Springer 1986. [ Links ]
[16] L. A. B. San Martin. Algebras de Lie. Campinas, S.P. Editora da Universidade Estadual de Campinas, Brasil, 1999. [ Links ]
[17] L. A. B. San Martin and C. J. C. Negreiros. "Invariant almost Hermitian structures on flag manifolds". Adv. Math. 178 (2003), 277-310. [ Links ]
[18] R.C. de Jesus Silva. Estruturas Quase Hermitianas Invariantes em Espaços Homogêneos de Grupos Semi-simples. Ph. D. tesis, Universidade Estadual de Campinas, Brasil, 2003.. [ Links ]
[19] J. A. Wolf and A. Gray. "Homogeneous spaces defined by Lie group automorphisms, II". J. Diff. Geom. 2:115-159, 1968. [ Links ]
[20] K. Yano. "On a structure defined by a tensor field of type (1,1) satisfying 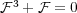 ". Tensor 14:99-109, 1963. [ Links ]
". Tensor 14:99-109, 1963. [ Links ]
Sofía Pinzón
Escuela de Matemáticas,
Universidad Industrial de Santander,
A.A. 678, Bucaramanga, Colombia.
Recibido: 30 de septiembre de 2005
Aceptado: 18 de septiembre de 2006













![∑ r (r + 1)dω(X0, X1, ...,Xr) = (- 1)iXi(ω(X0, X1, ...,Xˆi, ...,Xr)) + i=0 ∑ i+j (- 1) ω([Xi,Xj], X0,...,Xˆi, ...,Xˆj, ...,Xr). 0≤i<j≤r](/img/revistas/ruma/v47n1/1a1089x.png)
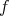 -estructures
-estructures![lN (lX α,lX β) = ɛαɛβɛα+β(ɛα + ɛβ - ɛα+β - ɛαɛ βɛα+β)[Xα, Xβ]; (12) 2 pN (lX α,lX β) = p[F X α,F X β] = ɛαɛβ(ɛα+β - 1)[Xα, Xβ]; (13) N (lX α,lXβ) = F {l(LiepXβF )lX α}; (14) N (pX α,pX β) = lN (pX α,pX β) = (ɛαɛ2 - ɛ2 - ɛ2 + 1)ɛ2 [X α,X β]. (15) β α β α+β](/img/revistas/ruma/v47n1/1a10323x.png)