Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO
Links relacionados
-
 Similares en
SciELO
Similares en
SciELO
Compartir
Revista de la Unión Matemática Argentina
versión impresa ISSN 0041-6932versión On-line ISSN 1669-9637
Rev. Unión Mat. Argent. v.47 n.1 Bahía Blanca ene./jun. 2006
Connections compatible with tensors. A characterization of left-invariant Levi-Civita connections in Lie groups
Paolo Piccione and Daniel V. Tausk
Abstract: Symmetric connections that are compatible with semi-Riemannian metrics can be characterized using an existence result for an integral leaf of a (possibly non integrable) distribution. In this paper we give necessary and sufficient conditions for a left-invariant connection on a Lie group to be the Levi-Civita connection of some semi-Riemannian metric on the group. As a special case, we will consider constant connections in 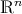 .
.
2000 Mathematics Subject Classification. 53B05, 53C05
In this short note we address the following problem: given a (symmetric) connection  on a smooth manifold
on a smooth manifold 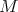 , under which conditions there exists a semi-Riemannian metric
, under which conditions there exists a semi-Riemannian metric 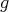 in
in 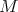 which is
which is  -parallel? This problem can be studied using holonomy theory (see [2]). Alternatively, the problem can be cast in the language of distributions and integral submanifolds, as follows. A connection
-parallel? This problem can be studied using holonomy theory (see [2]). Alternatively, the problem can be cast in the language of distributions and integral submanifolds, as follows. A connection  on a manifold
on a manifold 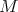 induces naturally a connection in all tensor bundles over
induces naturally a connection in all tensor bundles over 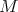 (see for instance [4, § 2.7]), in particular, on the bundle of all (symmetric) (2,0)-tensors on
(see for instance [4, § 2.7]), in particular, on the bundle of all (symmetric) (2,0)-tensors on 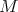 , say,
, say, 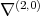 . If
. If 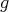 is a
is a 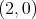 -tensor on
-tensor on 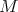 ,
, 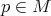 and
and 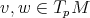 , then the curvature
, then the curvature 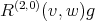 is the bilinear form on
is the bilinear form on 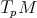 given by:
given by:
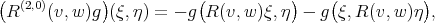 | (1) |
where 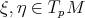 and
and 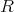 is the curvature tensor of
is the curvature tensor of  . A semi-Riemannian metric is a (globally defined) symmetric nondegenerate
. A semi-Riemannian metric is a (globally defined) symmetric nondegenerate 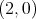 -tensor on
-tensor on 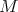 , and compatibility with
, and compatibility with  is equivalent to the property that the section is everywhere tangent to the horizontal distribution determined by the connection
is equivalent to the property that the section is everywhere tangent to the horizontal distribution determined by the connection  . However, such distribution is in general non integrable, namely, integrability of the horizontal distribution is equivalent to the vanishing of the curvature tensor
. However, such distribution is in general non integrable, namely, integrability of the horizontal distribution is equivalent to the vanishing of the curvature tensor 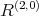 , which is equivalent to the vanishing of
, which is equivalent to the vanishing of 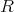 . Hence, the classical Frobenius theorem cannot be employed in this situation. Nevertheless, the existence of simply one integral submanifold of a distribution, or, equivalently, of a parallel section of a vector bundle endowed with a connection, may occur even in the case of non integrable distributions. From (1), one sees immediately that if
. Hence, the classical Frobenius theorem cannot be employed in this situation. Nevertheless, the existence of simply one integral submanifold of a distribution, or, equivalently, of a parallel section of a vector bundle endowed with a connection, may occur even in the case of non integrable distributions. From (1), one sees immediately that if 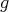 is a
is a 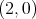 -tensor on
-tensor on 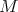 , then the condition that
, then the condition that 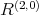 vanishes along
vanishes along 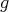 is equivalent to the condition of anti-symmetry of
is equivalent to the condition of anti-symmetry of 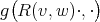 , for all
, for all 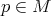 and all
and all 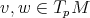 .
.
Let us consider the case that an open neighborhood 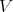 of a point
of a point 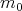 of a manifold
of a manifold 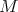 is ruled by a family of curves issuing from
is ruled by a family of curves issuing from 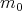 , parameterized by points of some manifold
, parameterized by points of some manifold 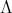 . What this means is that it is given a smooth function
. What this means is that it is given a smooth function 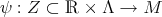 , defined on an open subset
, defined on an open subset 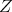 of
of  , with
, with 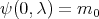 for all
for all 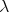 , and that admits a smooth right inverse
, and that admits a smooth right inverse 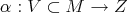 . Assume that it is given a nondegenerate symmetric bilinear form
. Assume that it is given a nondegenerate symmetric bilinear form 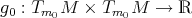 ; one obtains a semi-Riemannian metric on
; one obtains a semi-Riemannian metric on 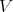 by spreading
by spreading 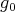 with parallel transport along the curves
with parallel transport along the curves 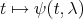 . If the tensor
. If the tensor 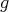 obtained in this way is such that
obtained in this way is such that 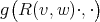 is an antisymmetric bilinear form on
is an antisymmetric bilinear form on 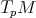 for all
for all 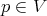 and all
and all 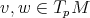 , then
, then 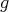 is
is  -parallel. The precise statement of this fact is the following:
-parallel. The precise statement of this fact is the following:
Proposition 1.1. Let 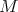 be a smooth manifold,
be a smooth manifold,  be a symmetric connection on
be a symmetric connection on 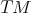 ,
, 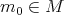 and
and  be a nondegenerate symmetric bilinear form on
be a nondegenerate symmetric bilinear form on 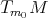 . Let
. Let 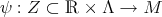 be a
be a 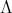 -parametric family of curves on
-parametric family of curves on 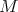 with a local right inverse
with a local right inverse 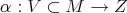 ; assume that
; assume that 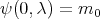 , for all
, for all  . For each
. For each 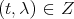 , we denote by
, we denote by  the parallel transport along
the parallel transport along 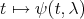 . Assume that for all
. Assume that for all  the linear operator:
the linear operator:
![-1 [ ] P (t,λ) R ψ(t,λ)(v,w ) P(t,λ) : Tm0M -→ Tm0M](/img/revistas/ruma/v47n1/1a1274x.png) | (2) |
is anti-symmetric with respect to 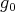 , for all
, for all 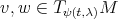 , where
, where

denotes the linear operator corresponding to the curvature tensor of  . Then
. Then  is the Levi-Civita connection of the semi-Riemannian metric
is the Levi-Civita connection of the semi-Riemannian metric 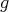 on
on 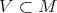 defined by setting:
defined by setting:

for all 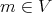 .
.
Proof. See [3] □
In the real analytic case, we have the following global result:
Proposition 1.2. Let 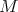 be a simply-connected real-analytic manifold and let
be a simply-connected real-analytic manifold and let  be a real-analytic symmetric connection on
be a real-analytic symmetric connection on 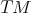 . If there exists a semi-Riemannian metric
. If there exists a semi-Riemannian metric 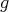 on a nonempty open connected subset of
on a nonempty open connected subset of 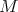 having
having  as its Levi-Civita connection then
as its Levi-Civita connection then 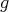 extends to a globally defined semi-Riemannian metric on
extends to a globally defined semi-Riemannian metric on 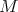 having
having  as its Levi-Civita connection.□
as its Levi-Civita connection.□
The two results above will be used in Sections 3, 4 and 5 to characterize symmetric connections in Lie groups that are constant in left invariant referentials. The case of 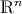 (Lemma 3.1 and Proposition 3.2), and more specifically the
(Lemma 3.1 and Proposition 3.2), and more specifically the 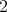 -dimensional case (Proposition 4.9), will be studied with some more detail.
-dimensional case (Proposition 4.9), will be studied with some more detail.
It is an interesting problem to study conditions for the existence, uniqueness, multiplicity, etc., of (symmetric) connections that are compatible with arbitrarily given tensors. It is well known that semi-Riemannian metrics admit exactly one symmetric and compatible connection, called the Levi-Civita connection of the metric. Uniqueness can be deduced also by a curious combinatorial argument, see Corollary 2.2. The next interesting case is that of symplectic forms, in which case one has existence, but not uniqueness. We will start the paper with a short section containing a couple of simple results concerning compatible connections. First, we will show the combinatorial argument that shows the uniqueness of the Levi-Civita connection of a semi-Riemannian metric tensor (Corollary 2.2). Second, we will prove that the existence of a symmetric connection compatible with a nondegenerate two-form 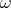 is equivalent to the fact that
is equivalent to the fact that 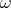 is closed, in which case there are infinitely many symmetric connections compatible with
is closed, in which case there are infinitely many symmetric connections compatible with 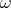 (Lemma 2.3).
(Lemma 2.3).
2. Connections compatible with tensors
Let 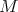 be a smooth manifold and let
be a smooth manifold and let  be any tensor in
be any tensor in 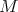 ; we will be mostly interested in the case when
; we will be mostly interested in the case when  is a semi-Riemannian metric tensor on
is a semi-Riemannian metric tensor on 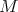 (i.e.,
(i.e.,  is a nondegenerate symmetric
is a nondegenerate symmetric 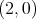 -tensor), or when
-tensor), or when 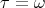 is a symplectic form on
is a symplectic form on 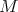 (i.e.,
(i.e.,  is a nondegenerate closed
is a nondegenerate closed 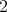 -form). If
-form). If  is a connection in
is a connection in 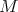 , i.e., a connection on the tangent bundle
, i.e., a connection on the tangent bundle 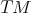 , then we have naturally induced connections on all tensor bundles on
, then we have naturally induced connections on all tensor bundles on 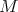 , all of which will be denoted by the same symbol
, all of which will be denoted by the same symbol  .
.
The torsion of  is the anti-symmetric tensor
is the anti-symmetric tensor
![T (X, Y) = ∇X Y - ∇Y X - [X, Y ],](/img/revistas/ruma/v47n1/1a12115x.png)
where ![[X, Y ]](/img/revistas/ruma/v47n1/1a12116x.png) denotes the Lie brackets of the vector fields
denotes the Lie brackets of the vector fields  and
and 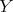 ;
;  is called symmetric if
is called symmetric if  . The connection
. The connection  is said to be compatible with
is said to be compatible with  if
if  is
is  -parallel, i.e., when
-parallel, i.e., when  .
.
Establishing whether a given tensor  admits compatible connections is a local problem. Namely, one can use partition of unity to extend locally defined connections and observe that a convex combination of compatible connections is a compatible connection. In local coordinates, finding a connection compatible with a given tensor reduces to determining the existence of solutions for a non homogeneous linear system for the Christoffel symbols of the connection.
admits compatible connections is a local problem. Namely, one can use partition of unity to extend locally defined connections and observe that a convex combination of compatible connections is a compatible connection. In local coordinates, finding a connection compatible with a given tensor reduces to determining the existence of solutions for a non homogeneous linear system for the Christoffel symbols of the connection.
It is well known that semi-Riemannian metric tensors admit a unique compatible symmetric connection, called the Levi-Civita connection of the metric tensor, which can be given explicitly by Koszul formula (see for instance [1]). Uniqueness of the Levi-Civita connection can be obtained by a curious combinatorial argument, as follows.
Suppose that  and
and 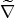 are connections on
are connections on 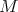 ; their difference
; their difference  is a tensor, that will be denoted by
is a tensor, that will be denoted by 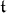 :
:
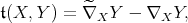
where 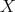 and
and  are smooth vector fields on
are smooth vector fields on 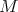 . If both
. If both  and
and 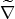 are symmetric connections, then
are symmetric connections, then 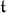 is symmetric:
is symmetric:
![^ ^ 𝔱(X, Y ) - 𝔱(Y,X ) = ∇X Y - ∇X Y - ∇Y X + ∇Y X = [X, Y ] + [Y, X ] = 0.](/img/revistas/ruma/v47n1/1a12139x.png)
Lemma 2.1. Let 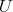 be a set and
be a set and 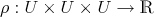 be a map that is symmetric in its first two variables and anti-symmetric in its last two variables. Then
be a map that is symmetric in its first two variables and anti-symmetric in its last two variables. Then 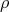 is identically zero.
is identically zero.
Proof. Let 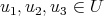 be fixed. We have:
be fixed. We have:

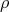 is anti-symmetric in the first and the third variables. On the other hand:
is anti-symmetric in the first and the third variables. On the other hand: 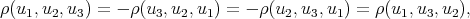
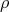 is symmetric in the second and the third variables. This concludes the proof. □
is symmetric in the second and the third variables. This concludes the proof. □ Corollary 2.2. There exists at most one symmetric connection which is compatible with a semi-Riemannian metric.
Proof. Assume that  is a semi-Riemannian metric on
is a semi-Riemannian metric on 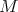 , and let
, and let  and
and 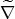 two symmetric connections such that
two symmetric connections such that 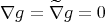 ; for all
; for all 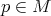 consider the map
consider the map 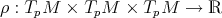 given by:
given by:
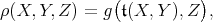
where 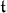 is the difference
is the difference  . Since
. Since  is symmetric, then
is symmetric, then 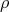 is symmetric in the first two variables. On the other hand,
is symmetric in the first two variables. On the other hand, 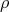 is anti-symmetric in the last two variables:
is anti-symmetric in the last two variables:
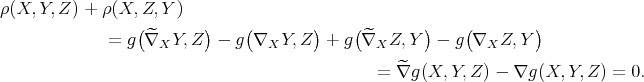 |
By Lemma 2.1,  , hence
, hence  , and thus
, and thus 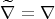 . □
. □
For symplectic forms, the situation changes radically. Among all nondegenerate two-forms, the existence of a symmetric compatible connection characterizes the symplectic ones:
Lemma 2.3. Let 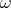 be a nondegenerate
be a nondegenerate  -form on a (necessarily even dimensional) manifold
-form on a (necessarily even dimensional) manifold 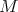 . There exists a symmetric connection in
. There exists a symmetric connection in 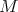 compatible with
compatible with 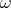 if and only if
if and only if 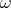 is closed. In this case, there are infinitely many symmetric connections that are compatible with
is closed. In this case, there are infinitely many symmetric connections that are compatible with  .
.
Proof. If 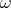 is closed, i.e., if
is closed, i.e., if  is a symplectic form on
is a symplectic form on 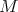 , Darboux theorem tells us that one can find coordinates
, Darboux theorem tells us that one can find coordinates 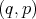 around every point of
around every point of 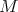 such that
such that 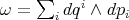 , which means that
, which means that 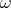 is constant in such coordinate system. The (locally defined) symmetric connection which has vanishing Christoffel symbols in such coordinates is clearly compatible with
is constant in such coordinate system. The (locally defined) symmetric connection which has vanishing Christoffel symbols in such coordinates is clearly compatible with 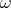 . As observed above, using partitions of unity one can find a globally defined symmetric connection compatible with
. As observed above, using partitions of unity one can find a globally defined symmetric connection compatible with 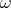 .
.
Conversely, if  is any symmetric connection in
is any symmetric connection in 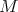 , then
, then 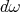 is given by
is given by 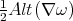 , where
, where 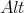 denotes the alternator; in particular, if there exists a compatible symmetric connection it must be
denotes the alternator; in particular, if there exists a compatible symmetric connection it must be 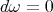 . □
. □
Let 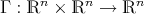 be a symmetric bilinear map and consider the symmetric connection
be a symmetric bilinear map and consider the symmetric connection  on
on  defined by:
defined by:
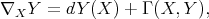 | (3) |
for any smooth vector fields 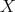 ,
, 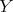 on
on  . We now apply the result of Proposition 1.1 to determine when
. We now apply the result of Proposition 1.1 to determine when  is the Levi-Civita connection of a semi-Riemannian metric on
is the Levi-Civita connection of a semi-Riemannian metric on 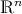 . Given
. Given 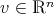 then the parallel transport
then the parallel transport 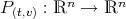 along the curve
along the curve 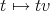 is given by:
is given by:
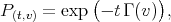
where we identify 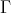 with the linear map
with the linear map 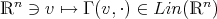 . For any
. For any 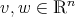 , the curvature tensor
, the curvature tensor 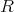 of
of  is given by:
is given by:
![n Rx (v,w) = Γ (v)Γ (w ) - Γ (w )Γ (v) = [Γ (v),Γ (w)] ∈ Lin (ℝ ),](/img/revistas/ruma/v47n1/1a12206x.png)
for all  . Applying Proposition 1.1 to the
. Applying Proposition 1.1 to the 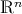 -parametric family of curves
-parametric family of curves 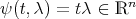 with right inverse
with right inverse 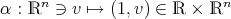 we obtain the following:
we obtain the following:
Lemma 3.1. Let 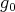 be a nondegenerate symmetric bilinear form on
be a nondegenerate symmetric bilinear form on 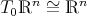 . Then
. Then 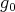 extends to a semi-Riemannian metric
extends to a semi-Riemannian metric 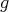 on
on 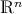 having (3) as its Levi-Civita connection if and only if the linear operator:
having (3) as its Levi-Civita connection if and only if the linear operator:
![( ) ( ) exp Γ (v) [Γ (w1),Γ (w2 )]exp - Γ (v) : ℝn → ℝn](/img/revistas/ruma/v47n1/1a12216x.png)
is anti-symmetric with respect to 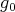 , for all
, for all  .□
.□
Given a nondegenerate symmetric bilinear form 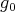 on
on 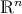 we denote by
we denote by 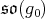 the Lie algebra of all
the Lie algebra of all 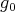 -anti-symmetric endomorphisms of
-anti-symmetric endomorphisms of 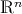 . Given a linear endomorphism
. Given a linear endomorphism 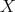 of
of 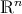 we write:
we write:
![adX (Y ) = [X, Y ] = XY - Y X,](/img/revistas/ruma/v47n1/1a12226x.png)
for all 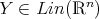 .
.
Proposition 3.2. Let 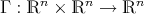 be a symmetric bilinear map and let
be a symmetric bilinear map and let 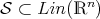 be the range of the linear map
be the range of the linear map 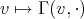 . A nondegenerate symmetric bilinear form
. A nondegenerate symmetric bilinear form  on
on 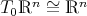 extends to a semi-Riemannian metric
extends to a semi-Riemannian metric 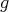 on
on 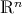 having (3) as its Levi-Civita connection if and only if:
having (3) as its Levi-Civita connection if and only if:
![(adX )k[Y,Z ] ∈ 𝔰𝔬(g0),](/img/revistas/ruma/v47n1/1a12235x.png)
for all 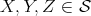 and all
and all  .
.
Proof. By Lemma 3.1, 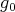 extends to a semi-Riemannian metric
extends to a semi-Riemannian metric 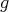 on
on  having (3) as its Levi-Civita connection if and only if:
having (3) as its Levi-Civita connection if and only if:
![exp(tX )[Y, Z]exp (- tX ) ∈ 𝔰𝔬(g0),](/img/revistas/ruma/v47n1/1a12241x.png)
for all 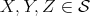 and all
and all 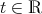 . The conclusion follows by observing that:
. The conclusion follows by observing that:
![+ ∞ ∑ tk k exp(tX )[Y, Z]exp (- tX ) = k!(adX ) [Y, Z]. □ k=0](/img/revistas/ruma/v47n1/1a12244x.png)
Corollary 3.3. Let 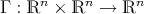 be a symmetric bilinear map and let
be a symmetric bilinear map and let 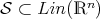 be the range of the linear map
be the range of the linear map 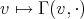 . Denote by
. Denote by 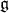 the Lie algebra spanned by
the Lie algebra spanned by  and by
and by ![𝔤 ′ = [𝔤,𝔤]](/img/revistas/ruma/v47n1/1a12250x.png) the commutator subalgebra of
the commutator subalgebra of 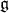 . If
. If 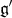 is contained in
is contained in 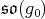 for some nondegenerate symmetric bilinear form
for some nondegenerate symmetric bilinear form 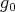 on
on 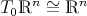 then
then 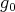 extends to a semi-Riemannian metric
extends to a semi-Riemannian metric 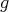 on
on 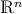 having (3) as its Levi-Civita connection.□
having (3) as its Levi-Civita connection.□
If  , the Lie algebra
, the Lie algebra 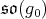 is one-dimensional. This observation allows us to show that, for
is one-dimensional. This observation allows us to show that, for  , the condition
, the condition 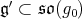 in the statement of Corollary 3.3 is also necessary for
in the statement of Corollary 3.3 is also necessary for 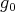 to extend to a semi-Riemannian metric
to extend to a semi-Riemannian metric 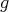 on
on 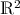 having (3) as its Levi-Civita connection.
having (3) as its Levi-Civita connection.
Lemma 4.1. Let 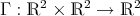 be a symmetric bilinear map and let
be a symmetric bilinear map and let  be the range of the linear map
be the range of the linear map 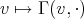 . Denote by
. Denote by 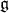 the Lie algebra spanned by
the Lie algebra spanned by 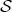 . Then a nondegenerate symmetric bilinear form
. Then a nondegenerate symmetric bilinear form 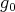 on
on  extends to a semi-Riemannian metric
extends to a semi-Riemannian metric 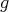 on
on 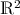 having (3) as its Levi-Civita connection if and only if
having (3) as its Levi-Civita connection if and only if 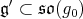 .
.
Proof. Define a sequence 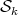 of subspaces of
of subspaces of 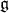 inductively by setting
inductively by setting 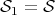 and by taking
and by taking ![Sk+1 = [S, Sk]](/img/revistas/ruma/v47n1/1a12279x.png) to be the linear span of all commutators
to be the linear span of all commutators ![[X, Y ]](/img/revistas/ruma/v47n1/1a12280x.png) , with
, with 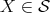 ,
, 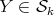 . Using the Jacobi identity it is easy to show that
. Using the Jacobi identity it is easy to show that ![[Sk, Sl] ⊂ Sk+l](/img/revistas/ruma/v47n1/1a12283x.png) and therefore:
and therefore:
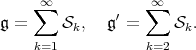
By Proposition 3.2, if extends to a semi-Riemannian metric 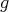 on
on 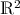 having (3) as its Levi-Civita connection then
having (3) as its Levi-Civita connection then  and
and  are contained in
are contained in 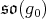 . Since
. Since 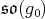 is one dimensional, we have either
is one dimensional, we have either 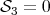 or
or  ; in the first case,
; in the first case,  for all
for all  and in the latter case
and in the latter case  for all
for all  . In any case,
. In any case,  and the conclusion follows. □
and the conclusion follows. □
Lemma 4.2. Let 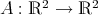 be a nonzero linear map. There exists a nondegenerate symmetric bilinear form
be a nonzero linear map. There exists a nondegenerate symmetric bilinear form 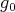 on
on  with
with 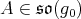 if and only if
if and only if 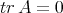 and
and 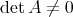 ; moreover,
; moreover, 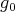 is positive definite (resp., has index
is positive definite (resp., has index  ) if and only if
) if and only if 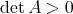 (resp.,
(resp., 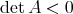 ).
).
Proof. Assume that 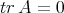 and
and 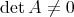 . Write
. Write  , with
, with 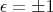 and
and 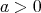 . It is easy to see that
. It is easy to see that 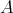 is represented by the matrix
is represented by the matrix 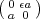 in some basis
in some basis 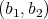 of
of 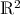 . We define
. We define 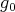 by setting:
by setting:
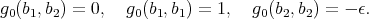 | (4) |
Conversely, if 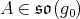 for some
for some 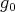 then we can choose a basis
then we can choose a basis 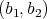 of
of 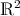 such that (4) holds and the matrix of
such that (4) holds and the matrix of 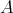 on such basis is of the form
on such basis is of the form 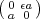 . □
. □
Corollary 4.3. Let 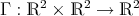 be a symmetric bilinear map and let
be a symmetric bilinear map and let 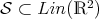 be the range of the linear map
be the range of the linear map 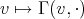 . Denote by
. Denote by 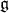 the Lie algebra spanned by
the Lie algebra spanned by 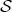 . There exists a semi-Riemannian metric on
. There exists a semi-Riemannian metric on 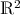 having (3) as its Levi-Civita connection if and only if either
having (3) as its Levi-Civita connection if and only if either 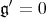 or
or 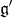 is one-dimensional and it is spanned by an invertible
is one-dimensional and it is spanned by an invertible  matrix.
matrix.
Proof. Follows from Lemmas 4.1 and 4.2, observing that the elements of 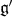 have null trace. □
have null trace. □
Lemma 4.4. Let 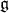 be a three-dimensional real Lie algebra with
be a three-dimensional real Lie algebra with 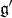 one-dimensional. Then the center
one-dimensional. Then the center 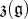 of
of 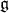 is one-dimensional.
is one-dimensional.
Proof. Let 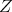 denote a generator of
denote a generator of 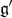 , so that
, so that ![[X, Y ] = α(X, Y )Z](/img/revistas/ruma/v47n1/1a12341x.png) , for all
, for all 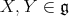 , where
, where  is an antisymmetric bilinear form on
is an antisymmetric bilinear form on 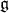 ; clearly, the kernel of
; clearly, the kernel of 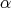 is the center of
is the center of 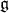 . Since
. Since 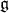 is three-dimensional, the kernel of
is three-dimensional, the kernel of 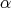 is either
is either 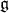 or it is one-dimensional; the first possibility does not occur, since
or it is one-dimensional; the first possibility does not occur, since  is nonzero. □
is nonzero. □
Corollary 4.5. Let 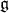 be a three-dimensional real Lie algebra with
be a three-dimensional real Lie algebra with 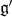 one-dimensional. Then there exists a basis
one-dimensional. Then there exists a basis 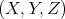 of
of 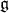 such that one the following commutation relations holds:
such that one the following commutation relations holds:
Proof. Choose a basis 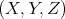 of
of 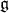 with
with 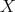 in
in 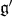 . If
. If 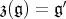 then
then ![[Y,Z ] ⁄= 0](/img/revistas/ruma/v47n1/1a12364x.png) , otherwise
, otherwise  ; thus, we can replace
; thus, we can replace 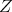 with a scalar multiple of
with a scalar multiple of 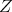 so that
so that ![[Y,Z ] = X](/img/revistas/ruma/v47n1/1a12368x.png) and relations (1) hold. If
and relations (1) hold. If 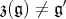 , we may assume that
, we may assume that 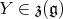 and
and ![[Z,X ] ⁄= 0](/img/revistas/ruma/v47n1/1a12371x.png) ; again, replacing
; again, replacing 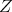 with a scalar multiple of
with a scalar multiple of 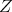 gives
gives ![[Z,X ] = X](/img/revistas/ruma/v47n1/1a12374x.png) and relations (2) hold. □
and relations (2) hold. □
In what follows we denote by 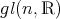 the Lie algebra of linear endomorphisms of
the Lie algebra of linear endomorphisms of 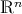 .
.
Lemma 4.6. Let 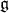 be a three-dimensional Lie subalgebra of
be a three-dimensional Lie subalgebra of 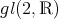 with
with 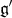 one-dimensional. There exists a basis
one-dimensional. There exists a basis 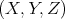 of
of 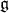 with
with 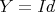 and
and ![[Z, X ] = X](/img/revistas/ruma/v47n1/1a12383x.png) .
.
Proof. We show that 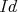 is in
is in 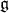 . Assume not. By Lemma 4.4, there exists a nonzero element
. Assume not. By Lemma 4.4, there exists a nonzero element 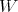 in
in 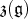 . Then
. Then 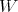 commutes with
commutes with 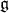 and with
and with 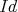 , which implies that
, which implies that 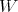 is in the center of
is in the center of 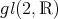 ; thus
; thus 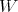 is a nonzero multiple of
is a nonzero multiple of 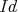 , contradicting our assumption.
, contradicting our assumption.
Now  implies that
implies that 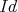 spans
spans 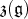 ; thus possibility (1) in the statement of Corollary 4.5 does not occur for it would imply that
; thus possibility (1) in the statement of Corollary 4.5 does not occur for it would imply that ![[Y, Z]](/img/revistas/ruma/v47n1/1a12398x.png) is a nonzero multiple of the identity. Hence possibility (2) occurs and we can assume that
is a nonzero multiple of the identity. Hence possibility (2) occurs and we can assume that 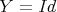 . □
. □
Lemma 4.7. If 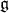 is a two-dimensional real Lie algebra with
is a two-dimensional real Lie algebra with 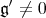 then there exists a basis
then there exists a basis 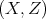 of
of 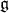 with
with ![[Z, X ] = X](/img/revistas/ruma/v47n1/1a12404x.png) .
.
Proof. Let 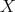 be a nonzero element in
be a nonzero element in 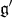 ; clearly,
; clearly, 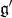 is one-dimensional. We can choose
is one-dimensional. We can choose 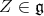 ,
, 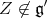 , with
, with ![[Z,X ] = X](/img/revistas/ruma/v47n1/1a12410x.png) . □
. □
Lemma 4.8. If 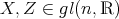 and
and ![[Z, X ] = X](/img/revistas/ruma/v47n1/1a12412x.png) then
then 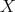 is not invertible.
is not invertible.
Proof. If  were invertible then
were invertible then ![[Z,X ] = X](/img/revistas/ruma/v47n1/1a12415x.png) would imply
would imply 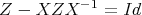 . A contradiction is obtained by taking traces on both sides. □
. A contradiction is obtained by taking traces on both sides. □
Proposition 4.9. Let 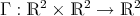 be a symmetric bilinear map and let
be a symmetric bilinear map and let 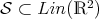 be the range of the linear map
be the range of the linear map 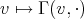 . Then there exists a semi-Riemannian metric
. Then there exists a semi-Riemannian metric 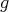 on
on 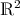 having (3) as its Levi-Civita connection if and only if
having (3) as its Levi-Civita connection if and only if ![[X, Y ] = 0](/img/revistas/ruma/v47n1/1a12422x.png) , for all
, for all 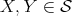 . In this case, a semi-Riemannian metric
. In this case, a semi-Riemannian metric 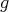 on
on 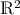 having (3) as its Levi-Civita connection can be chosen with an arbitrary value
having (3) as its Levi-Civita connection can be chosen with an arbitrary value 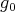 at the origin.
at the origin.
Proof. If ![[X,Y ] = 0](/img/revistas/ruma/v47n1/1a12427x.png) for all
for all 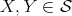 then, by Lemma 4.1, any nondegenerate symmetric bilinear form
then, by Lemma 4.1, any nondegenerate symmetric bilinear form  on
on 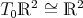 extends to semi-Riemannian metric
extends to semi-Riemannian metric 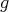 on
on 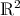 having (3) as its Levi-Civita connection. Now assume that there exists a semi-Riemannian metric on
having (3) as its Levi-Civita connection. Now assume that there exists a semi-Riemannian metric on  having (3) as its Levi-Civita connection and denote by
having (3) as its Levi-Civita connection and denote by 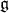 the Lie algebra spanned by
the Lie algebra spanned by 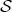 . By Corollary 4.3, either
. By Corollary 4.3, either 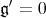 or
or 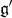 is one-dimensional and it is spanned by an invertible
is one-dimensional and it is spanned by an invertible 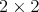 matrix. Let us show that the second possibility cannot occur. If
matrix. Let us show that the second possibility cannot occur. If 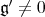 then
then 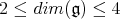 . If
. If 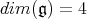 then
then 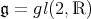 and
and  is three-dimensional, which is not possible. If either
is three-dimensional, which is not possible. If either 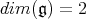 or
or 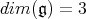 then by Lemmas 4.6 and 4.7 there exist
then by Lemmas 4.6 and 4.7 there exist 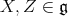 with
with ![[Z, X ] = X](/img/revistas/ruma/v47n1/1a12447x.png) and such that
and such that 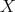 spans
spans 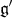 . By Lemma 4.8,
. By Lemma 4.8, 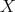 is not invertible and we obtain a contradiction. □
is not invertible and we obtain a contradiction. □
5. Left-Invariant Connections on Lie Groups
Let 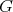 be a Lie group and
be a Lie group and  be a left-invariant connection on
be a left-invariant connection on  . The connection
. The connection  is determined by a bilinear map
is determined by a bilinear map 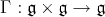 , i.e.:
, i.e.:
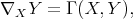
for any left-invariant vector fields 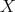 ,
, 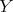 on
on 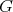 .
.
The torsion of  is given by:
is given by:
![T(X, Y ) = Γ (X, Y ) - Γ (Y,X ) - [X,Y ].](/img/revistas/ruma/v47n1/1a12461x.png)
Observe that  is torsion-free if and only if there exists a symmetric bilinear map
is torsion-free if and only if there exists a symmetric bilinear map 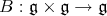 with
with ![Γ (X, Y ) = B (X, Y) + 1[X, Y ] 2](/img/revistas/ruma/v47n1/1a12464x.png) , for all
, for all 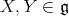 . If we identify
. If we identify 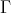 with the linear map
with the linear map 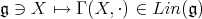 then
then  is torsion-free if and only if
is torsion-free if and only if 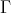 is a Lie algebra homomorphism. The curvature tensor of
is a Lie algebra homomorphism. The curvature tensor of  is given by:
is given by:
![( ) R (X,Y ) = [Γ (X ),Γ (Y )] - Γ [X, Y ] ;](/img/revistas/ruma/v47n1/1a12471x.png)
observe that the first bracket is the commutator in 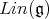 and the second is the Lie algebra product of
and the second is the Lie algebra product of 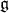 .
.
Given a curve 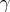 on
on 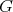 , we identify vector fields along
, we identify vector fields along 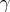 with curves on
with curves on 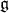 by left translation. Using this identification, the parallel transport of
by left translation. Using this identification, the parallel transport of 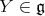 along a one-parameter subgroup
along a one-parameter subgroup 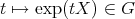 is given by
is given by 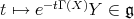 .
.
Proposition 5.1. Assume that  is torsion-free and let
is torsion-free and let 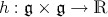 be a nondegenerate symmetric bilinear form. The following condition is necessary and sufficient for the existence of an extension of
be a nondegenerate symmetric bilinear form. The following condition is necessary and sufficient for the existence of an extension of 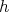 to a semi-Riemannian metric on a neighborhood of the identity of
to a semi-Riemannian metric on a neighborhood of the identity of 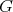 whose Levi-Civita connection is
whose Levi-Civita connection is  :
:
![Γ (Z)( ( )) - Γ (Z ) e [Γ (X ),Γ (Y)] - Γ [X, Y] e ∈ 𝔰𝔬(h), for all X, Y, Z ∈ 𝔤.](/img/revistas/ruma/v47n1/1a12486x.png) | (5) |
In (5) we have denoted by  the Lie subalgebra of
the Lie subalgebra of 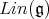 consisting of
consisting of 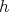 -anti-symmetric linear operators.
-anti-symmetric linear operators.
Proof. Set 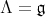 and consider the one-parameter family of curves
and consider the one-parameter family of curves 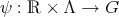 defined by
defined by 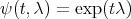 . If
. If 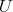 is an open neighborhood of the origin of
is an open neighborhood of the origin of 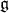 that is mapped diffeomorphically by
that is mapped diffeomorphically by 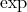 onto an open neighborhood
onto an open neighborhood  of the identity of
of the identity of 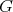 then a local right inverse for
then a local right inverse for 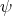 can be defined by setting
can be defined by setting 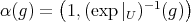 , for all
, for all  . The conclusion follows from Proposition 1.1. □
. The conclusion follows from Proposition 1.1. □
Corollary 5.2. Assume that  is torsion-free and let
is torsion-free and let 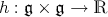 be a nondegenerate symmetric bilinear form. If
be a nondegenerate symmetric bilinear form. If 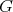 is (connected and) simply-connected then condition (5) is necessary and sufficient for the existence of a globally defined semi-Riemannian metric on
is (connected and) simply-connected then condition (5) is necessary and sufficient for the existence of a globally defined semi-Riemannian metric on 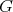 whose Levi-Civita connection is
whose Levi-Civita connection is  .
.
Proof. It follows from Proposition 5.1 and from Proposition 1.2 observing that left-invariant objects on a Lie group are always real-analytic. □
Lemma 5.3. Condition (5) is equivalent to:
![n ( ( )) ad Γ (Z) [Γ (X ),Γ (Y)] - Γ [X, Y] ∈ 𝔰𝔬(h ), for all X,Y, Z ∈ 𝔤, n ≥ 0,](/img/revistas/ruma/v47n1/1a12506x.png)
where ![adA (B ) = [A, B ]](/img/revistas/ruma/v47n1/1a12507x.png) , for all
, for all  .
.
Proof. Replace 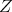 by
by  in (5) and compute the Taylor expansion in powers of
in (5) and compute the Taylor expansion in powers of 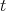 of the corresponding expression. □
of the corresponding expression. □
[1] M. P. do Carmo, Riemannian Geometry, Birkhäuser, Boston, 1992. [ Links ]
[2] S. Kobayashi and K. Nomizu, Foundations of differential geometry. Vol I, Interscience Publishers, New York-London, 1963. [ Links ]
[3] P. Piccione, D. V. Tausk, The single-leaf Frobenius theorem with applications, preprint 2005, to appear in Resenhas do Instituto de Matemática e Estatística da Universidade de São Paulo. [ Links ]
[4] P. Piccione, D. V. Tausk, The theory of connections and G-structures: applications to affine and isometric immersions, XV Escola de Geometria Diferencial, Publicações do IMPA, Rio de Janeiro, 2006, ISBN 85-244-0248-2. [ Links ]
Paolo Piccione
Departamento de Matemática,
Universidade de São Paulo,
São Paulo, Brazil.
piccione@ime.usp.br
http://www.ime.usp.br/˜piccione
Daniel V. Tausk
Departamento de Matemática,
Universidade de São Paulo,
São Paulo, Brazil.
tausk@ime.usp.br
http://www.ime.usp.br/˜tausk
Recibido: 27 de septiembre de 2005
Aceptado: 29 de agosto de 2006













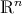
![[X, Y ] = [X, Z] = 0](/img/revistas/ruma/v47n1/1a12355x.png) ,
, ![[Y,Z ] = X](/img/revistas/ruma/v47n1/1a12356x.png) ;
;![[X, Y ] = [Y,Z ] = 0](/img/revistas/ruma/v47n1/1a12357x.png) ,
, ![[Z, X ] = X](/img/revistas/ruma/v47n1/1a12358x.png) .
.
