Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO
Related links
-
 Similars in
SciELO
Similars in
SciELO
Share
Revista de la Unión Matemática Argentina
Print version ISSN 0041-6932On-line version ISSN 1669-9637
Rev. Unión Mat. Argent. vol.47 no.2 Bahía Blanca July/Dec. 2006
Hypersurfaces with constant mean curvature
Susana Fornari
Resume of the Conference presented in the II Encuentro de Geometría Diferencial La Falda, Sierras de Córdoba, Argentina, June 6-11, 2005.
Abstract: The hypersurfaces with constant mean curvature (cmc) are studied under different aspects:
1- As critical Points of a Variational Problem.
2- As solutions of a Dirichlet Problem.
3- Under the point of view of harmonicity of the Gauss map.
We explain, in a short wave, the principal technical and some results obtained in each aspects.
1. Hypersurfaces of cmc as critical points of a Variational Problem.
An 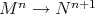 isometric immersion with constant mean curvature is a critical point of the area functional for variations preserving volume.
isometric immersion with constant mean curvature is a critical point of the area functional for variations preserving volume.
When the critical point is a local minimum, 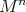 is called stable. In particular, the stable hypersurfaces, when compact, bound isoparametric domains (frontier with area minima for a given fixed volume).
is called stable. In particular, the stable hypersurfaces, when compact, bound isoparametric domains (frontier with area minima for a given fixed volume).
In 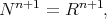
 or
or 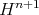 the unique compact and stable hypersurfaces with cmc. are the geodesic spheres [B-dC-E, 1984], [Heintze, 1988].
the unique compact and stable hypersurfaces with cmc. are the geodesic spheres [B-dC-E, 1984], [Heintze, 1988].
In 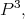 the real projective space of dim 3, the compact stable surfaces are embedded, have genus 0 or 1, geodesic sphere or flat torus, respectively. Ritoré-Ros, 1992, [R-R]. In this paper, the authors give the isoperimetrical profile of these surfaces.
the real projective space of dim 3, the compact stable surfaces are embedded, have genus 0 or 1, geodesic sphere or flat torus, respectively. Ritoré-Ros, 1992, [R-R]. In this paper, the authors give the isoperimetrical profile of these surfaces.
In the torus  the stable compact surfaces
the stable compact surfaces 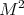 immersed with cmc are not classified. Partial results are known (see [F-R1] and references):
immersed with cmc are not classified. Partial results are known (see [F-R1] and references):
- the genus 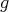 of
of 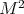 must be
must be 
- if  or
or 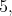 then
then 
- if 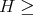 some constant
some constant 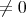 (depending on the radius of the Torus) then the stable surface with cmc
(depending on the radius of the Torus) then the stable surface with cmc 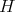 is embedded and
is embedded and  or
or 
- It is known a stable surface with genus 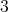 and
and 
Here 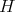 denotes the mean curvature of
denotes the mean curvature of 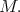
The stable non compact hypersurface with cmc. are also studied. In 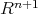 they are hyperplanes
they are hyperplanes In
In 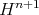 and
and 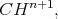 the horospheres and tubes with sufficiently large radius around totally geodesic cod 2 -submanifolds are cmc stable hypersurfaces.
the horospheres and tubes with sufficiently large radius around totally geodesic cod 2 -submanifolds are cmc stable hypersurfaces.
2. Constant Mean Curvature hypersurfaces as Solutions of a Dirichlet Problem.
It is known that if 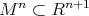 is the graph of a differentiable function
is the graph of a differentiable function 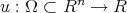
 then
then
|
|
where  denotes the gradient in
denotes the gradient in 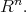
Therefore, the existence of a graph with constant mean curvature is equivalent to assure the existence of the solution of a Dirichlet problem:
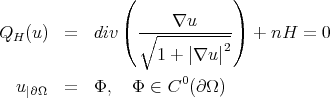
In this case, 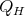 is a quasi linear elliptic operator of second order and it satisfies the Maximum Principle.
is a quasi linear elliptic operator of second order and it satisfies the Maximum Principle.
For limited domains, with appropriate conditions in the mean curvature of the boundary, the Dirichlet problem above has a unique solution (Serrin, 1969) [S].
For not limited domains 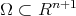 the existence of solutions depends on the construction of appropriate barriers (Perron's method).
the existence of solutions depends on the construction of appropriate barriers (Perron's method).
In  and
and 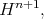 it is possible to define the "graph" of a real function whose domain is a region
it is possible to define the "graph" of a real function whose domain is a region 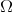 contained in a totally geodesic submanifold of dim
contained in a totally geodesic submanifold of dim 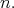 The Dirichlet problem is similar to the above problem, but the operator
The Dirichlet problem is similar to the above problem, but the operator 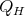 can be quite complicated.
can be quite complicated.
These graphs have been recently studied, some references are: Guio- Sá Earp, 2005 [G-S], Fornari, Lira, Ripoll, 2002 [F,L,R], Dajczer -Ripoll, preprint [D-R], Alias-Dajczer, preprint [A-D].
3. Constant Mean Curvature hypersurface and harmonicity of the Gauss map.
Given a complete surface 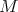 in
in 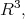 it is well known that:
it is well known that:
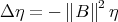
where 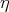 is the unit normal to a surface,
is the unit normal to a surface,  ,
,  is the Laplacian of
is the Laplacian of 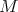 and
and  is the norm of the second fundamental of
is the norm of the second fundamental of  .
.
The above equation has been used to obtain many important results, one of them, due to Hoffman, Osserman and Shoen, 1982, [H-O-S] said:
"If 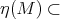 closed hemisphere of
closed hemisphere of 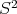 then
then 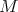 is a plane or a right circular cylinder"
is a plane or a right circular cylinder"
or equivalently
"If the function 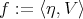 does not change sign on
does not change sign on 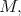 for same fixed vector
for same fixed vector 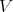
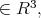 then
then 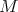 is invariant by a one parameter subgroup of translations of
is invariant by a one parameter subgroup of translations of 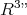
In a joint work with Espirito-Santo, Frensel and Ripoll, [ES-F-F-R], 2003, we obtain similar results for hypersurfaces 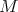 immersed with cmc
immersed with cmc 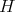 on a Lie group with bi-invariant metric. Later on, in 2005, with J. Ripoll, [F-R,2] we extend this result for hypersurfaces
on a Lie group with bi-invariant metric. Later on, in 2005, with J. Ripoll, [F-R,2] we extend this result for hypersurfaces 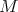 immersed with cmc
immersed with cmc 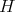 on a
on a  -dimensional Riemannian manifold
-dimensional Riemannian manifold 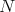 :
:
Consider the function 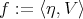 on
on 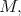 where
where 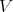 is a Killing field of
is a Killing field of 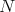 . We prove that:
. We prove that:
|
|
Using this result, similarly to those in  we prove
we prove
"if 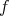 does not change sign on
does not change sign on  and
and 
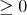 then
then 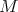 is invariant by the one parameter subgroup of isometries of
is invariant by the one parameter subgroup of isometries of 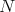 determined by
determined by  or
or 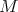 is umbilic".
is umbilic".
As consequence of this result we obtain a stability criterion for cmc surfaces in a manifold of dim 3:
"Let 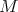 be a surface of constant mean curvature
be a surface of constant mean curvature 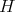 (not necessarily complete) in
(not necessarily complete) in 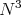 with
with 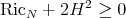 and let
and let 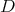 be a domain in
be a domain in 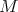 such that
such that 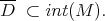 Let
Let 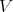 be a Killing vector field on
be a Killing vector field on 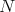 and assume that
and assume that 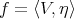 has a sign on
has a sign on 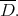 Then
Then 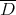 is stable".
is stable".
It follows that any radial or horizontal cmc graph in the half space model for the hyperbolic 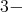 space is stable.
space is stable.
If the manifold 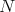 admits
admits  linearly independ Killing vector fields at each point, it is possible to define a normal Killing translation map
linearly independ Killing vector fields at each point, it is possible to define a normal Killing translation map 
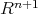
|
|
This map is a natural extension of the Gauss map of a hypersurface in 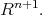
We obtain:
|
|
Using this formula we prove the following results:
Let 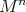 be a compact riemannian manifold immersed with cmc in a riemannian manifold
be a compact riemannian manifold immersed with cmc in a riemannian manifold 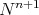 with
with 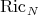
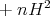
 . If
. If 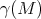 is contained in a half space of
is contained in a half space of 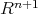 then
then 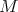 is invariant by a one parameter subgroup of isometries of
is invariant by a one parameter subgroup of isometries of 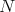 or
or 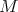 is umbilic.
is umbilic.
In particular, it arises in this context a natural and interesting extension of a conjecture of M. P. do Carmo which asserts that the Gauss image of a complete cmc surface in 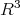 which is not a plane nor a cylinder contains a neighborhood of some equator of the sphere:
which is not a plane nor a cylinder contains a neighborhood of some equator of the sphere:
Conjecture (An extension of a conjecture of M. P. do Carmo):
Let 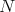 be a
be a  dimensional Killing parallelizable riemannian manifold and let
dimensional Killing parallelizable riemannian manifold and let  be a Killing basis of
be a Killing basis of 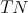 . Let
. Let 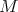 be a complete constant mean curvature hypersurface immersed in
be a complete constant mean curvature hypersurface immersed in 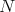 and let
and let  be the normal Killing translation map associated to
be the normal Killing translation map associated to 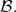 If
If 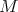 is not invariant by a Killing field generated (over the real numbers) by
is not invariant by a Killing field generated (over the real numbers) by 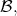 then the radial projection of
then the radial projection of 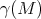 on the unit sphere covers a neighborhood of some equator of the sphere.
on the unit sphere covers a neighborhood of some equator of the sphere.
[A-D] Alias, L; Dajczer, M, "Geodesic normal graphs of constant mean curvature" preprint. [ Links ]
[D-R] Dajczer, M; Ripoll, J: "An extension of a Theorem of Serrin to graphs in warped products", preprint. [ Links ]
[ES-F-F-R] Espirito-Santo, N.; Fornari, S., Frensel K.; Ripoll, J. "Constant mean curvature hypersurfaces in a Lie group with a bi-invariant metric"; Manuscripta Mathematica 111-4 (2003), 459-470. [ Links ]
Frensel K.; Ripoll, J. "Constant mean curvature hypersurfaces in a Lie group with a bi-invariant metric"; Manuscripta Mathematica 111-4 (2003), 459-470. [ Links ]
[F-L-R] Fornari, S.; Lira, J.; Ripoll, J; "Geodesic Graphs with constant mean curvature in Spheres"; Geometria Dedicata 90, (2002), 201-216. [ Links ]
[F-R,1] Fornari, S., Ripoll, J; "Stability of compact hypersurfaces with constant mean curvature", Indiana J. Math., vol 43, no 1 (1994), 367-380. [ Links ]
[F-R,2] Fornari, S., Ripoll, J; "Killing fields, generalized Gauss map and constant mean curvature hypersurfaces"; Illinois J. Math. 48-4 (2005), 1385-1403. [ Links ]
[G-S] Earp, R. S.; Guio, E. M. "Existence and non-existence for a mean curvature equation in hyperbolic space" Communications On Pure And Applied Analysis, USA, v. 4, n. 3, (2005), p. 549-568, [ Links ]
[H-O-S] Hoffman D.; Osserman R.; Schoen R.: "On the Gauss map of complete constant mean curvature surfaces in 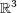 and
and 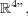 Comm. Math. Helv. 57, (1982), 519 - 531. [ Links ]
Comm. Math. Helv. 57, (1982), 519 - 531. [ Links ]
[R-R] Ritoré M., Ros A., "Stable constant mean curvature tori and the isoperimetric problem in three space forms", Comm. Math. Helv. vol. 67, N. 2, (1992} [ Links ]
[S] Serrin, J. "The problem of Dirichlet for quasilinear elliptic differential equations with many independent variables". Philos. Trans. Royal Soc. London Ser. A, 264 (1969), 413-496. [ Links ]
Susana Fornari
Departamento de Matemática - UFMG
Belo Horizonte, Brasil.
sfornari@mat.ufmg.br
Recibido: 1 de septiembre de 2005
Aceptado: 17 de septiembre de 2006