Serviços Personalizados
Journal
Artigo
Indicadores
-
 Citado por SciELO
Citado por SciELO
Links relacionados
-
 Similares em
SciELO
Similares em
SciELO
Compartilhar
Revista de la Unión Matemática Argentina
versão impressa ISSN 0041-6932versão On-line ISSN 1669-9637
Rev. Unión Mat. Argent. v.49 n.1 Bahía Blanca jan./jun. 2008
Saturated neighbourhood models of Monotonic Modal Logics
Sergio Arturo Celani
Abstract. In this paper we shall introduce the notions of point-closed, point-compact, and m-saturated monotonic neighbourhood models. We will give some characterizations, and we will prove that the ultrafilter extension and the valuation extension of a model are m-saturated.
Key words and phrases. monotonic modal logic, neighbourhood frames and models, m-saturated models, ultrafilter extension, valuation extension.
2000 Mathematics Subject Classification. 06D05, 06D16, 03G10.
Monotonic neighbourhood semantics (cf. [1] and [6]) is a generalization of Kripke semantics. It is also the standard tool for reasoning about monotonic modal logics in which some (Kripke valid) principles such as  , do not hold. A monotonic neighbourhood model, or monotonic model, is a structure
, do not hold. A monotonic neighbourhood model, or monotonic model, is a structure 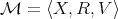 where
where 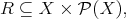
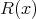 is closed under supersets for each
is closed under supersets for each 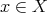 , and
, and 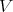 is a valuation defined on
is a valuation defined on  .
.
The main objective of this paper will be the identification and study of some properties of saturation of monotonic models. It is also intented to prove that the ultrafilter and valuation extension of a monotonic model is 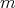 -saturated. We will define the image-compact, point-compact, point-closed, and modally saturated (or
-saturated. We will define the image-compact, point-compact, point-closed, and modally saturated (or  -saturated) models. These notions are defined in topological terms. For each monotonic model
-saturated) models. These notions are defined in topological terms. For each monotonic model 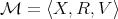 we will define a topology
we will define a topology 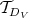 in the set
in the set
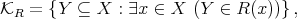
using the Boolean algebra 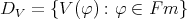 , and taking as sub-basis the collection of all sets of the form
, and taking as sub-basis the collection of all sets of the form

The topological space 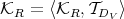 is called the hyperspace of
is called the hyperspace of 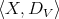 relative to
relative to 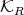 . The notions of point-compact, point-closed, and
. The notions of point-compact, point-closed, and  -saturated monotonic models are defined relative to this space. For instance, a model
-saturated monotonic models are defined relative to this space. For instance, a model 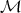 is
is 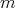 -saturated if
-saturated if 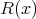 is a compact subset of
is a compact subset of 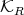 for each
for each  , and for each
, and for each 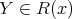 , there exists a compact set of
, there exists a compact set of 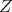 of
of 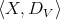 such that
such that 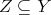 and
and 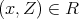 . With this notion of
. With this notion of 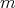 -saturation we will be able to prove that the ultrafilter extension of a monotonic model is
-saturation we will be able to prove that the ultrafilter extension of a monotonic model is 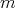 -saturated. This question has already been addressed in [6], but with a different notion of
-saturated. This question has already been addressed in [6], but with a different notion of 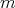 -saturation.
-saturation.
In Section 2 we will recall the principal results on the relational and algebraic semantics for monotonic modal logic. In Section 3 we will introduce the notions of compact, image-compact, point-compact, point-closed, and 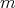 -saturated monotonic models. We will give a characterizations of point-compact models. We will show that some of the notions introduced are invariant by surjective bounded morphisms. Section 4 is the core of this paper. We shall prove that for a monotonic model
-saturated monotonic models. We will give a characterizations of point-compact models. We will show that some of the notions introduced are invariant by surjective bounded morphisms. Section 4 is the core of this paper. We shall prove that for a monotonic model 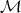 there exists at least two saturated extensions: the valuation extension and the ultrafilter extension. The valuation extension of a model is a bounded image of the ultrafilter extension, and as the property of
there exists at least two saturated extensions: the valuation extension and the ultrafilter extension. The valuation extension of a model is a bounded image of the ultrafilter extension, and as the property of 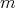 -saturation is invariant under surjective bounded morphism, we will deduce that the valuation extension is also
-saturation is invariant under surjective bounded morphism, we will deduce that the valuation extension is also 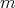 -saturated. Saturated extensions of monotonic models may be seen as a completation of the underlying frame structure. For Kripke models, the saturated extensions are modally saturated structures, which implies that modally states are bisimilar (see [7] for Kripke models and [6] for monotonic models).
-saturated. Saturated extensions of monotonic models may be seen as a completation of the underlying frame structure. For Kripke models, the saturated extensions are modally saturated structures, which implies that modally states are bisimilar (see [7] for Kripke models and [6] for monotonic models).
A monotonic algebra is a pair 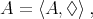 where
where 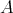 is a Boolean algebra, and
is a Boolean algebra, and 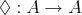 is a monotonic function, i.e. if
is a monotonic function, i.e. if 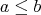 then
then 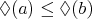 , for all
, for all 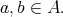 The dual operator
The dual operator  is defined as
is defined as 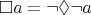 . The filter (ideal) generated by a set
. The filter (ideal) generated by a set 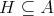 will be denoted by
will be denoted by 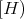 (
(![(H ])](/img/revistas/ruma/v49n1/1a1048x.png) . The set of all prime filters or ultrafilters of
. The set of all prime filters or ultrafilters of 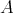 is denoted by
is denoted by  .
.
Given a set 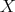 , we denote by
, we denote by 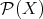 the powerset of
the powerset of 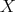 , and for a subset
, and for a subset 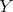 of
of 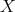 , we write
, we write  for the complement
for the complement 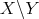 of
of  in
in 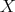 . We will call a space a pair
. We will call a space a pair 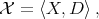 where
where 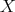 is a set, and
is a set, and 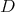 a subalgebra of the Boolean algebra of
a subalgebra of the Boolean algebra of 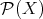 . We note that
. We note that 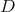 is a basis of a topology
is a basis of a topology 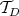 on
on 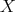 whose open sets are the unions of subsets of
whose open sets are the unions of subsets of 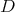 . All the elements of
. All the elements of 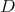 are clopen (closed and open) subsets of
are clopen (closed and open) subsets of  , because
, because 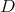 is a Boolean algebra, but an arbitrary clopen set does not to be need an element of
is a Boolean algebra, but an arbitrary clopen set does not to be need an element of 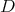 . Given a space
. Given a space 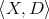 and
and 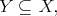 we will use the notation
we will use the notation 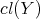 to express the closure of
to express the closure of  The set of all closed subsets (compact subsets) of
The set of all closed subsets (compact subsets) of 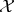 will be denoted by
will be denoted by  (
(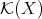 ). We note that
). We note that 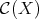 and
and 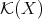 are posets under the inclusion relation. Some topological properties of
are posets under the inclusion relation. Some topological properties of  can be characterized in terms of the map
can be characterized in terms of the map 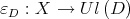 given by
given by  . The map
. The map 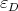 is called the insertion map in [2]. A space
is called the insertion map in [2]. A space  is called a Boolean space if it is compact and totally disconnected. If
is called a Boolean space if it is compact and totally disconnected. If  is a Boolean space, then the family
is a Boolean space, then the family 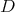 of clopen subsets is a basis for
of clopen subsets is a basis for 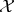 .
.
To each Boolean algebra 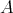 we can associate a Boolean space whose points are the elements of
we can associate a Boolean space whose points are the elements of 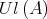 with the topology determined by the basis
with the topology determined by the basis  , where
, where 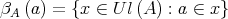 . It is known that
. It is known that 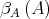 is a Boolean subalgebra of
is a Boolean subalgebra of 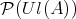 . By the explanation above we have that, if
. By the explanation above we have that, if 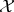 is a Boolean space, then
is a Boolean space, then 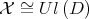 , by means of the map
, by means of the map 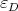 , and if
, and if 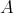 is a Boolean algebra, then
is a Boolean algebra, then 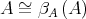 , by means of the map
, by means of the map 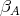 . Moreover, it is known that the map
. Moreover, it is known that the map 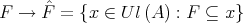 establishes a bijective correspondence between the lattice of all filters of
establishes a bijective correspondence between the lattice of all filters of 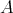 and the lattice
and the lattice 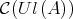 of all closed subsets of
of all closed subsets of 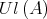 .
.
Definition 1. Let  be a space. The lower topology
be a space. The lower topology 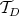 on a subset
on a subset 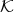 of
of 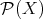 has as sub-base the collection of all sets of the form
has as sub-base the collection of all sets of the form 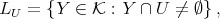 for
for 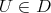 . The pair
. The pair 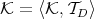 is called the hyperspace of
is called the hyperspace of 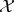 relative to
relative to  .
.
Let 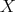 be a set. A neighbourhood relation, or multirelation, defined on
be a set. A neighbourhood relation, or multirelation, defined on 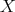 is a relation
is a relation 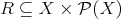 .
.
Definition 2. A monotonic neighbourhood frame, or monotonic frame, is a structure 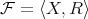 where
where 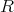 is a multirelation on
is a multirelation on 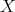 such that
such that 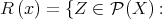
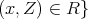 is an increasing subset of
is an increasing subset of 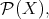 for all
for all 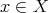 .
.
Every monotonic frame 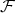 gives rise to a monotonic algebra of sets. Consider the monotonic map
gives rise to a monotonic algebra of sets. Consider the monotonic map 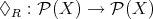 defined by:
defined by:
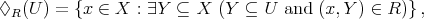
for each 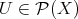 . It is clear that the pair
. It is clear that the pair 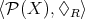 is a monotonic algebra. Using the notation introduced in Definition 1 the map
is a monotonic algebra. Using the notation introduced in Definition 1 the map 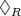 can be defined also as
can be defined also as 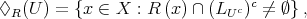 for each
for each 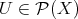 . The dual map
. The dual map 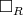 is defined by
is defined by
for each  .
.
Next we show that any monotonic algebra with monotone gives rise to a monotonic frame by invoking the basic Stone representation. In other words, we represent the elements of a monotonic algebra as subsets of some universal set, namely the set of all ultrafilters, and then define a multirelation over this universe.
Let 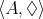 be a monotonic algebra. Let us define a multirelation
be a monotonic algebra. Let us define a multirelation 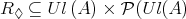 by:
by:
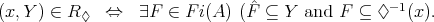 | (2.1) |
where 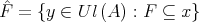 . We note that for any filter
. We note that for any filter 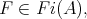 and for all
and for all 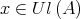 ,
,
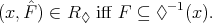
Theorem 3. Let 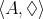 be a monotonic algebra. Then
be a monotonic algebra. Then
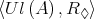 is a monotonic frame.
is a monotonic frame. for all
for all 
Proof. (1) Clearly 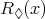 is an increasing subset of
is an increasing subset of 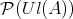 , for each
, for each 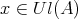 .
.
(2) We prove that for all 
 , and for all
, and for all 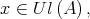
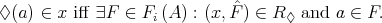
If 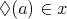 , then
, then 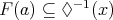 . So,
. So, 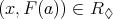 . If there exists
. If there exists  such that
such that 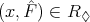 and
and 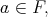 then
then 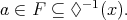 Thus,
Thus,  . As consequence of this we have that
. As consequence of this we have that 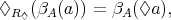 for all
for all 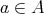 . Now it is easy to prove
. Now it is easy to prove 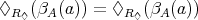 . □
. □
Let us consider a propositional language 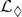 defined by using a denumerable set of propositional variables
defined by using a denumerable set of propositional variables 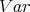 , the connectives
, the connectives 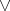 and
and 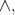 the negation
the negation  , the modal connective
, the modal connective  , and the propositional constant
, and the propositional constant 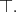 We shall denote by
We shall denote by  the operator defined as
the operator defined as  , for
, for 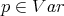 . The set of all well formed formulas will be denoted by
. The set of all well formed formulas will be denoted by 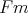 .
.
Definition 4. A monotonic model in the language 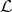 is a structure
is a structure 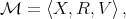 where
where 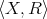 is a monotonic frame, and
is a monotonic frame, and  is a valuation.
is a valuation.
Every valuation can be extended to 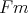 by means of the following clauses:
by means of the following clauses:
The notions of truth at a point, validity in a model and validity in a frame for formulas are defined as is usual. A formula 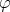 is valid at point
is valid at point 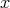 in a model
in a model 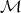 , in symbols
, in symbols  if
if 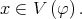 The formula
The formula 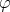 is valid in a model
is valid in a model 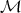 , in symbols
, in symbols 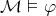 , if
, if 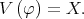
Let 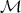 be a monotonic model. As
be a monotonic model. As 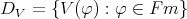 is a Boolean algebra of sets, and
is a Boolean algebra of sets, and 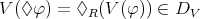 , for every
, for every  ,
,  is a monotonic subalgebra of the algebra
is a monotonic subalgebra of the algebra  . We shall denote by
. We shall denote by 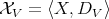 the space generated taking
the space generated taking  as a basis for a topology defined in
as a basis for a topology defined in 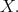 Let
Let
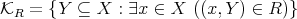
the range of the multirelation 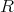 . Let
. Let 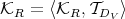 the hyperspace of
the hyperspace of 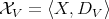 relative to
relative to 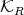 . Recall that
. Recall that 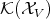 denotes the set of all compact subsets of
denotes the set of all compact subsets of  .
.
Definition 5. Let 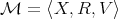 be a model. We shall say that:
be a model. We shall say that:
 is compact if the space
is compact if the space 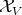 is compact,
is compact,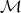 is image-compact if for all
is image-compact if for all 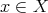 and for all
and for all 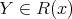 , there exists
, there exists  such that
such that 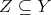 and
and 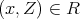 .
.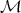 is point-compact if
is point-compact if 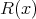 is compact subset in the topological space
is compact subset in the topological space 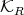 , for each
, for each 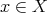 ,
,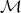 is point-closed, if
is point-closed, if 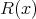 is a closed subset of the space
is a closed subset of the space 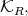 for each
for each 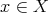 .
.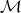 is modally saturated (or m-saturated) if it is image-compact and point-compact.
is modally saturated (or m-saturated) if it is image-compact and point-compact.
Remark 6. In [6] Hansen defines the notion of 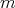 -saturated model as follows:
-saturated model as follows:
A model 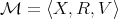 is
is 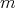 -saturated if the following conditions hold:
-saturated if the following conditions hold:
- (m1) For any
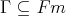 ,
, 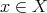 and
and 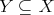 such that
such that 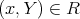 , if
, if 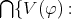
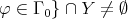 , for all finite subset
, for all finite subset  of
of 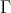 , then
, then 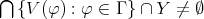 .
. - (m2) For any
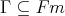 , and
, and 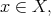 if for every finite subset
if for every finite subset 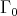 of
of 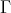 there exists an
there exists an 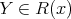 such that
such that 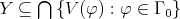 , then there exists an
, then there exists an 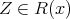 such that
such that 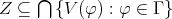 .
.
The condition (m2) is equivalent to say that the model 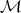 is point-compact, as we will see. But the condition (1) is not equivalent to the notion of image-compact. Hansen's definition has the disadvantage that the ultrafilter extension of a model (see definition 15) fails to meet the condition (m1). Now we will see that the condition (m2) is equivalent to require that
is point-compact, as we will see. But the condition (1) is not equivalent to the notion of image-compact. Hansen's definition has the disadvantage that the ultrafilter extension of a model (see definition 15) fails to meet the condition (m1). Now we will see that the condition (m2) is equivalent to require that 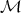 should be point-compact.
should be point-compact.
Proposition 7. Let  be a model. Then
be a model. Then 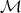 is point-compact iff it satisfies the condition (m2).
is point-compact iff it satisfies the condition (m2).
Proof.  Let
Let 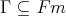 and
and 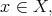 such that for every finite subset
such that for every finite subset 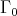 of
of 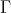 there exists an
there exists an 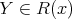 such that
such that 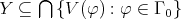 . Suppose that
. Suppose that 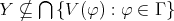 , for any
, for any 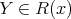 . Then for each
. Then for each 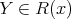 there exists
there exists 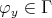 such that
such that  . So,
. So,
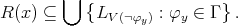
As  is a compact subset of
is a compact subset of 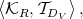 there exists a finite subset
there exists a finite subset 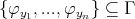 such that
such that
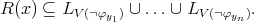 | (3.1) |
By hypothesis, there exists 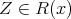 such that
such that 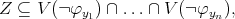 which is a contradiction to (3.1). Thus, there exists an
which is a contradiction to (3.1). Thus, there exists an 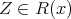 such that
such that 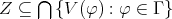 .
.
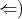 Let
Let 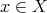 . We prove that
. We prove that 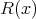 is compact subset in the topological space
is compact subset in the topological space 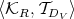 . Let
. Let 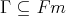 such that
such that 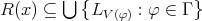 . Suppose that
. Suppose that
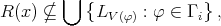
for any finite subset 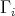 of
of 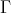 . So for each finite subset
. So for each finite subset 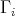 of
of 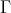 there exists
there exists 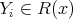 such that
such that 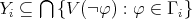 . From condition (m1), there exists
. From condition (m1), there exists 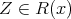 such that
such that 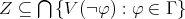 . Thus,
. Thus, 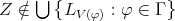 , which is a contradiction. □
, which is a contradiction. □
Let 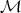 be a model. Recall that the insertion map
be a model. Recall that the insertion map  of
of 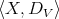 is defined by
is defined by 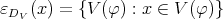 , for each
, for each 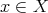 . We write
. We write 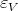 by
by 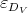 .
.
Lemma 8. Let 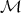 be a model. If
be a model. If 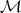 is point-closed, then
is point-closed, then
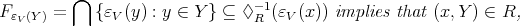
for all 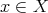 and for all
and for all  .
.
Proof. Let 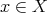 and
and 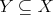 such that
such that 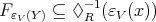 . Suppose that
. Suppose that 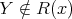 . Since
. Since 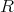 is point-closed, there exists
is point-closed, there exists 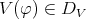 such that
such that 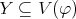 and
and 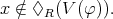 Since
Since 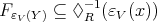 , there exists
, there exists 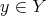 such that
such that 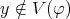 , which is a contradiction, because
, which is a contradiction, because 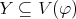 . Therefore
. Therefore 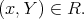 □
□
Let 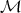 be a model. Let us consider the following property:
be a model. Let us consider the following property:
(P): For all 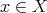 and for all
and for all 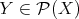 , if
, if  then there exists
then there exists  such that
such that 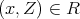 and
and 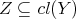 ,
,
where 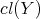 is the topological closure in the space
is the topological closure in the space 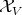 .
.
Proposition 9. Let 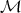 be a model. If
be a model. If 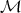 is point-compact, then
is point-compact, then 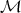 satisfies the property (P).
satisfies the property (P).
Proof. Let 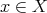 and let
and let 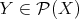 . Assume that
. Assume that
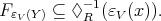 | (3.2) |
Suppose that for all 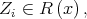
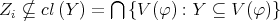 . So for each
. So for each 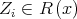 there exists
there exists 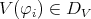 such that
such that 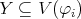 and
and 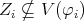 , i.e.
, i.e. 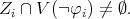 Thus,
Thus,

Since 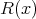 is a compact subset of
is a compact subset of 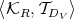 , there exists a some finite set of formulas
, there exists a some finite set of formulas  such that
such that
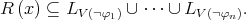
Then,
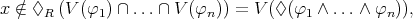
and 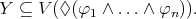 By (3.2),
By (3.2), 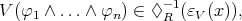 i.e.,
i.e., 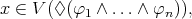 which is a contradiction. Therefore, there exists
which is a contradiction. Therefore, there exists 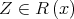 such that
such that 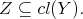 □
□
Proposition 10. Let 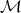 be a compact model. Then
be a compact model. Then 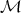 satisfies the property (P) iff
satisfies the property (P) iff  is point-compact.
is point-compact.
Proof. Suppose that 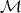 satisfies the property (P). Let
satisfies the property (P). Let 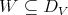 . Suppose that for every finite subset
. Suppose that for every finite subset 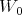 of
of 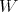
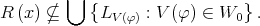 | (3.3) |
We prove that 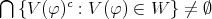 . Suppose the contrary. Then
. Suppose the contrary. Then 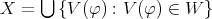 . As
. As 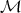 is compact,
is compact, 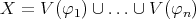 , for some
, for some  . From (3.3), there exists
. From (3.3), there exists 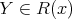 such that
such that 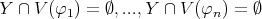 , i.e.,
, i.e., 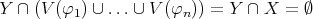 , which is impossible. Thus,
, which is impossible. Thus, 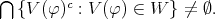 Let
Let
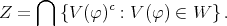
It is clear that 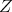 is a closed subset of
is a closed subset of 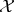 , and by compacity,
, and by compacity, 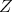 is compact. We prove that
is compact. We prove that
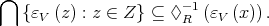
If 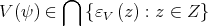 , then
, then  . By compacity, there exists a finite set
. By compacity, there exists a finite set  such that
such that
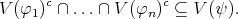
Then 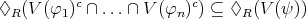 . From (3.3), there exists
. From (3.3), there exists 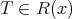 such that
such that  i.e.,
i.e., 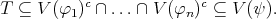 Thus,
Thus, 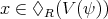 . So,
. So,
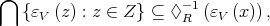
and by hypothesis there exists 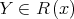 such that
such that 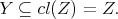 Then
Then 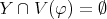 , for every
, for every 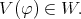 Thus,
Thus,
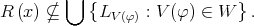
The other direction is followed by Proposition 9. □
The maps between monotonic frames and monotonic models which preserve the modal structure will be referred to as bounded morphisms. These have previously been studied in [6] (see also [5]).
Definition 11. ([6]) A bounded morphism between two monotonic models 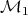 and
and 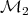 is a function
is a function  such that
such that
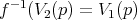 , for each propositional variable
, for each propositional variable 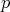 ,
,- If
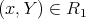 , then
, then 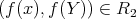 , and
, and - If
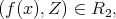 then there exists
then there exists 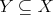 such that
such that 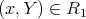 and
and 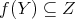 .
.
It follows that truth of modal formulas is invariant under bounded morphisms ([6]).
Proposition 12. Let 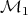 and
and 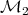 be monotonic models. If
be monotonic models. If  is a bounded morphism from
is a bounded morphism from 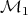 to
to 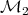 then for each formula
then for each formula 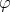 ,
, 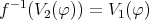 .
.
The following technical lemma is needed in the next results.
Lemma 13. Let 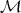 be a model. Then
be a model. Then 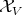 is compact iff
is compact iff 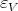 is surjective.
is surjective.
Proof. 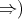 Let
Let 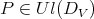 . We prove that
. We prove that 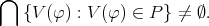 Let us suppose the opposite. Then
Let us suppose the opposite. Then  Since
Since  is compact,
is compact,  for some formulas
for some formulas  . So,
. So, 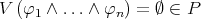 , which is impossible. It follows that there exists
, which is impossible. It follows that there exists  . Now, it is easy to see that
. Now, it is easy to see that 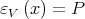 .
.
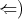 Let
Let  Suppose that for any finite subset
Suppose that for any finite subset  of
of 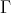 ,
, 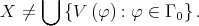 Let us consider the filter
Let us consider the filter 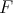 of
of 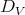 generated by the set
generated by the set 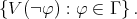 It is not difficult to prove that
It is not difficult to prove that 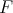 is proper. It follows that there exists an ultrafilter
is proper. It follows that there exists an ultrafilter 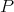 of
of 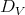 such that
such that 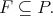 Since the map
Since the map 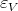 is onto, there exists
is onto, there exists 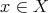 such that
such that 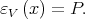 Then,
Then, 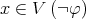 for all
for all 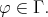 So,
So, 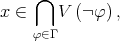 which is a contradiction. Thus, there is a finite subset
which is a contradiction. Thus, there is a finite subset 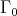 of
of 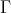 , such that
, such that  . □
. □
The concepts of compact and point-compact models are preserved by surjective bounded morphisms.
Proposition 14. Let  be a bounded morphism between the monotonic models
be a bounded morphism between the monotonic models 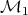 and
and 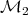 .
.
- If
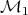 is compact, then
is compact, then 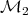 is compact.
is compact. - If
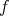 is surjective and
is surjective and 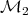 is compact, then
is compact, then 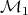 is compact.
is compact. - If
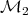 is point-compact, then
is point-compact, then 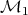 is point compact.
is point compact. - If
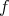 is surjective and
is surjective and 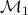 is point-compact
is point-compact then
then 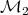 is point compact.
is point compact.
Proof. (1) By Lemma 13 we need to prove that the map  is surjective. Let
is surjective. Let 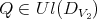 . Consider the set
. Consider the set 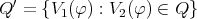 . It is easy to prove that
. It is easy to prove that 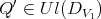 . Since
. Since 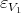 is surjective, there exists
is surjective, there exists 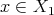 such that
such that 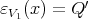 . Let
. Let 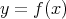 . Then, it is easy to see that
. Then, it is easy to see that 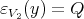 .
.
(2) By Lemma 13 we need to prove that the map  is surjective. Let
is surjective. Let 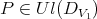 . Consider
. Consider 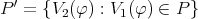 . It is easy to see that
. It is easy to see that 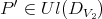 . As
. As  is surjective, there exists
is surjective, there exists 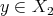 such that
such that  . Since
. Since 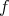 is surjective, there exists
is surjective, there exists 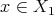 such that
such that 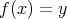 . We prove that
. We prove that 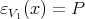 . For all
. For all 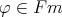 ,
, 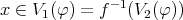 iff
iff 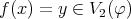 iff
iff 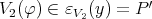 iff
iff 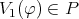 . Thus,
. Thus, 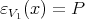 .
.
(3) Let 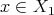 and let
and let  Suppose that
Suppose that

Taking into account that 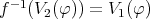 , for all
, for all 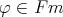 , it is easy to see that
, it is easy to see that
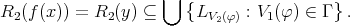
As 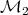 is point-compact, there exists a finite set
is point-compact, there exists a finite set  such that
such that 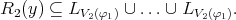 Let
Let 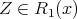 . Then
. Then 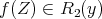 . So there exists
. So there exists 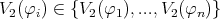 such that
such that 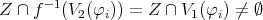 . Thus
. Thus 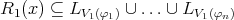 .
.
(4) Let 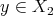 and let
and let 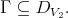 Suppose that
Suppose that
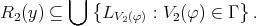
As 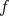 is surjective there exists
is surjective there exists 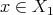 such that
such that 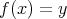 . We prove that
. We prove that
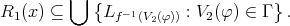 | (3.4) |
Let 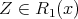 . Since
. Since 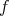 is a bounded morphism,
is a bounded morphism, 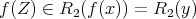 . So, there exists
. So, there exists 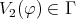 such that
such that 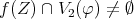 , i.e.
, i.e. 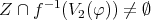 . Thus (3.4) is valid. As
. Thus (3.4) is valid. As 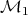 is point-compact, there exists
is point-compact, there exists 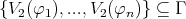 such that
such that 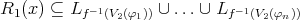 . We prove that
. We prove that
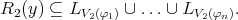
Let 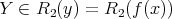 . Since
. Since 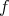 is a bounded morphism, there exists
is a bounded morphism, there exists 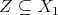 such that
such that 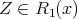 and
and 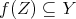 . As
. As 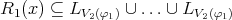 , there exists
, there exists 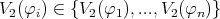 such that
such that 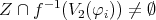 .i.e.
.i.e. 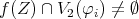 . It follows
. It follows  . Thus,
. Thus, 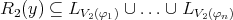 . So,
. So, 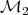 is point-compact. □
is point-compact. □
4. Ultrafilter and model extension
Let  be a model. Let
be a model. Let 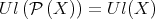 . Let
. Let 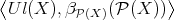 the Boolean space of the algebra
the Boolean space of the algebra 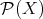 . Let us consider the ultrafilter frame
. Let us consider the ultrafilter frame 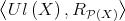 of the monotonic algebra
of the monotonic algebra 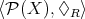 . Recall that
. Recall that 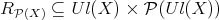 is defined as:
is defined as:
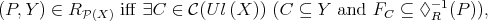
where 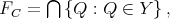 and
and 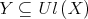 . We write
. We write 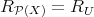 . We note that if
. We note that if 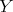 is a closed subset of
is a closed subset of 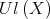 , then
, then
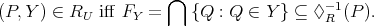
Definition 15. The ultrafilter extension of a monotonic model 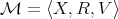 is the structure
is the structure
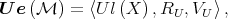
where  is a map defined by:
is a map defined by:
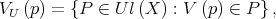
for every 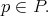
It is easy to see that 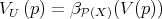 , for each
, for each 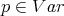 (see [6]).
(see [6]).
Given a model  we can define another extension taking the set
we can define another extension taking the set 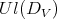 as the base set of a model. Let
as the base set of a model. Let 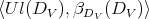 be the Boolean space of
be the Boolean space of 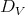 . Let us consider the ultrafilter frame
. Let us consider the ultrafilter frame 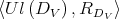 of the monotonic algebra
of the monotonic algebra 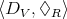 , where
, where  is defined by
is defined by
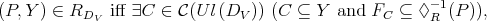
where 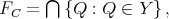 and
and 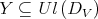 .
.
Definition 16. The valuation extension, or valuation model, of a model  is the structure
is the structure
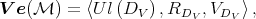
where the function 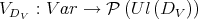 is defined by
is defined by
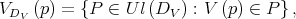
for every 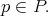
We note that 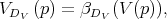 for each
for each  .
.
Theorem 17. Let 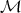 be a monotonic model. Then for any
be a monotonic model. Then for any 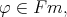
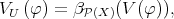 and
and 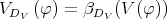 ,
,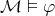 iff
iff 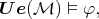 and
and 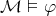 iff
iff 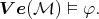
Proof. We prove  , for any
, for any 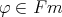 . The other proof is very similar. The proof is by induction on the complexity of
. The other proof is very similar. The proof is by induction on the complexity of 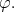 We consider the case
We consider the case 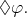 Let
Let 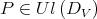 . Let
. Let 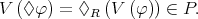 Let us consider the filter
Let us consider the filter 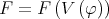 in the Boolean algebra
in the Boolean algebra 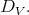 Then it is easy to see that
Then it is easy to see that 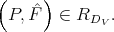 For the other direction, suppose that
For the other direction, suppose that 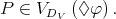 Then there exists a filter
Then there exists a filter  of
of 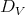 such that
such that

By inductive hypothesis we have 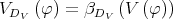 . Thus,
. Thus, 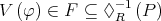 , i.e.,
, i.e., 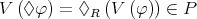 .
.
(2). We prove 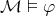 iff
iff 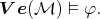 The other proof is similar. Assume that
The other proof is similar. Assume that 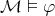 . Then,
. Then, 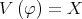 . But
. But  belong to every ultrafilter of
belong to every ultrafilter of 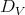 . Then,
. Then, 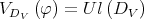 , i.e.,
, i.e., 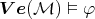 . Now, if
. Now, if  , then there exists
, then there exists 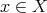 such that
such that 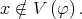 Then,
Then, 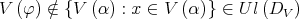 . Thus,
. Thus,  □
□
Theorem 18. Let 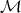 be a model. Then the model
be a model. Then the model 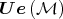 is
is 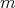 -saturated.
-saturated.
Proof. Let 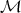 be a monotonic model. We prove that
be a monotonic model. We prove that 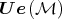 is compact i.e.,
is compact i.e., 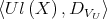 is a compact space. From Lemma 13 it is enough to prove that the map
is a compact space. From Lemma 13 it is enough to prove that the map 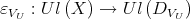 is surjective. Let
is surjective. Let  . Consider the filter
. Consider the filter 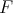 in
in 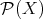 generated by
generated by 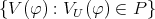 . It is clear that
. It is clear that 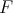 is proper. So there exists
is proper. So there exists 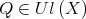 such that
such that 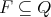 . By construction
. By construction 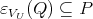 . So,
. So, 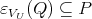 , and consequently
, and consequently  is surjective.
is surjective.
We prove that 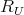 is point-compact in the hyperspace
is point-compact in the hyperspace 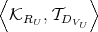 , where
, where 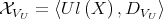 . As
. As 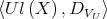 is a compact space we will apply Proposition 10. Let
is a compact space we will apply Proposition 10. Let 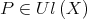 and let
and let 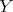 be a subset of
be a subset of 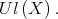 Suppose that
Suppose that
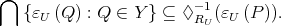
We need to prove that there exists a subset 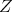 of
of 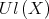 such that
such that 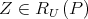 and
and 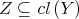 , where
, where 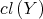 is the topological closure of
is the topological closure of  in the topological space
in the topological space 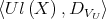 .
.
Consider the filter 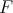 generated by the set
generated by the set 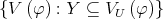 . We prove that
. We prove that
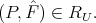
Let 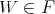 . Then there exists
. Then there exists 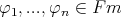 such that
such that
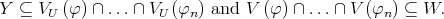
So, 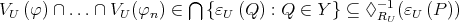 . Then
. Then

i.e. 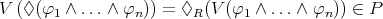 . Then
. Then  . Thus
. Thus 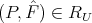 . Taking into account that the topological closure of
. Taking into account that the topological closure of 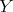 in the space
in the space 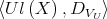 is
is 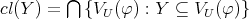 , it is easy to prove that
, it is easy to prove that  . Therefore
. Therefore 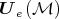 is
is 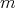 -saturated. □
-saturated. □
Theorem 19. Let 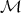 be a model. Then the model
be a model. Then the model 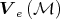 is
is 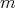 -saturated.
-saturated.
Proof. Since 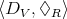 is a subalgebra of the algebra
is a subalgebra of the algebra 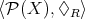 , we can define a map
, we can define a map 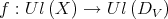 by
by 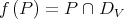 . By well-established results in the duality theory of Boolean algebras the map
. By well-established results in the duality theory of Boolean algebras the map 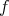 is the dual map of the inclusion homomorphism between
is the dual map of the inclusion homomorphism between 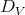 and
and 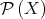 . From the results given by H. Hansen [6] we get that
. From the results given by H. Hansen [6] we get that 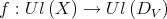 is a surjective bounded morphism between the ultrafilter extension
is a surjective bounded morphism between the ultrafilter extension 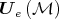 and the valuation extension
and the valuation extension 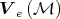 . Since
. Since 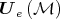 is
is 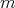 -saturated
-saturated by Proposition 14 we get that
by Proposition 14 we get that 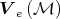 is also
is also 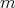 -saturated. □
-saturated. □
[1] B. F. Chellas, Modal Logic: an introduction, Cambridge Univ. Press,1980. [ Links ]
[2] R. Goldblatt, Maps and Monads for Modal Frames, Studia Logica Volume 83, Numbers 1-3 (2006), pp.309-331. [ Links ]
[3] R. Goldblatt, Mathematics of Modality, CSLI Lectures Notes 43, 1993. [ Links ]
[4] R. Goldblatt, Logics of Time and Computation, CSLI Lectures Notes 7, Second Edition, 1992. [ Links ]
[5] H. H. Hansen and C. Kupke. A Coalgebraic Perspective on Monotone Modal Logic, Electronic Notes in Theoretical Computer Science, 106, pages 121-143, Elsevier, 2004. [ Links ]
[6] H. H. Hansen. Monotonic modal logic (Master's thesis). Preprint 2003-24, ILLC, University of Amsterdam, 2003. [ Links ]
[7] M. J. Hollenberg, Hennessey-Milner Classes and Process Algebra. In M. de Rijke A. Ponse and Y. Venema, editors, Modal Logic and Process Algebra: a Bisimulation Perspective, volume 53 of CSLI Lecture Notes, pages 187-216. CSLI Publications, 1995. [ Links ]
Sergio Arturo Celani
CONICET and Departamento de Matemáticas,
Facultad de Ciencias Exactas,
Universidad Nacional del Centro, Pinto 399,
7000 Tandil, Argentina
scelani@exa.unicen.edu.ar
Recibido: 10 de abril de 2008
Aceptado: 28 de abril de 2008