Natural frequencies of thin rectangular plates with partial intermediate supports

M.R. Escalante^{1, 2}, M.B. Rosales^{1, 3} and C.P. Filipich^{1, 4}

¹ Departamento de Ingeniería, Universidad Nacional del Sur, 8000 Bahía Blanca, Argentina.
² F.R.C.U., Universidad Tecnológica Nacional, 3260 C. del Uruguay, Argentina.
escalam@frcu.utn.edu.ar
³ CONICET, Argentina.
mrosales@criba.edu.ar
⁴ F.R.B.B., Universidad Tecnológica Nacional, 8000 Bahía Blanca, Argentina.

Abstract — In the present study, a methodology to find natural frequencies with arbitrary precision of thin rectangular plates on linear intermediate supports and mixed boundary conditions is presented. This means that the edges are total or partially supported, clamped or free, or any combination of these. The layout, number and place of linear intermediate supports are arbitrary, which allows for the analysis of a wide range of cases that include intermediate supports of different kinds: simple and multiple, straight and curved, complete (the ends coincide with the plate edges) and partial (at least one of the ends is not coincident with the plate edges). In the case of curved linear supports, the curve can be open or closed. The generalized solution is obtained using the Whole Element Method. A continuous and a discrete model of equidistant points are studied both for intermediate supports and clamped edges. In all cases, both a systematic approach to the solution and the theoretical basis, which ensures the arbitrary precision of the results, should be emphasized. In order to illustrate the accuracy and efficiency of the method described, numerical results are presented for several problems and comparison is made with previously published results in some cases and in some others with the Finite Element Method. These numerical results may be of interest to design engineers and researchers who conduct vibration studies.

Keywords — Plates. Free Vibrations. Intermediate Supports. Exact Frequencies.

I. INTRODUCTION
Rectangular plates on intermediate supports find use in many engineering structures and other areas of practical interest, such as slabs on columns, printed circuit boards or solar panels supported at a few points. With their potential applications, the vibration of plates with internal supports and with complex boundary conditions has received considerable attention from researchers. The free flexural vibration of rectangular plates has been the subject of numerous studies, many of which have been discussed by Leissa (1969, 1981,1987). Plates involving various complexities have been considered, including the case in which the plate is supported by internal lines. For such plates, most of the reported work has been concerned with plates with internal line supports, which are straight and parallel to the edges of the plates. Some examples of theses studies are the works by Veletsos and Newmark (1956), Wu and Cheung, (1974), Elishakoff and Stemberg (1979). The literature on the vibration of rectangular plates for which the internal line supports are not parallel to the edges is sparse. Two examples of such work are that by Gorman (1979) who studied the vibration of diagonally supported rectangular plates, and that by Takahashi and Chishaki (1979), who represented the internal line support by rows of equidistant point supports and gave frequency parameters and mode shapes for a rectangular plate with an oblique line support passing through its center at various angles. Li and Gorman (1992) considered rectangular plates with free edges and intermediate linear supports along one or two diagonals with the superposition method. Other studies of rectangular plates with an oblique intermediate support were carried out by Kim (1995) and Sanzi and Laura (1989) who used the Rayleigh-Ritz method. Young and Dickinson (1993) used the Rayleigh-Ritz method to obtain the eigenvalues for the free vibration of rectangular plates with the supports lying along different types of curves, including a central circular support. Cheung and Kong (1995) used modified single-span vibrating beam functions with the finite layer method to study the vibration of shear-deformable plates with intermediate line supports. Another general study in this particular area is that by Fan and Cheung (1984), in which they used the spline strip element method to analyze plates with complex boundary conditions and point supports.
Huang and Thambiratnam (2001) used the finite step element method combined with a spring system to treat the free vibration analysis of plates on elastic intermediate supports. Saadatpour et. al. (2000) developed a numerical technique for the dynamic analysis of arbitrary quadrilateral -shaped plates with internal supports.
We must emphasize that in all these works the intermediate supports are complete, i.e., the ends coincide with the plate edges.
More recently, Zhao and Wei (2002) used the discrete singular convolution (DSC) algorithm for the analysis of rectangular plates with non-uniform and combined boundary conditions. Finally Zhao et al. (2002), who made an extensive bibliography review about the problem of vibration of thin plates with internal supports, proposed a novel computational method to study the problem of plate vibration under complex and irregular internal support conditions.
Except for this last paper, the authors are not aware of any other work published in the open literature dealing with plates with partial internal line supports, which is the main objective in this work.
Technically, it is more difficult to analyze plates with irregular or partial internal supports. The problem is far more complicated to admit an analytical solution. For example, this problem is difficult to tackle by the Ritz Method if the internal support topology cannot be analytically expressed, even using penalty approaches. It is evident that the partial internal support is still a challenge for the Ritz method and it has not been solved by it yet.

II. FORMULATION OF THE PROBLEM
The vibrational problem is analyzed within the classical theory of thin plates (Germain-Lagrange) using the Whole Element Method (WEM). This is a direct variational method previously founded and developed for boundary value problems, as well as those with initial conditions and others governed by partial differential equations, uni, bi or tridimensional domains, conservative or not, linear or not: Rosales (1997), Filipich et. al. (1998, 1999), Rosales et al. (1999), Filipich and Rosales, (1997, 1999a, 1999b), Rosales and Filipich (2000), Rosales et al. (2000a,b).
It starts from an ad-hoc functional (in this problem, the energetic functional) and proposing an extremizing sequence of functions belonging to a complete set in L₂.
Unlike the Ritz Method in which each coordinate function must satisfy the boundary conditions, WEM requires the complete sequence to satisfy these conditions. Eventual non-satisfied conditions are taken into account by Lagrange multipliers. The sequences used are extended trigonometric series, which are systematically generated. It should be noted that the previous study of the mode shapes is not necessary.
In all cases, both a systematic approach to the solution and the theoretical basis that ensures the arbitrary precision (accuracy) of the results should be emphasized.
The results obtained with WEM are contrasted with the values obtained from the classical solution when this is available and with other ones obtained by others authors by approximate methods and the Finite Element Method (FEM) using the ALGOR^® software (Algor, 1999).
It is assumed that the plate under consideration is a thin rectangular plate, lies in the x-y plane, is bounded by the edges X = 0, X = a, Y = 0 and Y = b, of uniform thickness h_p, isotropic material, uniform density r, Young Modulus E.
In Fig. 1, a rectangular plate with a partial intermediate support with arbitrary design is shown. Let with 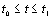be the equation of the intermediate support

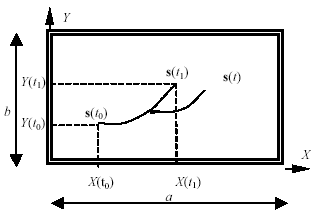
Figure 1: rectangular thin plate on intermediate linear supports

The energetic functional corresponding to the free vibrations of the thin rectangular plate, using the German-Lagrange theory, is:

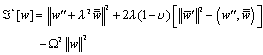(1)

where,,

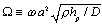w denotes the transverse displacement and the Poisson's ratio, being, , etc. There are also introduced the non-dimensional quantities

(2)

in order to work in the domain.
WEM requires an extremizing sequence, which in the thin plates problem is a bidimensional sequence. Being the functional under study, quadratic and positive defined, this sequence will be minimizing. When the plate problems are tackled with direct methods, boundary conditions that involve essential functions must be imposed, where the latter are the conditions that involve the solution and its derivative functions up to the order k-1, being 2k the order of the differential equation that govern the problem. Thus, both the displacement function and its first order derivatives must verify uniform convergence. One of the possible sequences to use is:

(3)

where, , ,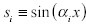

and A_ij, A_i0, A_0j, a_i, b_j, A₀₀, a₀, b₀, k₀ are the unknown coefficients, Escalante (2001).
Introducing the boundary conditions (B.C.) for the case of a rectangular simple supported plate in , that is:

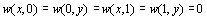
and

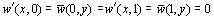
the sequence is reduced to:

(4)

A. Intermediate Supports

To illustrate the methodology we consider a simply supported rectangular plate with an internal support. We present two different models: continuous and discrete.

A.1. Continuous Model

In this case, the expression of the extended functional to be used is:

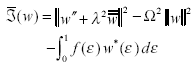(5)

where the two first terms correspond to the energetic functional for thin plates with their edges simply supported. The last term puts in evidence the nullity of the transversal displacement on the internal support, by means of the integral that represents the virtual work of the reaction. In that integral, plays the role of a Lagrange multiplier. Next, we show the reasoning by which we obtain that integral.

Let be the distributed reaction on the intermediate support (unknown), the parametric equation of the support and 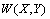the transversal displacement, the virtual work of the reaction on the support must be vanished, that is

(6)

where again, by changing to the new variables indicated in we obtain

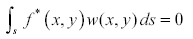(7)

being

the transversal displacement equations, the support reaction and the Cartesian equation of the support in dimensionless coordinates respectively. Equation written as a function of the parameter t is:

(8)

Defining:

(9)

isolating t:

(10)

and replacing it in we obtain:

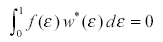(11)

where

and

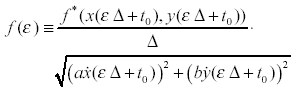
that is proportional to the reaction on the internal support and in general it is not object of study.

Next, we should apply the condition to minimize equation , that is

(12)

However, this would yield again to in [0,1], which we already knew and thus is not useful. We must think, then, that the Lagrange condition is actually an infinite sum, i.e.

(13)

To be consequent with WEM, we propose to expand the unknown reaction as an extended trigonometric series, that is

(14)

where a_p, a₀, k, b_p and c_p are unknown constants. As we know both (14)(a) and (14)(b) have uniform convergence for all continuous in [0,1].

Then, the extended functional in case we adopt the series given by (14)(c) for is:

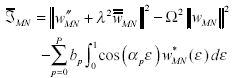(15)

being , with p = 0, 1, ..., P

Now, applying the condition and integrating it we yield to

(16)

where:

(17)

and

(18)

For equation (16) to be satisfied for any arbitrary variations of dA_ij and db_p the following equations must be verified:

(19)

Isolating A_ij from (19)(a) and replacing it in (19)(b) we obtain

(20)

Defining

(21)

equation can be written as follows:

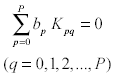(22)

The fundamental equation represents a homogeneous linear system of order from which frequencies W_k (k = 0, 1 ,2, ..., P) can be obtained.

A.2 Discrete Model

The second alternative that we propose in order to tackle the intermediate support is a discrete model considering it as an equidistant set of points.
Let with be again the parametric equation of the intermediate linear support, P intermediate points are considered, whose dimensionless coordinates are

with and
As the support has been replaced by the set of equidistant points, the condition of null transversal displacement is required for each of them. That is obtained by using Lagrange multipliers.
Thus, the extended functional will be:

(23)

By vanishing the first variation we obtain:

(24)

being:

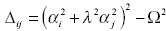(25)

and

(26)

For equation (16) to be satisfied for any arbitrary variations of dA_ij and dl_k the following equations must be verified:

(27)

Isolating A_ij from (27)(a) and replacing it in (27)(b) we have

(28)

Defining:

(k, q = 0, 1, 2, ..., P-1)

(29)

equation (20) is reduced to

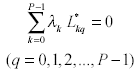(30)

Equation (30) represents a homogeneous linear system with order P from which P frequencies W_k ( k = 1, 2, ..., P) can be obtained.

B. Partially Clamped Edges

The same ideas exposed before to deal with intermediate supports can be applied to model total or partial clamped edges. In analogous way, we have both a continuous and discrete model as well.
For brevity we show only the discrete model. The partially clamped edges are dealt with the same way as the intermediate supports. The partially clamped edge is replaced by Q equidistantly spaced points, to which normal rotation must be restrained. That is, the first normal derivative is equal to zero:

(31)

being

,

is the operator gradient and is the unit normal vector (director cosines) on the clamped edge. Then, per each partial clamped edge we need to add the terms to put them in evidence in the functional. i.e.

(32)

where h_q is the Lagrange multiplier.

C. Convergence Study

To obtain the eigenvalues with the desired precision, the convergence study must be done adopting at first, a fixed value for P. This means adopting a rigid model of P points for the intermediate support. Then, the M and N values are increased until the desired precision is attained. Next, a larger value for P is adopted and the calculations are repeated increasing again the M and N values. The eigenvalue obtained in this step will have a higher or equal value than the obtained in the previous step because this model is more rigid.

III. NUMERICAL RESULTS
In order to illustrate the accuracy and utility of the above-described approach, numerical results are presented for several examples and comparison is made with previously published results when possible.
In Table 1 natural frequencies of a rectangular simply supported plate are given for two different cases of intermediate linear support. Results are obtained for both, continuous and discrete model and they are contrasted with the results obtained by the Finite Element Method.

Table 1: Frequencies Parameters. A: Continuous model, M = N =300, P = 50, B: Discrete model, C: FEM (ALGOR), Mod. Model C: M = N = 300, P = 30; MEF (ALGOR) Mesh: 1024 elements. P₀=(x₀, y₀), P₁=(x₁,y₁).

Next, natural frequencies of the square plate with various boundary conditions and an intermediate support along its diagonal are given in Table 2.
It should be noted that as long as the plate under study has partial or totally free edges, the energetic functional used by this methodology includes the terms corresponding to the Gaussian curvature.
The results are contrasted with the values obtained by the Finite Element Method (FEM) by using the ALGOR^® software and with those obtained by Kim (1995) by the Rayleigh-Ritz Method using a polynomial orthogonal set as trial functions.
The case of a square plate with various boundary conditions and a partial intermediate support is shown in Table 3. The results are compared only with the values obtained by the FEM (ALGOR^®), since according to the authors, no others previous results have been published in the open literature, which deal with partial intermediate supports using other methodologies.
The numerical results corresponding to the case of a square plate simply supported and a partial intermediate curved support are also shown in Table 4. In this example, the internal support is a circumferential arc with radius r = 0.25 and center coincident with that of the plate.

Table 2: Natural frequencies of a plate with various boundary conditions and an intermediate support along its diagonal. WEM: M = N = 300, P = 30, Q = 90, u = 0.3. FEM (ALGOR): mesh of 1600 elements. (*) Kim (1995)

Finally, the numerical results for two examples of rectangular plates with multiple intermediate supports are given in Table 5. The frequency parameters are compared again with the values obtained by FEM using the software ALGOR since there are not any known values obtained with other methodologies.

Table 3: Natural frequencies of a square plate with varied boundary conditions and a partial intermediate support. WEM: M = N = 300, P = 30, Q = 15, u = 0.3. FEM (ALGOR): mesh of 1600 elements.

Table 4: Natural frequencies of a fully simple square plate with an intermediate circumferential arc support. Center (0.5,0.5), r = 0.25. WEM: M = N = 400, a) P = 20, b) P = 30, c) P = 35, P = 40. FEM (ALGOR) Mesh of 1600 elements. (*) Young and Dickinson (1993).

The plate is simply supported on its edges and the three lowest eigenvalues are given.

V. CONCLUSIONS
In this work a methodology to determine natural frequencies of thin rectangular plates, with arbitrary precision using the Whole Element Method (WEM) was shown. This is a direct variational method previously founded and developed for boundary value problems, as well as those with initial conditions and others governed by partial differential equations, uni, bi or three-dimensional domains, conservative or not, linear or not. It starts from an ad-hoc functional (in this problem, the energetic functional) and proposing an extremizing sequence of functions belonging to a complete set in L₂. It should be noted that the previous study of the mode shapes is not necessary.
The boundary conditions must be satisfied by the complete sequence and eventual non-satisfied conditions are taken into account by Lagrange multipliers. The sequences used are extended trigonometric series, which are systematically generated and formally identical in all cases.
Two models (discrete and continuous) were proposed to consider the intermediate supports. In both cases, the efficiency is similar, however the discrete model is more practical and simpler to implement by computational algorithms.
Although we limited our attention to the vibration of rectangular thin plates with linear intermediate supports, the methodology can be used to analyze plates with complex and irregular internal support conditions as well.

Table 5: Natural frequencies parameters of rectangular plates with multiple partial intermediate supports. l : aspect ratio. WEM: P = 20, M = N = 300, FEM: Mesh of 5120 elements.

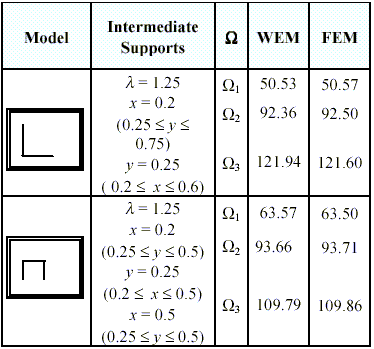
In the examples we have analyzed, a discrete model for the clamped edges was adopted. Then the effect of the clamped segment on the edges on the vibration is taken into account by replacing the clamped segment on the edges with rows of equidistantly spaced points. These, in turn, are restrained from either transverse deflection or normal rotation respectively.
The generality of the model studied is one of the most important advantages, since a unique computational algorithm allows determining the natural frequencies of thin plates with various and non-classical boundary conditions and with multiple internal linear or irregular supports.
It has been shown that the methodology proposed may be used successfully. Good agreement is observed for all cases and the results can be used for comparative studies by future researchers.

REFERENCES
1. ALGOR Software. Versión 12.04 WIN 1999. Pittsburg, USA: Algor Inc.         [ Links ]
2. Cheung Y.K. and Kong J. "The application of a new finite strip to the free vibration of rectangular plates of varying complexity". Journal of Sound and Vibration 181, 341-353 (1995).         [ Links ]
3. Elishakoff, I. and Sternberg, A. "Eigenfrequencies of continuous plates with arbitrary number of equal spans," Journal of Applied Mechanic. 46, 656-662 (1979)         [ Links ]
4. Escalante, Mario R. "Frecuencias naturales de placas rectangulares delgadas con apoyos no convencionales utilizando una solución generalizada," Tesis de Magíster en Ingeniería, Universidad Nacional del Sur, Argentina (2001).         [ Links ]
5. Fan S.C. and Cheung Y.K. " Flexural free vibrations of rectangular plates with complex support conditions" Journal of Sound and Vibration 93(1), 81-94 (1984).         [ Links ]
6. Filipich, C. P. and Rosales, M. B. "A variational solution for an initial conditions problem". Applied Mechanics Review 50, S50-S55 (1997).         [ Links ]
7. Filipich, C. P. and Rosales, M. B. "Arbitrary precision frequencies of a free rectangular thin plate," Journal of Sound and Vibration 230, 521-539, (1999)         [ Links ]
8. Filipich, C. P. and Rosales, M. B. "Dynamic behavior of a uniform linear beam supported with non-linear rotational springs," Proceedings of the 4^th World Congress on Computational Mechanics (WCCM), Buenos Aires, Argentina. CD-ROM (1999b).         [ Links ]
9. Filipich, C. P., Rosales, M. B. and Bellés, P. M. "Natural vibration of rectangular plates considered as tridimensional solids," Journal of Sound and Vibration 212, 599-610 (1998)         [ Links ]
10. Filipich, C. P., Rosales, M. B. And Palma, E. D. "A one-dimensional advection-diffusion equation solved via an arbitrary precision generalized solution," Proceedings of the 20^th. Iberian Latin-American Congress On Comp. Methods In Eng. (XX CILAMCE), CDROM, San Pablo, Brasil, (1999).         [ Links ]
11. Gorman, D. J. "Solutions of the Lèvy type for the free vibration analysis of diagonally supported rectangular plates," Journal of Sound and Vibration 66, 239-246 (1979)         [ Links ]
12. Huang M.H. and Thambiratnam D.P. "Free vibration analysis of rectangular plates on elastic intermediate supports". Journal of Sound and Vibration 240(3), 567-580 (2001).         [ Links ]
13. Kim, C. S. "Free vibration of rectangular plates with an arbitrary straight line support," Journal of Sound and Vibration 180, 769-784 (1995).         [ Links ]
14. Leissa, A. W. "Plate vibration research, 1976-1980: classical theory," The Shock and Vibration Digest, 13(9), 11-22 (1981).         [ Links ]
15. Leissa, A. W. "Recent studies in plate vibrations 1981-1985 part I: classical theory," The Shock and Vibration Digest 19(2), 11-18 (1987).         [ Links ]
16. Leissa, A. W. Vibration of plates, NASA SP -160. Office of Technology Utilization, NASA, Washington, D.C. (1969)         [ Links ]
17. Li, N. and Gorman, D. J. "Free vibration analysis of rectangular plates with free edges and line support along diagonals," American Institute of Aeronautics and Astronautics Journal 30, 2351-2353 (1992).         [ Links ]
18. Rosales, M. B. "A non-classical variational method and its application to statics and dynamics of structural elements"(in Spanish). Ph.D. Thesis, Universidad Nacional del Sur, Argentina (1997).         [ Links ]
19. Rosales M. B., Filipich C. P. and Escalante M. R. "Frecuencias arbitrariamente precisas de placas apoyada en sus vértices," Proc. del 6^to Congreso Argentino de Mecánica Computacional, CDROM, Mendoza, Argentina (1999).         [ Links ]
20. Rosales, M. B. and Filipich, C. P. "Vibraciones de membranas de forma arbitraria a través de una solución generalizada," Proc. of the XI Congress on Numerical Methods and their Applications, ENIEF 2000, Bariloche, Argentina (2000).         [ Links ]
21. Rosales, M. B., Filipich, C. P. and Escalante, M. R. "Comp. dinámico de losas rectangulares con apoyos internos," Proc. del Congreso de Ingeniería 2000, CDROM, Buenos Aires, Argentina (2000a).         [ Links ]
22. Rosales, M. B., Filipich, C. P. and Escalante, M. R. "Análisis dinámico de placas rectangulares con apoyos lineales intermedios parciales rectos o curvos," Proc. of the XI Congress on Numerical Methods and their Applications, ENIEF 2000, Bariloche, Argentina (2000b).         [ Links ]
23. Sanzi H.C. and Laura P.A.A. "Fundamental frequency of continuous plates: the effect of oblique supports". Journal of Sound and Vibration 129, 517-519 (1989)         [ Links ]
24. Saadatpour M.M., Azhari M. and Bradford M.A. " Vibration analysis of simply supported plates of general shape with internal point and line supports using the Galerkin method". Engineering Structures 22, 1180-1188 (2000).         [ Links ]
25. Takahashi, K and Chishaki, T. "Free vibrations of a rectangular plate on oblique supports," Journal of Sound and Vibration 60, 299-304 (1979).         [ Links ]
26. Veletsos, A. S. and Newmark N. M. "Determination of natural frequencies of continuous plates hinged along two apposite edges," Journal of Applied Mechanics 23, 97-102 (1956).         [ Links ]
27. Wu, C. I. and Cheung, Y. K. "Frequency Analysis of rectangular plates continuous in one or two directions," Journal of Earthquake Engineering and Structural Dynamics. 3, 3-14 (1974).         [ Links ]
28. Young, P. G. and Dickinson, S. M. "On the free flexural vibration of rectangular plates with straight or curved internal line supports," Journal of Sound and Vibration 162(1), 123-135 (1993).         [ Links ]
29. Zhao Y.B. and Wei G.W. "DSC. Analysis of rectangular with non-uniform boundary conditions". J. of Sound and Vibration 255(2), 203-228 (2002).         [ Links ]
30. Zhao Y.B., Wei G.W and Xiang Y. "Plate vibration under irregular internal support" International J. of Solids and Structures 39, 1361-1383 (2002).        [ Links ]