ARTICLES

Free and forced convective MHD oscillatory flow over an infinite porous surface in an oscillating free stream

S. Ahmed

Department of Mathematics, Goalpara College, Goalpara, Assam - 783101, INDIA.
e-mail: sahin_glp@yahoo.co.in

Abstract — This paper deals with the mixed convection hydromagnetic oscillatory flow and periodic heat transfer of a viscous incompressible and electrically conducting fluid past an infinite vertical porous plate. The plate is subjected to a constant suction velocity and heat absorbing sinks, while the free stream is oscillating with time. A magnetic field of uniform strength is applied in the direction normal to the plate. The transient, nonlinear and coupled governing equations are solved using multi-parameter perturbation technique. Approximate solutions have been derived for the velocity and temperature fields as well as mean skin-friction and mean rate of heat transfer. It is found that, the increase in magnetic field strength leads to decrease transient velocity as well as temperature. Further, the amplitude (|H|) as well as phase (tanß) of the mean rate of heat transfer increases with increasing magnetic field strength (M) for electrolytic solution (Pr=1.0), while a reverse phenomenon is observed for mercury (Pr=0.025).

Keywords — Unsteady Flow; MHD; Heat Transfer; Skin-Friction; Free Stream.

I. INTRODUCTION

Unsteady mixed convection MHD flow past a vertical porous plate has been studied widely because of its importance in aeronautics, missile aerodynamics, etc. When the difference between the wall temperature and the ambient fluid temperature is quite appreciable, it causes free convection currents to flow in the boundary layer and consequently the skin-friction and rate of heat transfer at the walls are affected. The transverse magnetic field and suction or injection at the walls also influences the flow pattern and hence the skin-friction and the rate of heat transfer at the walls to large extent. The study of such flow was initiated by Lighthill (1954) who studied the effects of free oscillations on the flow of a viscous incompressible fluid past an infinite plate. The theory was extended for free convection boundary layers along a semi-infinite vertical plate by Nanda and Sharma (1963). Messiha (1966) studied two-dimensional incompressible fluid flow problem along an infinite flat plate with no heat transfer between the fluid and the plate when the suction velocity normal to the plate as well as the external flow varies periodically with time. Further Raptis and Perdikis (1985) studied the unsteady two-dimensional free convective flows through highly porous medium. Recently, Ahmed and Ahmed (2004) analyzed the effect of two-dimensional MHD oscillatory flow along a uniformly moving infinite vertical porous plate bounded by porous medium. Later on, the effects of unsteady free convective MHD flow through a porous medium bounded by an infinite vertical porous plate were investigated by Ahmed (2007).

Soundalgekar (1974) studied the effects of free convection currents on steady MHD flow past a vertical porous plate. Pop and Soundalgekar (1974) have investigated unsteady free convection flow past an infinite plate with constant suction and heat sources. Vighnesam and Soundalgekar (1998) studied the combined free and forced convection flow of water from a vertical plate with variable temperature. Further, Ogulu and Prakash (2006) investigated the effects magnetic field on heat transfer unsteady flow past an infinite moving vertical plate with variable suction. Singh and Cowling (1963) have considered the effect of magnetic field on free convective flow of electrically conducting fluids past a semi-infinite flat plate. Sacheti et al. (1994) derived an exact solution for the unsteady MHD problem. Sattar and Alam (1995) have presented the MHD free convective flow with Hall current in a porous medium for electrolytic solution (viz. salt water). But they have neither considered the effect of constant suction nor included the heat absorbing sink and viscous dissipative term. The analysis of propagation of thermal energy through mercury and electrolytic solution in the presence of external magnetic field and heat absorbing sinks has wide range of applications in chemical and aeronautical engineering, atomic propulsion, space science etc. In view of this, Sahoo et al. (2003) have analyzed the effects of MHD unsteady free convection flow past an infinite vertical plate with constant suction and heat sink. Therefore, the main objective of this study is to investigate the effect of periodic heat transfer on unsteady mixed convection MHD flow past a vertical porous flat plate with constant suction and heat sink when the free stream velocity oscillates in time about a non-zero constant mean. The boundary layer equations governing the problem under consideration are solved by multi-parameter perturbation technique and giving more importance on analytic solution. For this study, mercury (Pr=0.025) and electrolytic solution (Pr=1.0) are the only fluids under consideration.

II. MATHEMATICAL ANALYSIS

We consider an unsteady mixed convection flow of an incompressible and electrically conducting viscous fluid, along an infinite vertical porous flat plate as shown in Fig. 1.

Fig. 1. The physical coordinate system.

The -axis is taken along the infinite vertical plate in the upward direction and axis is perpendicular to it into the fluid flowing with free stream velocity. The velocity vector and the applied transverse magnetic field may be taken as where are the unit vectors along x-axis and y-axis respectively. In view of these, we consider that:

(i) All the fluid properties except density in the buoyancy force term are constant;

(ii) The influence of the density variations in other terms of the momentum and energy equations, and the variation of the expansion coefficient with temperature is negligible;

(iii) The Eckert number E_c and the magnetic Reynolds number are small so that the induced magnetic field can be neglected.

(iv) All the physical variables are independent of x, except possibly the pressure.

With foregoing assumptions and closely following Sahoo et al. (2003) and Soundalgekar (1974), the governing equations of continuity, momentum and energy for mixed convective flow and heat transfer are given by:

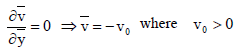	(1)
	(2)
	(3)

Also the free stream velocity oscillates with time is assumed to be of the form:

(4)

where U₀ is the mean stream velocity and e (<<<1) the amplitude of the free stream variation.

By Joulean Heat dissipation, the corresponding boundary conditions of the problem are:

(5)

In the free stream, from (2), we get

(6)

Eliminating between (2) and (5), we get

(7)

Introducing the following dimensionless quantities:

All physical variables are defined in nomenclature.

With the help of boundary conditions (5) and the dimensionless quantities, the equations (3) and (7) reduce to

	(8)
	(9)

and the non-dimensional free stream is

U(t) = 1 + e e^iOt. (10)

The non-dimensional boundary conditions are:

(11)

In this study, the term e e^iOt facilitates the decomposition of the governing coupled equations into simple component equations corresponding to various powers of e, that is, to the steady and unsteady parts. Further, from physical point of view also, this is quite reasonable. As in the case of free stream velocity, we have superimposed a small oscillating part over a steady mean wall temperature. It is a familiar procedure in solving oscillating problems by Lighthill's (1954) technique.

III. METHOD OF SOLUTION

When the amplitude of oscillations (e<<<1) is very small, we can assume the solutions of flow velocity u and temperature field T in the neighborhood of the plate as:

(12)

Where u₀ and T₀ are respectively the mean velocity and mean temperature.

On using (12) into Eqs. (8) and (9), equating harmonic and non-harmonic terms and neglecting e, the following set of equations are obtained:

	(13)
	(14)
	(15)
	(16)

where primes denotes differentiation with respect to y.

The modified boundary conditions are:

(17)

The Eqs. (13) to (16) are still coupled for the variables u₀, u₁, T₀and T₁. To solve them, it is to be noted that E_c<<1 for all incompressible fluid and assumed that:

(18)

where F stands for u₀, u₁, T₀ or T₁ .

On using (18) into Eqs. (13) to (16) and equating the like powers of E_c, the following equations are obtained:

	(19)
	(20)
	(21)
	(22)
	(23)
	(24)
	(25)
	(26)

Subject to the boundary conditions:

	(27)
	(28)

In view of the boundary conditions (27) and (28), the solutions of the differential equations (19) to (26) are:

	(29)
	(30)
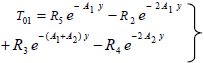	(31)
	(32)
	(33)
	(34)
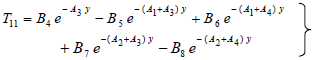	(35)
	(36)

where

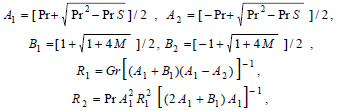
and the other constants like A₃ to A₄ , B₃ to B₁₄ , R₃ to R₁₀ are not presented here for the sake of brevity.

Separating real and imaginary parts of the velocity and temperature expressions (12) and taking only the real parts, the velocity and temperature fields in terms of the fluctuating parts are given by:

u = u₀ + e (M _r cosOt - M _i sin Ot ) (37)

T = T₀ + e (T_r cosOt -T_i sinOt). (38)

The transient velocity and temperature for Ot = π/2 are in the form

u = u₀ - e M _i and T = T₀ - e T_i (39)

The periodic temperature and free stream velocity play an important role in heat transfer characteristics.

IV. SKIN-FRICTION AND RATE OF HEAT TRANSFER

Due to a fluid motion, the dimensionless shearing stress on the surface of a body is known as skin-friction and is defined by the Newton's law of viscosity

(40)

With the help of (37) and (41), the shearing stress component at the plate can be calculated in non-dimensional form as:

(41)

Splitting the Eq. (41) into real and imaginary parts and taking real parts only:

(42)

where

In the dynamics of viscous fluid one is not much interested to know all the details of the velocity and temperature fields but would certainly like to know quantity of heat exchange between the body and the fluid. Since at the boundary the heat exchange between the fluid and the body is only due to conduction, according to Fourier's Law, we have

(43)

With the help of (39) and (43), the coefficient of heat transfer can be calculated in non-dimensional form:

(44)

Splitting Eq. (44) into real and imaginary parts and taking real parts only:

(45)

where

Expressions for B_r, B_i, H_r and H_i are not presented here for the sake of brevity.

V. RESULTS AND DISCUSSION

It is very difficult to study the influence of all parameters involved in the problem on the flow and the thermal field. Therefore, this study focused on the effects of the magnetic field, Sink-strength, Grashof number and Prandtl number on the velocity and temperature field as well as on the mean and fluctuating parts of the skin-friction and the rate of heat transfer. For reference purpose, the values of Prandtl number (Pr) is chosen for mercury (Pr=0.025) and electrolytic solution (Pr=1.0), while the Grashof number Gr>0 corresponds to cooling of the plate. The effects of M, Gr, S and Pr on the mean velocity (u₀) and the transient velocities (u) are shown in the respective Figs. 3 and 4 with other parameters are fixed. It is observed from Fig. 3 that an increase in Gr and S leads to an increase in the mean velocity; but increase in Pr and M reduces the mean velocity. Also the behaviour of transient velocity (Fig. 4) under the influence of M, Gr, S and Pr is same as in case of mean velocity. It is evident from Figs. 3 and 4 that the presence of the transverse magnetic field (M) produces a resistive force on both mean velocity and transient velocity. This force is called the Lorentz force acting on the flow velocity, which leads to slow down the motion of electrically conducting fluid. The increase of mean velocity and transient velocity at the plate with Gr are the greatest. Also it is found that both u₀ and u increase first near the plate and then the trend gets reversed as y increases.

Fig. 2. Comparison of mean velocity distributions of the present work and Sahoo et al. (2003) at M=1, Gr=5, S = -0.2, Ec = 0.005.

Fig.3. Effects of M, Gr, S and Pr on Mean Velocity u₀ for E_c = 0.005

Fig. 4. Effects of M, Gr, S and Pr on Transient Velocity u when E_c=0.005, e=0.5, Ot=π/2, O=5

The effects of M, Gr, S and E_C on the mean temperature (T₀) have been exhibited by the curves shown in Fig. 5. It is noticed that increase in magnetic field and Grashof number raises the mean temperature, whereas increase in Eckert number (E_C) and Sink strength reduces the mean temperature.

Fig. 5. Effects of M, Gr, S and E_c on Mean Temperature T₀ for Pr = 1.0:

In the present study, to verify the accuracy of the present work, particular results are compared with those available in the literature. The unsteady results without free stream velocity oscillating with time are compared with Sahoo et al. (2003). Figure 2 displays this comparison, it can be seen that the agreement between the results is excellent. This has established confidence in the analytical results reported in this article. Therefore, it is seen that the analytical solutions of the present problem with U=0 is same as Sahoo et al. (2003) solutions.

Figure 6 displays the effects of M, Gr, S and O on the transient temperature (T). The transient temperature is rising with the increase in Grashof number (Gr) and frequency parameter (O). It is also noticed that, the increase in magnetic field and Sink strength reduces T. It is found that the mean temperature and transient temperature rises with the Grashof number for both the cases of Pr = 1.0 and Pr=0.025.

Fig. 6. Effects of M, Gr, S and O on Transient Temperature T when E_c = 0.005, Pr = 0.025, e = 0.5, Ot = π/2:

The effects of frequency parameter and phase angle on transient velocity (u) are shown in Figs. 7 and 8. In both the figures, it is observed that increase in frequency parameter increases the transient velocity in mercury or electrolytic solution, while increase in phase angle reduces the transient velocity for both the cases of mercury and electrolytic solution.

Fig. 7. Effects of O and Ot on the transient velocity u when Pr=1.0, M=1, Gr=2, S=-0.2, Ec=0.005, e=0.5

Fig. 7. Effects of O and Ot on the transient velocity u when Pr=0.025, M=1, Gr=2, S=-0.2, Ec=0.005, e=0.5

The numerical values of mean Skin-friction () and corresponding amplitude |B| and phase (tana) are presented in the Table-1 for different values of M, Gr, S, and Pr A close study of Table-1 indicates that an increase in magnetic field increases , while it decreases with increase in Skin-strength and Grashof number for Pr = 1.0, but a similar result is obtained for Pr = 0.025 under the influence of M and S. Furthermore, it is interesting to note that for Pr=0.025 the mean skin-friction increases with increasing Grashof number which is true from the physical point of view because of buoyancy increases in the upward direction. It is observed that, the increase in M, Gr and S increases |B| for both Pr=1.0 and Pr = 0.025. Also for Pr = 1.0 it is seen that, an increase in M and Gr decreases tana; but in presence of mercury, this effects of M and Gr increases tana. Also there is a similar behaviour of S is observed on tana in case of Pr = 1.0 or Pr = 0.025.

Table 1: Values of mean skin-friction (), amplitude (|B|) and phase (tana ) for E_c = 0.005, e = 0.5 , Ot = π/2, O = 3.0:

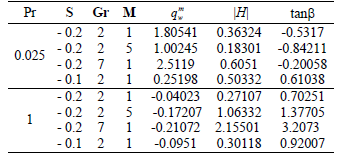
The numerical values of mean heat transfer (), amplitude |H| and phase tanß are presented in the Table-2 for different values of M, Gr, S and Pr . The mean rate of heat transfer decreases with increase in magnetic field strength or sink-strength for both situations Pr = 1.0 and Pr = 0.025, while a reverse phenomenon is observed for Grashof number. The amplitude and phase both increases with magnetic field strength in case of electrolytic solution and this behaviour is opposite to the case of mercury. The effects of increase in Grashof number and Sink-strength on tanß are same as in case of amplitude |H| for Mercury as well as electrolytic solution. It is noticed that, increases with Gr for Pr = 0.025, but this effect is reversed to Pr=1.0.

Table 2: Values of mean heat transfer (), amplitude (|H|) and phase (tanß), for Ec = 0.005 , e = 0.5 , Ot = π/2 , O = 3.0 :

VI. CONCLUSIONS

1. It is observed that the mean and transient velocity decreases with the increase in Pr. Physically this is true because the increase in the Prandtl number is due to increase in the viscosity of the fluid, which makes the fluid thick and hence a decrease in the velocity of the fluid.

2. The magnetic parameter M has a retarding effect on both mean velocity and transient velocity. This is a result of magnetic pull of the Lorentz force acting on the velocity field.

3. Due to heat dissipation, it is observed that the mean temperature and transient temperature rises with the Grashof number for both the electrolytic solution and Mercury.

4. The increase of mean velocity and transient velocity at the plate with Gr are the greatest and this behaviour is opposite to the electrolytic solution.

5. It is observed that, the effects of increase in magnetic field and Grashof number on are reversed to in both the cases Pr = 1.0 and Pr=0.025.

6. The behaviour of |H| and |B| are similar for Pr=1.0.

7. Also it is noticed that, tana and tanß increases respectively in the fluid of mercury and electrolytic solution.

8. It is noticed that velocity increases rapidly in the neighbourhood of the plate and then decreases far away from the plate.

NOMENCLATURE

	Velocity components along and direction respectively,
v0	Mean suction velocity,
u	Dimensionless velocity component,
g	Acceleration due to gravity,
B0	Magnetic field,
	Time,
	Free stream velocity,
	Temperature at the plate,
	Free stream temperature,
	Fluid temperature,
T	Dimensionless temperature,
Pr	Prandtl number,
Gr	Grashof number,
S	Sink strength,
M	Hartmann number,
	Mean skin-friction,
	Mean heat transfer and
Ec	Eckert number.

Greek symbols

ß	Coefficient of volume expansion,
e	Amplitude parameter,
?	Thermal conductivity,
?	Density,
μ	Coefficient of viscosity,
	Kinematic viscosity,
O	Frequency parameter,

Subscripts

W	Evaluated at wall conditions
∞	Evaluated at free stream conditions

ACKNOWLEDGEMENT
The present work is dedicated to Dr. V. M. Soundalgekar of IIT-Bombay for his novel work on unsteady heat transfer flow in the decade of 1980's. The author is thankful to the referees for their comments towards the improvement of the paper.

REFERENCES

1. Ahmed, S. and N. Ahmed, "Two-dimensional MHD oscillatory flow along a uniformly Moving infinite vertical porous plate bounded by porous medium", IJPAM, 35, 1309-1319 (2004).        [ Links ]

2. Ahmed, S., "Effects of unsteady free convective MHD flow through a porous medium bounded by an infinite vertical porous plate", Bull. Cal. Math. Soc., 99, 511-522 (2007).        [ Links ]

3. Lighthill, M.J., "The response of laminar skin Friction and heat transfer to fluctuations in the stream velocity", Proc. Roy. Soc. A224, 1-16 (1954).        [ Links ]

4. Messiha, S.A.S., "Laminar boundary layers in oscillatory flow along an infinite flat plate with variable suction", Proc. Camb. Phil. Soc., 62, 329-37 (1966).        [ Links ]

5. Nanda, R.S. and V.P. Sharma, "Free convection boundary layers along a semi-infinite vertical plate", J. Fluid Mech. 15, 419-428 (1963).        [ Links ]

6. Ogulu, A. and J. Prakash, "Heat transfer to unsteady magneto-hydrodynamic flow past an infinite moving vertical plate with variable suction", Royal Swedish Academy of Scies, 74, 232-239 (2006).        [ Links ]

7. Pop, I. and V.M. Soundalgekar, "Unsteady free convection flows past an infinite plate with constant suction and heat sources", Int. J. Heat Mass Transfer, 17, 85-92 (1974).        [ Links ]

8. Raptis, A.A. and C.P. Perdikis, "Oscillatory flow through a porous medium by the presence of free convective flow", Int .J. Engng. Sci., 23, 51-55 (1985).        [ Links ]

9. Singh, K.R. and T.G. Cowling, "Effect of magnetic field on free convective flow of electrically conducting fluids past a semi-infinite flat plate." Quart. J. Mech. Appl. Math., 16, 1-15 (1963).        [ Links ]

10. Soudalgekar, V.M., "Free convection effects on steady MHD flow past a vertical porous plate", J. Fluid Mech., 66, 541-55 (1974).        [ Links ]

11. Sacheti, N.C., P. Chandran and A.K. Singh, "An exact solution for the unsteady MHD flow", Int. Comm. Heat Mass Transfer, 21, 131-142 (1994).        [ Links ]

12. Sattar, A.Md. and Md.M. Alam, "MHD free convective heat and mass transfer flow with Hall current and constant heat flux through a porous medium", IJPAM, 26, 157-167 (1995).        [ Links ]

13. Sahoo, P.K., N. Datta and S. Biswal, "MHD unsteady free convection flow past an infinite vertical plate with constant suction and heat sink", IJPAM, 34, 145-55 (2003).        [ Links ]

14. Vighnesam, N.V. and V.M. Soundalgekar, "Combined free and force convection flow of water at 4^oC from a vertical plate with variable temperature", Ind. J. of Engineering and Material Sciences, 5, 124-126 (1998).        [ Links ]

Received: June 28, 2008.
Accepted: June 29, 2009.
Recommended by Subject Editor Walter Ambrosini.