Enhancement to the LuGre model for global description of friction phenomena

R. Kelly¹

¹ División de Física Aplicada, CICESE, Apdo. Postal 2516, Adm. 1, Ensenada, B.C., 22800, Mexico
rkelly@cicese.mx

Abstract — The LuGre model of friction -a bristle based model- predicts important friction phenomena useful for control of mechanical systems. This model accurately describes the behavior of control systems for small initial conditions. This paper proposes a simple but fundamental modification of the model in order to improve the reliability in a global sense. This improvement increases the comprehension of experimental evidences in control of mechanisms.

Keywords — Control. Friction. Mechanisms. LuGre Model. PD Control.

I. INTRODUCTION

The study of friction in the automatic control community has grown during the last decade (Armstrong-Helouvry, 1991; Armstrong-Helouvry, et al. 1994; Alvarez-Ramirez, et al. 1995). The reason is that friction is responsible for many undesirable phenomena observed in implementation of control systems for high precision mechanical systems such as robotic manipulators. Friction produces undesirable behaviors in control systems such as positioning and tracking errors, and limit cycles (Shapiro, 2000). Compensating for friction to attenuate these effects has been one of the main research issues in mechanism control over the years (see Lischinsky et al. (1999) and reference therein).

Viscous friction and Coulomb friction are by far the most popular ingredients in friction models utilized for control of mechanical systems. More elaborate models incorporate in addition to viscous and Coulomb friction also the Stribeck effect, to better capture the behavior of motion at low velocity (Armstrong-Helouvry, 1991). Although these friction models are simple because they establish that friction force or torque depends on the instantaneous relative velocity between the bodies in contact, there exist also dynamic friction models where the actual friction force or torque is a function of the instantaneous velocity but also of the previous behavior (Ludema, 1996). To the latter class belongs the LuGre model proposed in Canudas et al. (1995) which describes the effects of viscous and Coulomb friction but also more complex friction behavior such as stick-slip motion (Polycarpou and Soom, 1995), presliding displacement (Hsieh and Pan, 2000), and Stribeck effect.

The LuGre model for friction proposed in Canudas et al. (1995) seems to accommodate pretty well to expectation of improving precision in mechanisms by control based in such a friction model. Nevertheless, in this paper we present simulation evidences of a simple mechanical system incorporating this friction model which predicts unrealistic behavior when departing from certain initial conditions. To overcome this drawback, this paper proposes a simple but fundamental modification to the model allowing to match its predictions to real expectations irrespective of the initial conditions.

II. LUGRE MODEL OF FRICTION

The LuGre model widely described in Canudas et al. (1995) consists of a differential equation where the relative velocity between the bodies in contact is the system input, and the friction force or torque f opposing to the bodies motion is the system output. The LuGre model can be written in a suitable form as

	(1)
	(2)

where

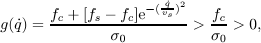(3)

z is an immeasurable state variable called "average deflection of the bristles" (Canudas et al., 1995) because the friction interface between the bodies is thought as a contact between bristles (Rice and Moslehy, 1997), f_c is the Coulomb friction coefficient, f_s is the static friction coefficient satisfying f_s > f_c, f_v is the viscous friction coefficient, and vs is the Stribeck velocity coefficient. Finally, σ₀ and σ₁ are the stiffness and damping coefficients respectively. In sum, the LuGre model is composed by the differential equation (1) and the output equation (2).

III. LIMITATION OF THE LUGRE MODEL

In this section we show that the LuGre model fails to predict a specific behavior when departing form certain initial conditions. To illustrate this behavior, let us consider as mechanism an innocuous rigid body having inertia J where friction f opposes to the rotational motion:

(4)

where τ is the external applied torque.

Consider that friction f is modeled by the LuGre friction model (l)-(2), and the applied torque τ - τ₀ > 0 is a constant torque. Hence, the system dynamics is governed by the following nonlinear differential equation:

(5)

whose equilibria set is given by

(6)

The equilibrium set E in (6) always exists no matter how large the input torque τ₀ might be. This simple analysis of the equilibrium set E means that if the initial condition for the system originates within any point in the set E, i.e. [q(0) (0) z(0)]^T ∈ E, then the body will remain at rest in its initial position, i.e. q(t) - q(0) for all t ≥ 0, irrespective of the value of the applied constant torque τ₀- Although the claim is true for small values of the applied torque τ₀, the conclusion is obviously wrong for enough large applied torques ro because motion will be always present, thus the position q(t) cannot remain constant. This incoherence of the LuGre model is at the origin of the enhancement proposed in this paper. Before that, let's see the system behavior more in details.

Hereafter we pay attention to the particular case when the applied constant torque τ₀ is larger than the static friction coefficient f_s, i.e. to > f_s > 0. Extensive simulations of system (5) have been carried out to capture the qualitative behavior of the system. The results of these simulations are summarized in Fig. 1 where some trajectories in the state space are depicted. Two important structures in the state space are shown in Fig. 1: an invariant set denoted by IS, and the equilibrium set E. Let us analyze each structure in the following paragraphs.

Figure 1 depicts a structure in the state space corresponding to the invariant set IS defined by

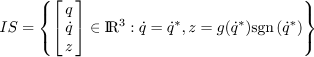(7)

Figure 1: Trajectories in the state space for τ₀ > f_s: Original LuGre model

where sgn() is the sign "function" defined as

and is the unique solution of

From definition (7) and the system model (5), it can be proven that the set IS is an invariant set because for any initial condition in IS, the corresponding solution remains within IS, i.e.

This invariant set IS is expected to be there because experimental evidences demonstrate that if a constant torque To larger than the static friction coefficient f_s is applied to the body, then it will move and converge asymptotically to a constant velocity different from zero.

As previously pointed out, the equilibrium set E appears in the state space depicted in Fig. 1. Some trajectories converge to the set E even though the simulations have been obtained for a large applied torque in the sense that τ₀ > f_s. All the points in the set correspond to zero velocity, that is, all trajectories converging to the set E finally yield (t) → 0 as t → ∞. This prediction of the LuGre model contradicts the expected behavior of the system.

Although the validity of the LuGre is correct in a local region around of the invariant set IS, e.g. by restricting the initial condition z(0) to be small in the sense |z(0)|< f_s/τ₀, we believe that it is important to have a full global model to describe the behavior in the complete state space. This is the motivation to introduce the following improved LuGre model.

IV. IMPROVED LUGRE MODEL

The mathematical explanation to the incorrect prediction generated by the LuGre model when the applied torque τ₀ is larger than the static friction coefficient f_s is the existence of the equilibria set E. Thus, the LuGre model must be adjusted in such a way that the equilibrium set E do not exist any more when τ₀ > f_s. Towards this end, notice that the equilibria E given in (6) contain = 0 and z solution of

τ₀ - σ₀z = 0 (8)

which results in z = τ₀/σ₀ irrespective of the value of τ₀. Since = 0 appears in any possible equilibrium, hence one way to guarantee that no equilibria exist is by modifying (8) in such a way that the solution z = τ₀/σ₀ exists if and only if to < f_s. Let us propose the following simple expression

τ₀ - σ₀ sat(z; f_s/σ₀) = 0 (9)

where sat(x; k) stands for the hard saturation function defined as

for any x ∈ and k > 0.

Because |sat(z;f_s/σ₀)| ≤ f_s/σ₀ for all z ∈ , then no solution z exists for (9) when τ₀ > f_s as desired. These arguments motivate to modify only the output equation (2) of the LuGre model in the following way

(10)

In sum, the modified LuGre dynamic friction model is described by the state equation (1) and the new output equation (10) with g() still given in (3). As a consequence of the modification, the friction torque output / of the modified LuGre model at rest = 0 is now explicitly bounded as |f| ≤ f_s which holds for all q, z ∈ . This result obviously matches the well known fact that at rest the friction torque is smaller or equal than the static friction parameter.

Let us analyze again the behavior of the simple mechanical system studied previously but using now the modified LuGre friction model (1) and (10). Consider the motor model (4) where the modified LuGre model (1) and (10) is utilized. The motion of the body is governed by

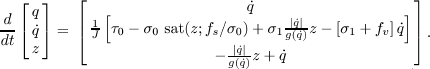(11)

The equilibria of this nonlinear differential equation are those elements of the state space for which = 0 and z solution of (9). The latter equation has solution if and only if the applied torque τ₀ is smaller or equal than the static friction coefficient f_s. For this case, the equilibria are contained in the set E defined in (6).

Since (9) has no solution z when the applied torque τ₀ is larger than the static friction coefficient f_s, then we get the conclusion that the system (11) has no equilibria whereas τ₀ > f_s. This means that the body cannot remain at rest, which agrees with experimental observations and simulation results.

Figure 2: Trajectories in the state space for τ₀ > f_s Improved LuGre model

A set of numerical simulations for τ₀ > f_s were conducted of the system (11) derived form the novel improved LuGre model. These simulations include the same initial conditions and parameters that those used to produce Fig. 1. The simulation results depicted on Fig. 2 show that the equilibrium set E no longer exist but only the invariant set IS as desired. The invariant set IS corresponds exactly to (7) generated from the original LuGre model; this is thanks to the fact that sat(g()sgn());f_s/σ₀) = g()sgn() because from (3) we have f_s/σ₀ > g(q).

In sum, the proposed improved LuGre model described by (1), (3), and (10), has the following desirable global structural features when τ₀ > f_s:

• The equilibrium set E is not present any more.
• The same invariant set IS generated by the original LuGre model is preserved.

It is worth remarking that an alternative output equation to (10) is given by

which preserves all the global structural behavior of (10) but also the important physical property of passivity (Barabanov and Ortega, 2000).

V. APPLICATION TO CONTROL A DC MOTOR

The usefulness of the improved LuGre friction model is illustrated in the control of a direct current (DC) motor. A classical linear description of a linear DC motor

Figure 3: Equilibria of the system with PD control

considering the torque τ as the input is given by (4) (Moreno and Kelly, 2002). Consider that the friction f is captured by the new improved LuGre friction model (1), (3) and (10).

Let us consider the Proportional-Derivative (PD) control given by

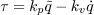(12)

where is the positioning error defined as = q_d - q with q_d being the desired rotor angular position which is assumed to be constant, k_p and k_v are positive gains. It is well known that in the absence of friction, the closed-loop system is globally asymptotically stable (Spong and Vidyasagar, 1989).

The presence of friction may deteriorate the control performance. In order to analyze this situation, let us obtain the closed-loop system by substituting the control action (12) into the motor system composed by (4), (1) and (10). This leads to

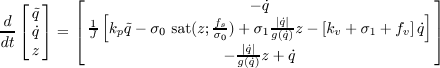
which has the equilibria set depicted in Fig. 3 and characterized by

According to this analysis, the corresponding positioning errors for equilibria in the set W are bounded as

(13)

Therefore, the upper bound on the positioning error || can be decreased by increasing the controller gain k_p (see Fig. 3). This intuitive behavior which states that the position error due to static friction can be reduced by increasing the gain k_p is predicted by the original LuGre model but under the constraint of |z(0)| ≤ f_s/σ₀ which has been now relaxed thanks to the proposed modification.

Figure 4: PD control: Experimental result

Digital implementation of the controller (12) was conducted on a experimental DC motor. The static friction parameters for the motor were obtained according to the procedure described in Kelly et al. (2000); in particular, the static friction parameter is f_s = 0.85 [Nm]. During experiments, the proportional gain k_p was set k_p = l.4 [Nm/rad], hence the previous analysis predicts (13), that is, the position error || is upper bounded by f_s/k_p = 34.7 [deg]. This prediction was well confirmed during experiments as shown in Fig. 4 where the steady state position error is approximately 34 [deg].

VI. CONCLUSIONS

A modification to improve the LuGre model of dynamic friction has been proposed. This allows a global description of the friction phenomena, therefore to predict a behavior closer to experimental observations. The modification remains quite simple, and keeps the fine features of the original LuGre model. Finally, the idea behind the modification applies straightforward to other dynamic models of friction such as the Dahl model (Dahl, 1976).

REFERENCES
1. Alvarez-Ramirez, J., R. Garrido, and R. Femat, "Control of systems with friction", Physical Review E, 51, 6235-6238 (1995).         [ Links ]
2. Armstrong-Helouvry, B., Control of Machines with Friction, Kluwer Academic Publishers, Boston, MA. (1991).         [ Links ]
3. Armstrong-Helouvry, B., P. Dupont, and C. Canudas de Wit, "A survey of models, analysis tools and compensation methods for control of machines with friction", Automatica, 30, 1083-1138 (1994).         [ Links ]
4. Barabanov, N., and R. Ortega, "Necessary and sufficient conditions for passivity of the LuGre friction model", IEEE Transactions on Automatic Control, 45, 830-832 (2000).         [ Links ]
5. Canudas de Wit, C., H. Olsson, K.J. Astrom and P. Lischinsky, "A new model for control of systems with friction", IEEE Transactions on Automatic Control, 40, 419-425 (1995).         [ Links ]
6. Dahl, P. R., "Solid friction damping of mechanical vibrations", AIAA Journal, 14, 1675-1682 (1976).         [ Links ]
7. Hsieh, C., and Y. C. Pan, "Dynamic behavior and modelling of the pre-sliding static friction", Wear, 242, 1-17 (2000).         [ Links ]
8. Kelly, R., J. Llamas, and R. Campa, "A measurement procedure for viscous and Coulomb friction", IEEE Transactions on Instrumentation and Measurement, 49, 857-861 (2000).         [ Links ]
9. Lischinsky, P., C. Canudas de Wit and G. Morel, "Friction compensation for an industrial hydraulic robot", IEEE Control Systems, 19, 25-30 (1999).         [ Links ]
10. Ludema, K.C., "Mechanism-based modeling of friction and wear", Wear, 200, 1-7 (1996).         [ Links ]
11. Moreno, J., and R. Kelly, "On motor velocity control by using only position measurements: two cases studies", International Journal of Electrical Engineering Education, 39, 118-127 (2002).         [ Links ]
12. Polycarpou, A. A., and A. Soom, "Application of a two-dimensional model of continuous sliding friction to stick-slip", Wear, 181, 32-41 (1995).         [ Links ]
13. Rice, S. L., and F. A. Moslehy, "Modeling friction and wear phenomena", Wear, 206, 136-146 (1997).         [ Links ]
14. Shapiro, A. M., "Self-induced oscillations of friction process parameters", Wear, 237, 223-230 (2000).         [ Links ]
15. Spong, M. W., and M. Vidyasagar, Robot Dynamics and Control, John Wiley and Sons, Singapore (1989).         [ Links ]